Abstract
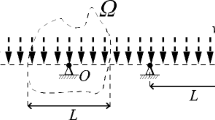
Since the time of Huygens in the 17th century it has been believed that, if the weight of the structural members themselves are negligible in comparison to the applied load, the optimum structure to carry a uniformly distributed load between pinned supports will take the form of a parabolic arch rib (or, equivalently, a suspended cable). In this study, numerical layout optimization techniques are used to demonstrate that when a standard material with equal tension and compressive strength is involved, a simple parabolic arch rib is not the true optimum structure. Instead, a considerably more complex structural form, comprising a central parabolic section and networks of truss bars in the haunch regions, is found to possess a lower structural volume.





Similar content being viewed by others
Notes
For example, Rozvany and Prager (1979) were careful to preface their work on optimal arch-grids as follows: ‘It is either stipulated or assumed without explicit proof that the optimal solution for a single load condition consists of arches which are subject only to compression and no bending moments so that all cross-sections are stressed uniformly to a given stress σ 0’.
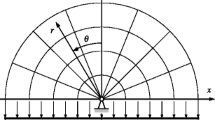
Note that if an over-restrictive domain is used the computed volume will tend to grossly overestimate the true optimal volume.
N.B. These ‘virtual’ bars act only to transmit the load to the most optimal point of application; they cannot form integral parts of the ‘real’ structure.
Only the results from analyses with 40 or more nodal divisions have been used in the computation of the best-fit constants for (9). This is because a certain minimum nodal resolution is required before the detailed structure of the optimal form presented here begins to emerge.
References
Chan HSY (1975) Symmetric plane frameworks of least weight. In: Sawczuk A, Mroz Z (eds) Optimization in structural design, Proc. IUTAM symp., Warsaw, pp 313–326
Chiandussi G, Codegone M, Ferrero S (2009) Topology optimization with optimality criteria and transmissible loads. Comput Math Appl 57(5):772–788
Darwich W, Gilbert M, Tyas A (2007) Optimum structure to carry a uniform load between two pinned supports. In: 7th world congress of structural and multidisciplinary optimization, Seoul, Korea
Dorn WS, Gomory RE, Greenberg HJ (1964) Automatic design of optimal structures. J de Mechanique 3:25–52
Fuchs MB, Moses E (2000) Optimal structural topologies with transmissible loads. Struct Multidisc Optim 19:263–273
Gilbert M, Tyas A (2003) Layout optimization of large-scale pin-jointed frames. Eng Comput 20(7–8):1044–1064. doi:10.1108/02644400310503017
Gilbert M, Darwich W, Tyas A, Shepherd P (2005) Application of large-scale layout optimization techniques in structural engineering practice. In: 6th world congresses of structural and multidisciplinary optimization, Rio de Janeiro, Brazil
Hemp WS (1974) Michell frameworks for uniform load between fixed supports. Eng Optim 1:61–69
Knuth D (1997) The art of computer programming, vol 2: seminumerical algorithms, 3rd edn. Addison-Wesley, Reading
Lockwood EH (1961) A book of curves. Cambridge University Press, Cambridge
McConnel RE (1974) Least-weight frameworks for loads across span. J Eng Mech ASCE 100:885–901
Owen JBB (1965) The analysis and design of light structures. Edward Arnold, London
Rozvany GIN (2009) A critical review of established methods of structural topology optimization. Struct Multidisc Optim 37(3):217–237
Rozvany GIN, Prager W (1979) A new class of structural optimization problems—optimal archgrids. Comput Methods Appl Mech Eng 19(1):127–150
Rozvany GIN, Wang CM (1983) On plane Prager-structures. Int J Mech Sci 25(7):519–527
Rozvany GIN, Wang CM, Dow M (1982) Prager-structures: archgrids and cable networks of optimal layout. Comput Methods Appl Mech Eng 31:91–113
Sylla ED (2003) Die werke von Jakob Bernoulli. Hist Math 30(3):378–380
Yang XY, Xie YM, Steven GP (2005) Evolutionary methods for topology optimisation of continuous structures with design dependent loads. Comput Struct 83(12–13):956–963
Author information
Authors and Affiliations
Corresponding author
Additional information
This research has been supported by Buro Happold Ltd (sponsorship of Wael Darwich’s doctoral studies) and EPSRC (Advanced Research Fellowship grant GR/S53329/01 held by Matthew Gilbert).
Appendix: Extrapolation of numerically computed data to estimate volume for infinite nodal density
Appendix: Extrapolation of numerically computed data to estimate volume for infinite nodal density
In a recent review article Rozvany (2009) observes that ‘Most of the authors in numerical topology optimization simply compare their solutions visually with the exact optimal truss topology and are satisfied with a vague resemblance’. He goes on to suggest that a rigorous verification approach should comprise the following steps:
-
1
For a given set of constraints, derive numerically the optimal topology for various numbers of elements.
-
2
Calculate the structural volume (weight) for each solution.
-
3
Extrapolate the volume (weight) value for infinite number of elements.
-
4
Compare this extrapolated value with that calculated analytically for the exact benchmarks.
These steps have been applied to the numerical data presented in Fig. 6.Footnote 4 These data appear to follow a relation of the form:
where \(V_{n_x}\) is the numerically computed optimal volume for n x equally spaced nodal divisions across the span, V ∞ is the volume when n x → ∞, and k and α are positive constants.
Using (9), a non-linear least-squares approach was used to find best-fit values for V ∞ , k and α. The resulting trend lines and values for V ∞ are shown in Fig. 6.
Rights and permissions
About this article
Cite this article
Darwich, W., Gilbert, M. & Tyas, A. Optimum structure to carry a uniform load between pinned supports. Struct Multidisc Optim 42, 33–42 (2010). https://doi.org/10.1007/s00158-009-0467-0
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00158-009-0467-0