Skip to main content
SpringerLink
Log in

Topology optimization of hyperelastic bodies including non-zero prescribed displacements

  • Research Paper
  • Published:
Structural and Multidisciplinary Optimization Aims and scope Submit manuscript

Abstract

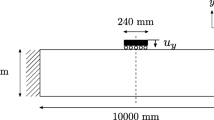
Stiffness topology optimization is usually based on a state problem of linear elasticity, and there seems to be little discussion on what is the limit for such a small rotation-displacement assumption. We show that even for gross rotations that are in all practical aspects small (<3 deg), topology optimization based on a large deformation theory might generate different design concepts compared to what is obtained when small displacement linear elasticity is used. Furthermore, in large rotations, the choice of stiffness objective (potential energy or compliance), can be crucial for the optimal design concept. The paper considers topology optimization of hyperelastic bodies subjected simultaneously to external forces and prescribed non-zero displacements. In that respect it generalizes a recent contribution of ours to large deformations, but we note that the objectives of potential energy and compliance are no longer equivalent in the non-linear case. We use seven different hyperelastic strain energy functions and find that the numerical performance of the Kirchhoff–St.Venant model is in general significantly worse than the performance of the other six models, which are all modifications of this classical law that are equivalent in the limit of infinitesimal strains, but do not contain the well-known collapse in compression. Numerical results are presented for two different problem settings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Notes

  1. For the nested formulation, the last term disappears and the Lagrangian becomes the potential energy.

References

  • Bendsøe MP (1989) Optimal shape design as a material distribution problem. Struct Optim 1:193–202

    Article  Google Scholar 

  • Bendsøe M, Sigmund O (2002) Topology optimization, theory, methods and applications. Springer, New York

    Google Scholar 

  • Bonet J, Wood RD (2008) Nonlinear continuum mechanics for finite element analysis. Cambridge University Press, Cambridge

    Book  MATH  Google Scholar 

  • Bruns TE, Sigmund O (2004) Toward the topology design of mechanisms that exhibit snap-through behavior. Comput Methods Appl Mech Eng 193:3973–4000

    Article  MathSciNet  MATH  Google Scholar 

  • Bruns TE, Sigmund O, Tortorelli DA (2002) Numerical methods for the topology optimization of structures that exhibit snap-through. Int J Numer Methods Eng 55:1215–1237

    Article  MATH  Google Scholar 

  • Bruns TE, Tortorelli D (2001) Topology optimization of non-linear elastic structures and compliant mechanisms. Comput Methods Appl Mech Eng 190:3443–3459

    Article  MATH  Google Scholar 

  • Buhl T, Pedersen CB, Sigmund O (2000) Stiffness design of geometrically nonlinear structures using topology optimization. Struct Multidisc Optim 19:93–104

    Article  Google Scholar 

  • Cho S, Jung H-S (2003) Design sensitivity analysis and topology optimization of displacement-loaded non-linear structures. Comput Methods Appl Mech Eng 192:2539–2553

    Article  MATH  Google Scholar 

  • Christensen P, Klarbring A (2008) An introduction to structural optimization. Springer, New York

    Google Scholar 

  • Curnier A (1994) Computational methods in solid mechanics. Kluwer Academic, Dordrecht

    Book  MATH  Google Scholar 

  • Fleury C (1979) Structural weight optimization by dual methods of convex programming. Int J Numer Methods Eng 14:1761–1783

    Article  MATH  Google Scholar 

  • Fleury C, Braibant V (1986) Structural optimization: a new dual method using mixed variables. Int J Numer Methods Eng 23:409–428

    Article  MathSciNet  MATH  Google Scholar 

  • Gea HC, Luo J (2001) Topology optimization of structures with geometrical nonlinearities. Comput Struct 79:1977–1985

    Article  Google Scholar 

  • Groenwold AA, Etman LFP (2008) On the equivalence of optimality criterion and sequential approximate optimization methods in the classical topology layout problem. Int J Numer Methods Eng 73:297–316

    Article  MathSciNet  MATH  Google Scholar 

  • Haftka RT, Gürdal Z (1992) Elements of structural optimization. Kluwer Academic, Dordrecht

    Book  MATH  Google Scholar 

  • Holzapfel GA (2000) Nonlinear solid mechanics, a continuum approach for engineering. Wiley, New York

    MATH  Google Scholar 

  • Huang X, Xie YM (2008) Topology optimization of nonlinear structures under displacement loading. Eng Struct 30:2057–2068

    Article  Google Scholar 

  • Jung D, Gea HC (2004) Topology optimization of nonlinear structures. Finite Elem Anal Des 40:1417–1427

    Article  Google Scholar 

  • Kemmler R, Lipka A, Ramm E (2005) Large deformations and stability in topology optimization. Struct Multidisc Optim 30:459–476

    Article  MathSciNet  MATH  Google Scholar 

  • Klarbring A, Strömberg N (2011) A note on the min-max formulation of stiffness optimization including non-zero prescribed displacements. Struct Multidisc Optim. doi:10.1007/s00158-011-0674-3

    Google Scholar 

  • Lee WS, Youn SK (2004) Topology optimization of rubber isolators considering static and dynamic behaviours. Struct Multidisc Optim 27:284–294

    Article  Google Scholar 

  • Niu F, Xu S, Cheng G (2011) A general formulation of structural topology optimization for maximizing structural stiffness. Struct Multidisc Optim 43:561–572

    Article  Google Scholar 

  • Pajot JM, Maute K (2006) Analytical sensitivity analysis of geometrically nonlinear structures based on the co-rotational finite element method. Finite Elem Anal Des 42:900–913

    Article  MathSciNet  Google Scholar 

  • Pedersen C, Buhl T, Sigmund O (2001) Topology synthesis of large-displacement compliant mechanisms. Int J Numer Methods Eng 50:2683–2705

    Article  MATH  Google Scholar 

  • Pedersen P, Pedersen N (2011) Design objectives with non-zero prescribed support displacements. Struct Multidisc Optim 43:205–214

    Article  Google Scholar 

  • Sigmund O (2001) A 99 line topology optimization code written in Matlab. Struct Multidisc Optim 21:120–127

    Article  Google Scholar 

  • Zhou M, Rozvany GIN (1991) The COC algorithm, part II: topological, geometrical and generalized shape optimization. Comput Methods Appl Mech Eng 89:309–336

    Article  Google Scholar 

Download references

Acknowledgement

This project was financed by Swedish Foundation for Strategic Research through the ProViking programme.

Author information

Authors and Affiliations

  1. Mechanics, Department of Management and Engineering, The Institute of Technology, Linköping University, 581 83, Linköping, Sweden

    Anders Klarbring

  2. Department of Mechanical Engineering, Jönköping University, 551 11, Jönköping, Sweden

    Niclas Strömberg

Authors
  1. Anders Klarbring
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Niclas Strömberg
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Niclas Strömberg.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Klarbring, A., Strömberg, N. Topology optimization of hyperelastic bodies including non-zero prescribed displacements. Struct Multidisc Optim 47, 37–48 (2013). https://doi.org/10.1007/s00158-012-0819-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00158-012-0819-z

Keywords

Search

Navigation