Determination of metamaterial parameters by means of a homogenization approach based on asymptotic analysis

Abstract

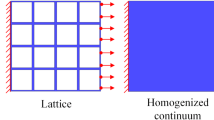
By using modern additive manufacturing techniques, a structure at the millimeter length scale (macroscale) can be produced showing a lattice substructure of micrometer dimensions (microscale). Such a system is called a metamaterial at the macroscale, because its mechanical characteristics deviate from the characteristics at the microscale. Consequently, a metamaterial is modeled by using additional parameters. These we intend to determine. A homogenization approach based on asymptotic analysis establishes a connection between these different characteristics at micro- and macroscales. A linear elastic first-order theory at the microscale is related to a linear elastic second-order theory at the macroscale. Small strains (and, correspondingly, small gradients) are assumed at both scales. A relation for the parameters at the macroscale is derived by using the equivalence of energy at macro- and microscales within a so-called representative volume element (RVE). The determination of the parameters becomes possible by solving a boundary value problem within the framework of the finite element method. The proposed approach guarantees that the additional parameters vanish if the material is purely homogeneous; in other words, it is fully compatible with conventional homogenization schemes based on spatial averaging techniques. Moreover, the proposed approach is reliable, because it ensures that the obtained additional parameters are insensitive to choices of the RVE consisting of a repetition of smaller RVEs depending upon the intrinsic size of the structure.

Appendix: Asymptotic solution for the displacement field

The asymptotic solution for an RVE is derived. Specifically, the solutions of Eqs. (24), (25), and (26) are shown.

We start with Eq. (24). Because $C_{ijkl}^\text {m}$ is a function of $\varvec{y}$, the only possible general solution of Eq. (24) is to restrict $\overset{0}{u}_i({\varvec{X}})$, since it is $\varvec{y}$-periodic and has a bounded gradient. The solution in the order of $\epsilon ^{-2}$ can be given as:

$$\begin{aligned} \overset{0}{u}_i = \overset{0}{u}_i({\varvec{X}}). \end{aligned}$$

(43)

Note that $\overset{0}{u}_i({\varvec{X}})$ depends only on the macroscopic coordinates. It is assumed to be the known macroscopic displacement $\overset{0}{u}_i({\varvec{X}}) =u^\text {M}_i({\varvec{X}})$. By substituting Eq. (43) into Eq. (25), by introducing $\varphi _{abc}=\varphi _{abc}(\varvec{y})$, for the inverse operation, we obtain

$$\begin{aligned}&\frac{\partial C_{ijab}^\text {m}}{\partial y_j} \frac{\partial \overset{0}{u}_a}{\partial X_b} = - \frac{\partial }{\partial y_j} \bigg ( C_{ijkl}^\text {m} \frac{\partial \overset{1}{u}_k}{\partial y_l} \bigg ), \nonumber \\&\frac{\partial C_{ijab}^\text {m} }{\partial y_j} = - \frac{\partial }{\partial y_j} \bigg ( C_{ijkl}^\text {m} \frac{\partial \varphi _{abk} }{\partial y_l} \bigg ), \nonumber \\&\frac{\partial }{\partial y_j} \bigg ( C_{ijkl}^\text {m} \Big ( \frac{\partial \varphi _{abk}}{\partial y_l} + \delta _{ak} \delta _{bl} \Big ) \bigg ) = 0. \end{aligned}$$

(44)

Then, the general solution of Eq. (25) can be given as:

$$\begin{aligned} \overset{1}{u}_i = \varphi _{abi} \overset{0}{u}_{a,b} + \overset{1}{\bar{u}}_i({\varvec{X}}), \end{aligned}$$

(45)

where $\overset{1}{\bar{u}}_i =\overset{1}{\bar{u}}_i({\varvec{X}})$ are integration constants in $\varvec{y}$.

Substitution of Eqs. (43) and (45) (with $\overset{1}{\bar{u}}_i({\varvec{X}}) = 0$) into Eq. (26) leads to

$$\begin{aligned} C_{ijkl}^\text {m} \overset{0}{u}_{k,lj} + C_{ijkl}^\text {m} \frac{\partial \varphi _{abk}}{\partial y_l} \overset{0}{u}_{a,bj} + \frac{\partial }{\partial y_j} \big ( C_{ijkl}^\text {m} \varphi _{abk} \big ) \overset{0}{u}_{a,bl} + \frac{\partial }{\partial y_j} \Big ( C_{ijkl}^\text {m} \frac{\partial \overset{2}{u}_k}{\partial y_l} \Big ) + f_i = 0. \end{aligned}$$

(46)

Please note that the body force $\varvec{f}$ keeps unchanged on the micro- and macroscales. We recall the governing equation in the macroscale which reads [3]:

$$\begin{aligned}&\Bigg (\frac{\partial w^\text {M}}{\partial u_{i,j}^\text {M}} - \Big (\frac{\partial w^\text {M}}{\partial u_{i,jk}^\text {M}}\Big )_{,k} \Bigg )_{,j} + f_i= 0, \nonumber \\&C^\text {M}_{ijkl} u^\text {M}_{k,lj} - D_{ijklmn}^\text {M} u_{l,mnkj}^\text {M} + f_i= 0. \end{aligned}$$

(47)

By neglecting the fourth-order term in Eq. (47) and by using $\overset{0}{u}_i({\varvec{X}}) =u^\text {M}_i({\varvec{X}})$, we obtain

$$\begin{aligned} f_i = -C^\text {M}_{ijkl} u^\text {M}_{k,lj} = -C^\text {M}_{ijkl} \overset{0}{u}_{k,lj}. \end{aligned}$$

(48)

Substituting Eq. (48) into Eq. (46) leads to

$$\begin{aligned} \frac{\partial }{\partial y_j} \Big ( C_{ijkl}^\text {m} \frac{\partial \overset{2}{u}_k}{\partial y_l} \Big ) = -\Big ( C_{icab}^\text {m} + C_{ijkl}^\text {m} \frac{\partial \varphi _{abk}}{\partial y_l} \delta _{jc} + \frac{\partial }{\partial y_j} \big ( C_{ijkl}^\text {m} \varphi _{abk} \big ) \delta _{lc} - C^\text {M}_{icab} \Big ) \overset{0}{u}_{a,bc}. \end{aligned}$$

(49)

Because $\overset{0}{u}_{a,bc}$ is constant in $\varvec{y}$, we can introduce $\psi _{abci}$ depending on $\varvec{y}$ and decompose as follows:

$$\begin{aligned} \overset{2}{u}_i = \psi _{abci} \overset{0}{u}_{a,bc} + \overset{2}{\bar{u}}_i({\varvec{X}}), \end{aligned}$$

(50)

where $\psi _{abcd}=\psi _{abcd}(\varvec{y})$ and $\overset{2}{\bar{u}}_i({\varvec{X}})$ are integration constants in $\varvec{y}$. By substituting Eq. (50) (with $\overset{2}{\bar{u}}_i({\varvec{X}}) = 0$) into Eq. (49), it is found that the tensor $\psi _{abcd}$ must fulfill the following equation:

$$\begin{aligned} \frac{\partial }{\partial y_j} \bigg ( C_{ijkl}^\text {m} \Big ( \frac{\partial \psi _{abck}}{\partial y_l} + \varphi _{abk} \delta _{lc} \big ) \bigg ) + C_{ickl}^\text {m} \Big ( \frac{\partial \varphi _{abk}}{\partial y_l} + \delta _{ka} \delta _{lb} \Big ) - {{C}}_{icab}^\text {M} = 0, \end{aligned}$$

(51)

such that Eq. (20) provides

$$\begin{aligned} u^\text {m}_i({\varvec{X}},\varvec{y}) = \overset{0}{u}_i({\varvec{X}}) + \epsilon \varphi _{abi}(\varvec{y}) \overset{0}{u}_{a,b}({\varvec{X}}) + \epsilon ^2 \psi _{abci}(\varvec{y}) \overset{0}{u}_{a,bc}({\varvec{X}}) + \cdots . \end{aligned}$$

(52)