Flow of soft solids squeezed between planar and spherical surfaces

Abstract

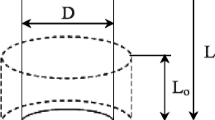
The force to squeeze a Herschel–Bulkley material without slip between two approaching surfaces of various curvature is calculated. The Herschel–Bulkley yield stress requires an infinite force to make plane–plane and plane–concave surfaces touch. However, for plane–convex surfaces this force is finite, which suggests experiments to access the mesoscopic thickness region (1–100 μm) of non-Newtonian materials using squeeze flow between a plate and a convex lens. Compared to the plane–parallel surfaces that are used most often for squeeze flow, the dependence of the separation h′ and approach speed V on the squeezing-time is more complicated. However, when the surfaces become close, a simplification occurs and the near-contact approach speed is found to vary as V ∝ h′⁰ if the Herschel–Bulkley index is n<1/3, and V ∝ h′^(3n-1)/(2n) if n≥ 1/3. Using both plane–plane and plane–convex surfaces, concordant measurements are made of the Herschel–Bulkley index n and yield stress τ₀ for two soft solids. Good agreement is also found between τ₀ measured by the vane and by each squeeze-flow method. However, one of the materials shows a limiting separation and a V(h′) behaviour not predicted by theory for h′<10 μm, possibly owing to an interparticle structure of similar lengthscale.

Appendix

Exact and approximate expressions for I(n, b)

Exact expressions for I(n, b) of Eq. 17, for n=0, 1/2, 1, 3/2, and 2, are compared with the approximation of Eq. 18 to I(n, b) given by Lian et al. (2001), using their parameter $b = R\sqrt {2/\left( {Dh{^\prime }} \right)} = \sqrt {2H{^\prime }/h{^\prime }}$

n=0

$$ I\left( {0,b} \right)_{{\text{Eq 17}}} = 2b - 2\sqrt 2 \tan ^{ - 1} \left( {b/2^{1/2} } \right). $$

$$ I\left( {0,b} \right)_{{\text{Eq}}{\text{. 17}},\,h' \to 0} \cong 2b - \pi \sqrt 2 + \frac{4} {b} - \frac{8} {{3b^2 }} + \cdots \cong 2b - 4.442 + \frac{4} {b} - \frac{8} {{3b^2 }} + \cdots $$

$$ I\left( {0,b} \right)_{{\text{Eq}}{\text{. 18, }}h' \to 0} = 2b - 4.500 + \frac{4} {b}. $$

n=1/2

$$ I\left( {\tfrac{1} {2},b} \right)_{{\text{Eq}}{\text{. 17}}} = \frac{3} {{2^{7/4} }}\ln \left( {\frac{{1 - 2^{1/4} b^{1/2} + 2^{ - 1/2} b}} {{1 + 2^{1/4} b^{1/2} + 2^{ - 1/2} b}}} \right) + \frac{3} {{2^{3/4} }}\tan ^{ - 1} \left( {\frac{{2^{3/4} b^{1/2} }} {{2^{1/2} - b}}} \right) - \frac{{2b^{3/2} }} {{2 + b^2 }}. $$

$$ I\left( {\tfrac{1} {2},b} \right)_{Eq.{\text{ }}17,{\text{ }}h' \to 0} \cong \frac{{3\pi }} {{2^{3/4} }} - \frac{8} {{b^{1/2} }} + \frac{{32}} {{5b^{5/2} }} - \cdots \cong 5.604 - \frac{8} {{b^{1/2} }} + \frac{{32}} {{5b^{5/2} }} - \cdots $$

$$ I\left( {\tfrac{1} {2},b} \right)_{{\text{Eq (18), }}h{^\prime } \to 0} = 5.587 - \frac{8} {{b^{1/2} }} + \frac{{32}} {{5b^{5/2} }}. $$

n=1

$$ I\left( {1,b} \right)_{{\text{Eq}}{\text{. 17}}} = \frac{{b^4 }} {{\left( {2 + b^2 } \right)^2 }}. $$

$$ I\left( {1,b} \right)_{{\text{Eq}}{\text{. 17, }}h' \to 0} \cong 1 - \frac{4} {{b^2 }} + \frac{{12}} {{b^4 }} \cdots $$

$$ I\left( {1,b} \right)_{{\text{Eq}}{\text{. 18, }}h' \to 0} = 0.995 - \frac{4} {{b^2 }} + \frac{{12}} {{b^4 }}. $$

n=3/2

$$ I\left( {\tfrac{3} {2},b} \right)_{{\text{Eq}}{\text{. 17}}} = \frac{5} {{2^{25/4} }}\ln \left( {\frac{{1 + 2^{1/4} b^{1/2} + 2^{ - 1/2} b}} {{1 - 2^{1/4} b^{1/2} + 2^{ - 1/2} b}}} \right) + \frac{5} {{2^{21/4} }}\tan ^{ - 1} \left( {\frac{{2^{5/4} b^{1/2} }} {{2^{1/2} - b}}} \right) + \frac{{\left( {5/24} \right)b^{1/2} }} {{2 + b^2 }} - \frac{{\left( {5/3} \right)b^{1/2} }} {{\left( {2 + b^2 } \right)^2 }} - \frac{{\left( {8/3} \right)b^{5/2} }} {{\left( {2 + b^2 } \right)^3 }}. $$

$$ I\left( {\tfrac{3} {2},b} \right)_{{\text{Eq}}{\text{. }}17,{\text{ }}h' \to 0} \cong \frac{{5\pi }} {{2^{21/4} }} - \frac{{32}} {{7b^{7/2} }} + \frac{{47}} {{2b^{11/2} }} - \cdots \cong 0.4128 - \frac{{32}} {{7b^{7/2} }} + \frac{{23.5}} {{b^{11/2} }} - \cdots $$

$$ I\left( {\tfrac{3} {2},b} \right)_{{\text{Eq}}{\text{. 18, }}h' \to 0} = 0.4122 - \frac{{32}} {{7b^{1/2} }} + \frac{{23.273}} {{b^{11/2} }}. $$

n=2

$$ I\left( {2,b} \right)_{{\text{Eq}}{\text{. 17}}} = \frac{3} {{2^{9/2} }}\tan ^{ - 1} \left( {\frac{b} {{2^{1/2} }}} \right) + \frac{{b\left( {10 + 3b^2 } \right)}} {{16\left( {2 + b^2 } \right)^2 }} - \frac{{2b\left( {2 + 3b^2 } \right)}} {{\left( {2 + b^2 } \right)^4 }}. $$

$$ I\left( {2,b} \right)_{{\text{Eq}}{\text{. }}17,{\text{ }}h' \to 0} \cong \frac{{3\pi }} {{2^{11/2} }} - \frac{{32}} {{5b^5 }} + \frac{{320}} {{7b^7 }} \cdots \cong 0.2083 - \frac{{32}} {{5b^5 }} + \frac{{320}} {{7b^7 }} \cdots $$

$$ I\left( {2,b} \right)_{{\text{Eq}}{\text{. 18, }}h' \to 0} = 0.2104 - \frac{{32}} {{5b^5 }} + \frac{{320}} {{7b^7 }}. $$