An inventory model with trade-credit policy and variable deterioration for fixed lifetime products

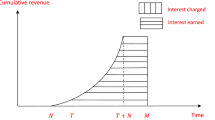
Abstract

The purpose of this study is two-fold. The first is to consider supplier’s and retailer’s trade-credit policy for fixed lifetime products and the second is to extend Mahata’s 2012 model with time varying deterioration where Mahata (Expert Syst Appl 39(3):3537–3550, 2012) wrote exponential deterioration but actually he considered constant deterioration. We assume that the suppliers offer full trade-credit to retailers but retailers offer partial trade-credit to their customers. Some numerical examples along with graphical representations are given to illustrate the model.

Appendices

Appendix 1

$$\begin{aligned} x_1&= \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1 -\ln (1+L)\frac{(1+L)^2}{2},\\ y_1&= -\!\ln (1\!+\!L\!-\!T)\frac{(1\!+\!L\!-\!T)^2}{2}\!+\!\left( \frac{\ln (1\!+\!L\!-\!T)}{2} +\frac{1}{4}\right) (2+2L-t_1-T)(t_1-T),\\ x_2&= \left( \frac{\ln (1+L)}{2}-\frac{1}{4}\right) (2+2L-t_1-M) (t_1-M)-\ln (1+L-M)\frac{(1+L-M)^2}{2}, \end{aligned}$$

and

$$\begin{aligned} y_2&= [\!-\!(T\!-\!M)(2\!+\!2L\!-\!M\!-\!T)\left( \frac{1}{2}\!+\!\ln (1+L-T)\right) +\ln (1+L-M)(1+L-M)^2\\&\quad -\ln (1+L-T)(1+L-T)^2]. \end{aligned}$$

Appendix 2

Case 1 $M\ge N$

Case 1.1 $M\le t_1$ i.e., $M\le t_M\le T$

$$\begin{aligned} \frac{dTRC_1(T)}{dT}=\frac{f_1(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_1(T)&= (h+cI_c)DT\Big [(2+2L-t_1-T)\left( \frac{(t_1-T)}{2(1+L-T)} -\frac{\ln (1+L-T)}{2}\right) \\&\quad -\,(1+L-T)-\ln (1+L-T)(t_1+1+L-2T)\Big ]\nonumber \\&\quad -\,A-h(P-D)\left[ \Big (\frac{1}{4}+\frac{\ln (1+L)}{2}\Big )(2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \nonumber \\&\quad -\,\left( \frac{hP}{2}+cI_cP\right) (1+L-t_1)^2\frac{\ln (1+L-t_1)}{2}\\&\quad -\,(h+cI_c)D\Big [-(1+L-T)^2\frac{\ln (1+L-T)}{2}\nonumber \\&\quad +\,\left( \frac{\ln (1+L-T)}{2}+\frac{1}{4}\right) (2+2L-t_1-T)(t_1 -T)\Big ]\\&\quad -\,cPt_1+\frac{sI_eD}{2}(M^2-(1-\alpha )N^2)-cI_c(P-D)\Bigg [\left( \frac{\ln (1+L)}{2}-\frac{1}{4}\right) \\&\quad \times (2+2L-t_1-M)(t_1-M)- \ln (1+L-M)\frac{(1+L-M)^2}{2}\Bigg ]\\&\quad -\, cI_cD\Bigg [\Big (\frac{1}{4}+\frac{\ln (1+L-T)}{2}\Big )(2+2L-t_1-T)(T-t_1)\\&\quad +\ln (1+L-T)\frac{(1+L-T)^2}{2}\Bigg ] \end{aligned}$$

To determine the optimal value of $T$ say $T^*_1$, we solve the equation $f_1(T)=0$.

We obtain $\frac{df_1(T)}{dT}>0 ~~\hbox {if} ~~T>0$. As $f_1(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_1(T)}{dT}$ is an increasing function on $[0,\infty )$. Using Lemma, $TRC_1(T)$ is a convex function on $[0,\infty )$.

In addition, we have as $\lim T\rightarrow \infty$, then $f_1(T)\rightarrow \infty$.

$$\begin{aligned} f_1(0)&= -\Big (A+h(P-D)\left[ \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \nonumber \\&\quad +\,\left( \frac{hP}{2}+cI_cP\right) \frac{(1+L-t_1)^2}{2}\ln (1+L-t_1)+hD\Bigg [-\ln (1+L)\frac{(1+L)^2}{2}\nonumber \\&\quad +\,\Big (\frac{\ln (1+L)}{2}+\frac{1}{4}\Big )(2+2L-t_1)t_1\Bigg ] -\frac{sI_eD(M^2-(1-\alpha )N^2)}{2} +cPt_1\nonumber \\&\quad +\,cI_c(P-D)\Big [\Big (\frac{\ln (1+L)}{2}-\frac{1}{4}\Big )(2+2L-t_1-M)(t_1-M)\\&\quad -\,\frac{\ln (1+L-M)}{2}(1+L-M)^2 \Big ]+\frac{cI_cP(1+L-t_1)^2}{2}\ln (1+L-t_1)\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_1(T)}{dT}&< 0; ~~~\hbox {if}~~~~~ T\in [0,T^*_1),\nonumber \\&= 0;~~~\hbox {if}~~~~~ T=T^*_1,\nonumber \\&> 0;~~~\hbox {if}~~~~~ T\in (T^*_1,\infty ). \end{aligned}$$

By applying the intermediate value theorem, we can state that the optimal solution $T^*_1$ exists and is unique.

Case 1. (b) $M\le T\le t_M$

$$\begin{aligned} \frac{dTRC_2(T)}{dT}=\frac{f_2(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_2(T)&= -A-h(P-D)\left[ \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \nonumber \\&\quad -\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)+ hD\Big [\ln (1+L-T)\frac{(1+L-T)^2}{2}\nonumber \\&\quad -\,\Big (\frac{1}{4}+\frac{\ln (1+L-T)}{2}\Big )(2+2L-t_1-T)(t_1-T)\Big ]-\frac{DcI_c}{2} \Big [-(T-M)\nonumber \\&\quad \times (2+2L-M-T)\left( \frac{1}{2}+\ln (1+L-T)\right) +\ln (1+L-M)(1+L-M)^2\nonumber \\&\quad -\,(1+L-T)^2\ln (1+L-T)\Big ] +\frac{sI_eD}{2}[M^2-(1-\alpha )N^2]-cPt_1\nonumber \\&\quad +\,\frac{DcI_cT}{2} \Big [(2T+M-2L-2)-(3T-M-2L-2)\ln (1+L-T)\nonumber \\&\quad -\,(2L+2-T-M)\Big ((T-M)\frac{1}{1+L-T}+\ln (1+L-T)\Big )\Big ] \end{aligned}$$

To determine the optimal value of $T$ say $T^*_2$, we solve the equation $f_2(T)=0$.

We obtain $\frac{df_2(T)}{dT}>0,~~\hbox {if} ~~T>0.$

As $f_2(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_2(T)}{dT}$ is an increasing function on $[0,\infty )$. Using Lemma, $TRC_2(T)$ is a convex function on $[0,\infty )$.

In addition, we obtain as $\lim T\rightarrow \infty$, then $f_2(T)\rightarrow \infty$.

$$\begin{aligned} f_2(0)&= -\Big (A+h(P-D)\left[ \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \nonumber \\&\quad +\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)- hD\Big [\ln (1+L)\frac{(1+L)^2}{2}-\Big (\frac{\ln (1+L)}{2}\nonumber \\&\quad -\,\frac{1}{4}\Big )(2+2L-t_1)t_1\Big ]+\frac{DcI_c}{2} \Big [M(2+2L-M)\left( \frac{1}{2}+\ln (1+L)\right) +(1+L\nonumber \\&\quad -\,M)^2\ln (1+L-M)-\ln (1+L)(1+L)^2\Big ] -\frac{sI_eD}{2}[M^2-(1-\alpha )N^2]\nonumber \\&\quad +\,cPt_1\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_2(T)}{dT}&< 0; ~~~\hbox {if}~~ T\in [0,T^*_2),\nonumber \\&= 0;~~~\hbox {if}~~ T=T^*_2,\nonumber \\&> 0;~~~\hbox {if}~~ T\in (T^*_2,\infty ). \end{aligned}$$

Using intermediate value theorem, we can say that a unique optimal solution $T^*_2$ exists.

Case 1. (c) $N\le T\le M$

$$\begin{aligned} \frac{dTRC_3(T)}{dT}=\frac{f_3(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_3(T)&= hDT\Big [(2+2L-t_1-T)\left( \frac{(t_1-T)}{2(1+L-T)}-\frac{\ln (1+L-T)}{2}\right) -(1+L-T)\nonumber \\&\quad -\,\ln (1+L-T)(t_1+1+L-2T)\Big ] -A-h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) \nonumber \\&\quad (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ] -\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1) \nonumber \\&\quad -hD\Big [\!-\ln (1\!+\!L\!-\!T)\frac{(1\!+\!L\!-\!T)^2}{2}\!+\!\left( \frac{\ln (1+L-T)}{2} +\frac{1}{4}\right) (2+2L-t_1-T) \nonumber \\&\quad (t_1-T)\Big ] -\frac{sI_eD}{2}(1-\alpha )N^2+\frac{sI_eDT^2}{2}-cPt_1 \end{aligned}$$

To determine the optimal value of $T$ say $T^*_3$, we solve the equation $f_3(T)=0.$

We obtain $\frac{df_3(T)}{dT}>0 ~~\hbox {if}~~T>0.$

As $f_3(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_3(T)}{dT}$ is an increasing function on $[0,\infty )$. Using the statement of Lemma, $TRC_3(T)$ is a convex function on $[0,\infty )$.

In addition, we find as $\lim T\rightarrow \infty$, then $f_3(T)\rightarrow \infty$.

Now

$$\begin{aligned} f_3(0)&= -\Big (A+h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ] \nonumber \\&\quad +\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)+hD\Big [-\ln (1+L)\frac{(1+L)^2}{2}+\Big (\frac{\ln (1+L)}{2} \nonumber \\&\quad +\,\frac{1}{4}\Big )(2+2L-t_1)t_1\Big ]+\frac{sI_eD}{2}(1-\alpha )N^2+cPt_1\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_3(T)}{dT}&< 0; ~~~~\hbox {if}~~~~ T\in [0,T^*_3),\nonumber \\&= 0;~~~~\hbox {if}~~~~ T=T^*_3,\nonumber \\&> 0;~~~~\hbox {if}~~~~ T\in (T^*_3,\infty ) \end{aligned}$$

Again using the intermediate value theorem, we conclude that a unique optimal solution $T^*_3$ exists.

Case 1. (d) $0<T\le N$

$$\begin{aligned} \frac{dTRC_4(T)}{dT}=\frac{f_4(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_4(T)&= hDT\Big [(2+2L-t_1-T)\left( \frac{(t_1-T)}{2(1+L-T)}-\frac{\ln (1+L-T)}{2}\right) -(1+L-T)\nonumber \\&\quad -\,\ln (1+L-T)(t_1+1+L-2T)\Big ] -A-h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) \nonumber \\&\quad (2+\,2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ] \!-\!\frac{hP(1\!+\!L\!-\!t_1)^2}{2}\ln (1+L-t_1)\!-\!hD \nonumber \\&\quad \Big [-\,\ln (1+L-T)\frac{(1+L-T)^2}{2}+\left( \frac{\ln (1+L-T)}{2} +\frac{1}{4}\right) (2+2L-t_1-T) \nonumber \\&\quad (t_1-\,T)\Big ]+\frac{sI_eD\alpha T^2}{2}-cPt_1 \end{aligned}$$

To determine the optimal value of $T$ say $T^*_4$, we solve the equation $f_4(T)=0$.

We obtain $\frac{df_4(T)}{dT}>0 ~~\hbox {if}~~ T>0.$

As $f_4(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_4(T)}{dT}$ is an increasing function on $[0,\infty )$. Using the Lemma, $TRC_4(T)$ is a convex function on $[0,\infty )$.

In addition, we know as $\lim T\rightarrow \infty$, then $f_4(T)\rightarrow \infty$.

$$\begin{aligned} f_4(0)&= -\Big (A+h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ] \nonumber \\&\quad +\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)+hD\Big [-\ln (1+L)\frac{(1+L)^2}{2}+\Big (\frac{\ln (1+L)}{2} \nonumber \\&\quad +\,\frac{1}{4}\Big )(2+2L-t_1)t_1\Big ]+cPt_1\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_4(T)}{dT}&< 0; ~~~\hbox {if} ~~T\in [0,T^*_4),\nonumber \\&= 0;~~~\hbox {if}~~ T=T^*_4,\nonumber \\&> 0;~~~\hbox {if}~~ T\in (T^*_4,\infty ) \end{aligned}$$

Using the intermediate value theorem, we can say that a unique optimal solution $T^*_4$ exists.

Case 2 $M<N$

Case 2. (a) $T\ge t_M$

$$\begin{aligned} \frac{dTRC_5(T)}{dT}=\frac{f_5(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_5(T)&= (h+cI_c)DT\Big [(2+2L-t_1-T)\left( \frac{(t_1-T)}{2(1+L-T)} -\frac{\ln (1+L-T)}{2}\right) \nonumber \\&\quad -\,(1+L-T)-\ln (1+L-T)(t_1+1+L-2T)\Big ] -A-h(P-D)\nonumber \\&\quad \Big [\Big (\frac{1}{4}+\,\frac{\ln (1+L)}{2}\Big )(2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ] -\Big (\frac{hP}{2}+cI_cP\Big )\nonumber \\&\quad (1+\,L-t_1)^2 \frac{\ln (1+L-t_1)}{2}-(h+cI_c)D\Big [ -\ln (1+L-T)\frac{(1+L-T)^2}{2}\nonumber \\&\quad +\,\left( \frac{\ln (1+L-T)}{2}+\frac{1}{4}\right) (2+2L-t_1-T)(t_1 -T)\Big ]+\frac{sI_eD\alpha M^2}{2}-cPt_1\nonumber \\&\quad -\,cI_c(P-D)\Big [\Big (-\frac{1}{4}+\frac{ \ln (1+L)}{2}\Big )(2+2L-t_1-M)(t_1-M)\nonumber \\&\quad -\, \ln (1+L-M)\frac{(1+L-M)^2}{2}\Big ]- cI_cD\Big [\Big (\frac{1}{4}+\frac{\ln (1+L-T)}{2}\Big )\nonumber \\&\quad (2+\,2L-t_1-T)(T-t_1)+\ln (1+L-T)\frac{(1+L-T)^2}{2}\Big ] \end{aligned}$$

To determine the optimal value of $T$ say $T^*_5$, we solve the equation $f_5(T)=0$.

We obtain $\frac{df_5(T)}{dT}>0 ~~\hbox {if} ~~T>0$. As $f_5(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_5(T)}{dT}$ is an increasing function on $[0,\infty )$. Using Lemma, $TRC_5(T)$ is a convex function on $[0,\infty )$.

In addition, we obtain as $\lim T\rightarrow \infty$, then $f_5(T)\rightarrow \infty$.

$$\begin{aligned} f_5(0)&= -\Big (A+h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ]\\&\quad +\,\left( \frac{hP}{2}+cI_cP\right) \frac{(1+L-t_1)^2}{2}\ln (1+L-t_1)+hD\Big [-\ln (1+L)\frac{(1+L)^2}{2}\\&\quad +\,\Big (\frac{\ln (1+L)}{2}+\frac{1}{4}\Big )(2+2L{-}t_1)t_1\Big ]{-}\frac{sI_eD\alpha M^2}{2} +cPt_1+cI_c(P-D)\Big [\Big ({-}\frac{1}{4}\\&\quad +\,\frac{\ln (1+L)}{2}\Big ) (2+2L-t_1-M)(t_1-M)- \ln (1+L-M)\frac{(1+L-M)^2}{2}\Big ]\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_5(T)}{dT}&< 0;\quad ~~~\hbox {if}~~~~~ T\in [0,T^*_5),\\&= 0;~~~\quad \hbox {if}~~~~~ T=T^*_5,\\&> 0;~~~\quad \hbox {if}~~~~~ T\in (T^*_5,\infty ) \end{aligned}$$

Using the intermediate value theorem, we can state that a unique optimal solution $T^*_5$ exists.

Case 2. (b) $M\le T\le t_M$

$$\begin{aligned} \frac{dTRC_6(T)}{dT}=\frac{f_6(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_6(T)&= -\Big (A+h(P-D)\left[ \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \nonumber \\&\quad +\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)- hD\Big [\ln (1+L-T)\frac{(1+L-T)^2}{2}-\Big (\frac{1}{4}\nonumber \\&\quad +\,\frac{\ln (1+L-T)}{2}\Big )(2+2L-t_1-T)(t_1-T)\Big ]+\frac{DcI_c}{2} \Big [-(T-M)(2+2L\nonumber \\&\quad -\,M-T)\left( \frac{1}{2}+\ln (1+L-T)\right) +\ln (1+L-M)(1+L-M)^2\nonumber \\&\quad -\,(1+L-T)^2\ln (1+L-T)\Big ] -\frac{sI_eDM^2\alpha }{2}+cPt_1-\frac{DcI_cT}{2} \Big [(2T+M\nonumber \\&\quad -\,2L-2)-(3T-M-2L-2)\ln (1+L-T)-(2L+2-T-M)\Big ((T\nonumber \\&\quad -\,M)\frac{1}{1+L-T}+\ln (1+L-T)\Big )\Big ]\Big ) \end{aligned}$$

To determine the optimal value of $T$ say $T^*_6$, we solve the equation $f_6(T)=0$.

We obtain $\frac{df_6(T)}{dT}>0 ~~\hbox {if} ~~T>0.$

As $f_6(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_6(T)}{dT}$ is an increasing function on $[0,\infty )$. Using Lemma, $TRC_6(T)$ is a convex function on $[0,\infty )$.

In addition, we find as $\lim T\rightarrow \infty$, then $f_6(T)\rightarrow \infty$.

$$\begin{aligned} f_6(0)&= -A-h(P-D)\left[ \left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\right] \\&\quad -\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)+ hD\Big [\ln (1+L)\frac{(1+L)^2}{2}-\Big (\frac{\ln (1+L)}{2}\\&\quad -\,\frac{1}{4}\Big )(2+2L-t_1)t_1\Big ]-\frac{DcI_c}{2} \Big [M(2+2L-M)\left( \frac{1}{2}+\ln (1+L)\right) \\&\quad +\,(1+L-M)^2\ln (1+L-M)-\ln (1+L)(1+L)^2\Big ] +\frac{sI_eDM^2\alpha }{2}-cPt_1 \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_6(T)}{dT}&< 0; ~~~\hbox {if}~~ T\in [0,T^*_6),\\&= 0;~~~\hbox {if}~~ T=T^*_6,\\&> 0;~~~\hbox {if}~~ T\in (T^*_6,\infty ) \end{aligned}$$

Using the intermediate value theorem, we can state that a unique optimal solution $T^*_6$ exists.

Case 2. (c) $0<T\le M$

$$\begin{aligned} \frac{dTRC_7(T)}{dT}=\frac{f_7(T)}{T^2} \end{aligned}$$

where

$$\begin{aligned} f_7(T)&= hDT\Big [(2+2L-t_1-T)\left( \frac{(t_1-T)}{2(1+L-T)} -\frac{\ln (1+L-T)}{2}\right) -(1+L-T)\nonumber \\&\quad -\,\ln (1+L-T)(t_1+1+L-2T)\Big ] -A-h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) \\&\quad (2+2L-t_1)t_1\!-\!\ln (1+L)\frac{(1+L)^2}{2}\Big ] \!-\!\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)\!-\!hD\\&\quad \Big [-\ln (1\!+L\!-T)\frac{(1\!+L\!-T)^2}{2}\!+\!\left( \frac{\ln (1+L-T)}{2} +\frac{1}{4}\right) (2+2L-t_1-T)(t_1\\&\quad -\,T)\Big ]+\frac{sI_eDT^2\alpha }{2}-cPt_1 \end{aligned}$$

To determine the optimal value of $T$ say $T^*_7$, we solve the equation $f_7(T)=0.$

We obtain $\frac{df_7(T)}{dT}>0, ~~\hbox {if}~~T>0.$

As $f_7(T)$ is an increasing function on $[0,\infty )$, then $\frac{dTRC_7(T)}{dT}$ is an increasing function on $[0,\infty )$. Using the Lemma, $TRC_7(T)$ is a convex function on $[0,\infty )$.

In addition, as $\lim T\rightarrow \infty$, then $f_7(T)\rightarrow \infty$.

Now

$$\begin{aligned} f_7(0)&= -\Big (A+h(P-D)\Big [\left( \frac{\ln (1+L)}{2}+\frac{1}{4}\right) (2+2L-t_1)t_1-\ln (1+L)\frac{(1+L)^2}{2}\Big ]\\&\quad +\,\frac{hP(1+L-t_1)^2}{2}\ln (1+L-t_1)+hD\Big [-\ln (1+L)\frac{(1+L)^2}{2}+\Big (\frac{\ln (1+L)}{2}\\&\quad +\,\frac{1}{4}\Big )(2+2L-t_1)t_1\Big ]+cPt_1\Big ) \end{aligned}$$

Then

$$\begin{aligned} \frac{dTRC_7(T)}{dT}&< 0; ~~~~\hbox {if}~~~~ T\in [0,T^*_7),\\&= 0;~~~~\hbox {if}~~~~ T=T^*_7,\\&> 0;~~~~\hbox {if}~~~~ T\in (T^*_7,\infty ) \end{aligned}$$

Using the intermediate value theorem, we can state that a unique optimal solution $T^*_7$ exists.