An inventory model under price and stock dependent demand for controllable deterioration rate with shortages and preservation technology investment

Abstract

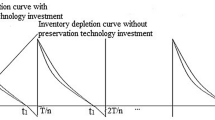
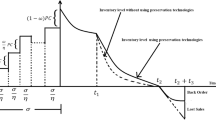
This paper develops an EOQ inventory model that considers the demand rate as a function of stock and selling price. Shortages are permitted and two cases are studied: (i) complete backordering and (ii) partial backordering. The inventory model is for a deteriorating seasonal product. The product’s deterioration rate is controlled by investing in the preservation technology. The main purpose of the inventory model is to determine the optimum selling price, ordering frequency and preservation technology investment that maximizes the total profit. Additionally, the paper proves that the total profit is a concave function of selling price, ordering frequency and preservation technology investment. Therefore, a simple algorithm is proposed to obtain the optimal values for the decision variables. Several numerical examples are solved and studied along with a sensitivity analysis.

Appendices

Appendix 1: Proof of Theorem 1

The first and second partial derivatives of the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (17) with respect to n are given below:

$$\begin{aligned} \frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial n}= & {} -\frac{cD(p)}{\lambda (\alpha )+\beta }\left( {1-\frac{\gamma ^{2}T^{2}}{2n^{2}}(\lambda (\alpha )+\beta )^{2}} \right) +hD(p)\left( {\frac{\gamma ^{2}T^{2}}{2n^{2}}} \right) \nonumber \\&-sD(p)\left( {-\frac{\gamma T^{2}}{n^{2}}+\frac{\gamma ^{2}T^{2}}{2n^{2}}+\frac{T^{2}}{n^{2}}} \right) -\frac{dD(p)}{\lambda (\alpha )+\beta }\left( {1-\frac{\gamma ^{2}T^{2}}{2n^{2}}(\lambda (\alpha )+\beta )^{2}} \right) ,\nonumber \\&+\frac{\beta dD(p)\gamma ^{2}T^{2}}{2n^{2}}-A \end{aligned}$$

(28)

$$\begin{aligned} \frac{\partial ^{2}(\textit{TP}(n,\alpha ,p))}{\partial n^{2}}= & {} -cD(p)\left( {\frac{\gamma ^{2}T^{2}}{n^{3}}(\lambda (\alpha )+\beta )} \right) -hD(p)\left( {\frac{\gamma ^{2}T^{2}}{n^{3}}} \right) -\frac{\beta dD(p)\gamma ^{2}T^{2}}{n^{3}} \nonumber \\&-\frac{sD(p)T^{2}}{n^{3}}\left( {2+\gamma (\gamma -2)} \right)<0 ,\; 0<\gamma <1. \end{aligned}$$

(29)

Clearly, from Eq. (29) it is concluded that the profit function given by Eq. (17) is concave in n. Notice that n must be an integer number. Thus, the determination of the optimal n is reduced to obtain a local optimal solution for n.

This completes the Proof of Theorem 1. $\square$

Appendix 2: Proof of Theorem 2

The first and second partial derivatives of the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (17) with respect to $\alpha$ are as follows,

$$\begin{aligned} \frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial \alpha }= & {} \frac{(c+d)\gamma ^{2}T^{2}\delta \lambda (\alpha )D(p)}{2n}-T, \end{aligned}$$

(30)

$$\begin{aligned} \frac{\partial ^{2}(\textit{TP}(n,\alpha ,p))}{\partial \alpha ^{2}}= & {} -\frac{(c+d)\gamma ^{2}T^{2}\delta ^{2}\lambda (\alpha )D(p)}{2n}-T<0. \end{aligned}$$

(31)

For straightforwardness, set $H(\alpha )=\frac{(c+d)\gamma ^{2}T^{2}\delta \lambda (\alpha )D(p)}{2n}-T$

$$\begin{aligned} \mathrm{State}\; \Delta _1 (n,p)= & {} \left. {H(\alpha )} \right| _{\alpha =0} =\frac{(c+d)\gamma ^{2}T^{2}\delta \lambda _0 D(p)}{2n}-T\\ \mathrm{and } \Delta _2 (n,p)= & {} \left. {H(\alpha )} \right| _{\alpha =\bar{{\alpha }}} =\frac{(c+d)\gamma ^{2}T^{2}\delta \lambda (\bar{{\alpha }})D(p)}{2n}-T. \end{aligned}$$

It is understandable that ${H}'(\alpha )<0$. Consequently $H(\alpha )$ is strictly decreasing in $\alpha$.

• (1) $If\;\Delta _1 (n,p)\le 0, H(\alpha )\le 0$ and $\forall \alpha \in \left[ {0,\bar{{\alpha }}} \right]$ then $\textit{TP}(n,\alpha ,p)$ is decreasing in $\alpha \in \left[ {0,\bar{{\alpha }}} \right]$. Thus, the optimal preservation cost is $\alpha ^{*}=0.$

• (2) $If\;\Delta _2 (n,p)\ge 0, H(\alpha )\ge 0$ and $\forall \alpha \in \left[ {0,\bar{{\alpha }}} \right]$ then $\textit{TP}(n,\alpha ,p)$ is increasing in $\alpha \in \left[ {0,\bar{{\alpha }}} \right]$.Therefore, the optimal preservation cost is $\alpha ^{*}=\bar{{\alpha }}.$

• (3) $If\;\Delta _1 (n,p)>0$ and $\Delta _2 (n,p)<0$ then, according to the intermediate value theorem, there is an unique value $\alpha \in \left( {0,\bar{{\alpha }}} \right)$ that satisfies $H(\alpha ^{*})=0 ,$ Thus,

  $$\begin{aligned} \frac{(c+d)\gamma ^{2}T^{2}\delta \lambda (\alpha ^{*})D(p)}{2n}-T=0. \end{aligned}$$

  (32)

This completes the Proof of Theorem 2. $\square$

Appendix 3: Proof of Theorem 3

The first and second partial derivatives of the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (17) with respect to p are presented below:

$$\begin{aligned} \begin{aligned} \frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial p}=&(a-2bp)T+\frac{cb}{\lambda (\alpha )+\beta }\left( {n+\frac{\gamma ^{2}T^{2}}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )T-\gamma T} \right) \\&+\,hb\left( {\frac{\gamma ^{2}T^{2}}{2n}} \right) +sb\left( {\frac{\gamma T^{2}}{n}-\frac{\gamma ^{2}T^{2}}{2n}-\frac{T^{2}}{n}} \right) +\frac{db}{\lambda (\alpha )+\beta }\\&\left\{ {n+\frac{\gamma ^{2}T^{2}}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )T-\gamma T} \right\} bd\gamma T+\frac{\beta db\gamma ^{2}T^{2}}{2n} \\ \end{aligned} \end{aligned}$$

(33)

Set $\frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial p}=0$ and solve it for the optimal $p^{*}$;

$$\begin{aligned}&\begin{aligned} \Rightarrow p^{*}=&\frac{c}{2(\lambda (\alpha )+\beta )}\left( {\frac{n}{T}+\frac{\gamma ^{2}T}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )-\gamma } \right) +\frac{h}{2}\left( {\frac{\gamma ^{2}T}{2n}} \right) \\&+\,\frac{s}{2}\left( {\frac{\gamma T}{n}-\frac{\gamma ^{2}T}{2n}-\frac{T}{n}} \right) +\frac{d}{2(\lambda (\alpha )+\beta )}\\&\left( {\frac{n}{T}+\frac{\gamma ^{2}T}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )-\gamma } \right) +\frac{d\gamma }{2}+\frac{\beta d\gamma ^{2}T}{4n}+\frac{a}{2b} . \\ \end{aligned} \end{aligned}$$

(34)

$$\begin{aligned}&\frac{\partial ^{2}\left( {\textit{TP}\left( {n,\alpha ,p} \right) } \right) }{\partial p^{2}}=-2bT<0 \end{aligned}$$

(35)

Hence, $p^{*}$ is the global optimal that maximizes the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (17) for fixed values of n and $\alpha$.

This completes the Proof of Theorem 3. $\square$

Appendix 4: Proof of Theorem 5

The first and second partial derivatives of the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (27) with respect to n are given below:

$$\begin{aligned}&\begin{aligned} \frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial n}=&-\frac{cD(p)}{\lambda (\alpha )+\beta }\left( {1-\frac{\gamma ^{2}T^{2}}{2n^{2}}(\lambda (\alpha )+\beta )^{2}} \right) +hD(p)\left( {\frac{\gamma ^{2}T^{2}}{2n^{2}}} \right) \\&+\,sD(p)\left( {1-\gamma } \right) ^{2}\frac{\eta T^{2}}{2n^{2}}+c_1 D(p)\left( {1-\gamma } \right) ^{2}\frac{\eta T^{2}}{2n^{2}} \\&-\frac{dD(p)}{\lambda (\alpha )+\beta }\left( {1-\frac{\gamma ^{2}T^{2}}{2n^{2}}(\lambda (\alpha )+\beta )^{2}} \right) +\frac{\beta dD(p)\gamma ^{2}T^{2}}{2n^{2}}-A \\ \end{aligned} \end{aligned}$$

(36)

$$\begin{aligned}&\begin{aligned} \frac{\partial ^{2}(\textit{TP}(n,\alpha ,p))}{\partial n^{2}}=&-cD(p)\left( {\frac{\gamma ^{2}T^{2}}{n^{3}}(\lambda (\alpha )+\beta )} \right) -hD(p)\left( {\frac{\gamma ^{2}T^{2}}{n^{3}}} \right) -\frac{\beta dD(p)\gamma ^{2}T^{2}}{n^{3}} \\&-\,\left( {s+c_1 } \right) D(p)\left( {1-\gamma } \right) ^{2}\frac{\eta T^{2}}{n^{3}}<0 ,\; 0<\gamma <1. \\ \end{aligned} \end{aligned}$$

(37)

Clearly, the profit function given by Eq. (27) is concave in n.

This completes the Proof of Theorem 5. $\square$

Appendix 5: Proof of Theorem 7

The first and second partial derivatives of the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (27) with respect to p are presented below:

$$\begin{aligned} \begin{aligned} \frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial p}=&(a-2bp)T+\frac{cb}{\lambda (\alpha )+\beta }\left( {n+\frac{\gamma ^{2}T^{2}}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )T-\gamma T} \right) \\&+\,hb\left( {\frac{\gamma ^{2}T^{2}}{2n}} \right) +\left( {s+c_1 } \right) nb\left( {1-\gamma } \right) ^{2}\frac{\eta T^{2}}{n^{2}} \\&+\frac{db}{\lambda (\alpha )+\beta }\left\{ {n+\frac{\gamma ^{2}T^{2}}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )T-\gamma T} \right\} \\&bd\gamma T+\frac{\beta db\gamma ^{2}T^{2}}{2n} \end{aligned} \end{aligned}$$

(38)

Set $\frac{\partial (\textit{TP}(n,\alpha ,p))}{\partial p}=0$ and solve it for the optimal $p^{*}$;

$$\begin{aligned}&\begin{aligned} \Rightarrow p^{*}=&\frac{c}{2(\lambda (\alpha )+\beta )}\left( {\frac{n}{T}+\frac{\gamma ^{2}T}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )-\gamma } \right) +\frac{h}{2}\left( {\frac{\gamma ^{2}T}{2n}} \right) \\&+\,\left( {s+c_1 } \right) n\left( {1-\gamma } \right) ^{2}\frac{\eta T^{2}}{n^{2}}+\frac{d}{2(\lambda (\alpha )+\beta )}\\ {}&\left( {\frac{n}{T}+\frac{\gamma ^{2}T}{2n}(\lambda (\alpha )+\beta )^{2}+(\lambda (\alpha )+\beta )-\gamma } \right) +\frac{d\gamma }{2}+\frac{\beta d\gamma ^{2}T}{4n}+\frac{a}{2b} . \\ \end{aligned} \end{aligned}$$

(39)

$$\begin{aligned}&\frac{\partial ^{2}\left( {\textit{TP}\left( {n,\alpha ,p} \right) } \right) }{\partial p^{2}}=-2bT<0 \end{aligned}$$

(40)

Hence, $p^{*}$ is the global optimal that maximizes the profit function $\textit{TP}(n,\alpha ,p)$ given by Eq. (27) for fixed values of n and $\alpha$.

This completes the Proof of Theorem 7. $\square$

Appendix 6: Algorithm for partial backlogging

Algorithm for partial backlogging

• Step 1. Initialize $n=1.$

• Step 2. Initialize $m=1$ and set the value of $p^{m}=p_0 .$

• Step 3. Compute $\Delta _1 (n,p),\Delta _2 (n,p)$ and perform any one of the following three cases 1, 2 or 3.

  • (1) If $\Delta _1 (n,p)\le 0,$ then $\alpha _1^m =0$. Determine $p_1^m$ from Eq. (39).

  • (2) If $\Delta _2 (n,p)\ge 0,$ then $\alpha _1^m =\bar{{\alpha }}$. Calculate $p_1^m$ from Eq. (39).

  • (3) If $\Delta _1 (n,p)>0$ and $\Delta _2 (n,p)<0$, Compute $\alpha _1^m$ by solving (32). Substitute the value of $\alpha _1^m$ into Eq. (39) and determine the corresponding value for $p_1^m$.

  Set $p^{m+1}=p_1^m$ and $\alpha ^{m}=\alpha _1^m$.

• Step 4. If $\left| {p^{m+1}-p^{m}} \right| \le 10^{-4}$, then $(\alpha ^{*},p^{*})=(\alpha ^{m},p^{m+1})$ and go to Step 5. Otherwise, set $m=m+1$ and go to Step 3.

• Set 5. Compute $\textit{TP}(n,\alpha ^{*},p^{*})$ with Eq. (27) which is the maximum for the profit function for a fixed value of n.

• Step 6. Set ${n}'=n+1$, repeat Step 2 to 5 and find $\textit{TP}({n}',\alpha ^{*},p^{*})$ with Eq. (27). Go to Step 7.

• Step 7. If $\textit{TP}({n}',\alpha ^{*},p^{*})\ge \textit{TP}(n,\alpha ^{*},p^{*})$, set $n={n}'$. Go to Step 6. Otherwise go to Step 8.

• Step 8. Set $(n^{*},\alpha ^{*},p^{*})=(n,\alpha ^{*},p^{*})$ and $\textit{TP}(n,\alpha ^{*},p^{*})$ as the optimal solution.

• Step 9. Compute the order quantity Q with Eq. (22).

• Step 10. Calculate shortage level using Eq. (21).

• Step 11. Determine the quantity of deteriorated items with Eq. (5).