Abstract
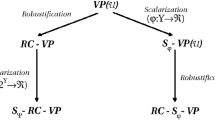
In this article, we consider vector optimization problems with uncertain data. We first formulate optimistic counterparts of the reference problems and propose concepts of efficient solutions to such counterparts. We then introduce concepts of pointwise and global well-posedness for optimistic counterparts. Using the generalized Gerstewitz’s function and properties of elements in the image space, we establish the relationships between well-posedness properties for the reference problems and that for scalar optimization ones. Based on such relations, we have studied sufficient conditions of these well-posedness properties for the considered problems via the corresponding scalar problems. Finally, by virtue of a forcing function, the characterizations of the two concepts of well-posedness for such problems are presented.

Similar content being viewed by others
References
Adida, E., & Perakis, G. (2010). Dynamic pricing and inventory control: robust versus stochastic uncertainty models-a computational study. Ann. Oper. Res., 181, 125–157.
Anh, L. Q., & Duy, T. Q. (2016). Tykhonov well-posedness for lexicographic equilibrium problems. Optimization, 65, 1929–1948.
Ansari, Q. H., & Yao, J. C. (2012). Recent developments in vector optimization. Berlin: Springer.
Bai, Y., Migórski, S., & Zeng, S. (2019). Well-posedness of a class of generalized mixed hemivariational-variational inequalities. Nonlinear Anal Real World Appl, 48, 424–444.
Beck, A., & Ben-Tal, A. (2009). Duality in robust optimization primal worst equals dual best. Oper Res Lett, 37, 1–6.
Ben-Tal, A., El Ghaoui, L., & Nemirovski, A. (2009). Robust optimization. Princeton, US: Princeton University Press.
Bianchi, M., Kassay, G., & Pini, R. (2010). Well-posed equilibrium problems. Nonlinear Anal Theory Methods Appl, 72, 460–468.
Birge, J. R., & Louveaux, F. (2011). Introduction to stochastic programming. Berlin: Springer.
Caprari, E., Baiardi, L. C., & Molho, E. (2019). Primal worst and dual best in robust vector optimization. Eur. J. Oper. Res., 275, 830–838.
Crespi, G., Kuroiwa, D., & Rocca, M. (2014). Convexity and global well-posedness in set-optimization. Taiwan. J. Math., 18, 1897–1908.
Crespi, G. P., Kuroiwa, D., & Rocca, M. (2017). Quasiconvexity of set-valued maps assures well-posedness of robust vector optimization. Ann. Oper. Res., 251, 89–104.
Crespi, G. P., Papalia, M., & Rocca, M. (2009). Extended well-posedness of quasiconvex vector optimization problems. J. Optim. Theory Appl., 141, 285–297.
Crespi, G. P., Papalia, M., & Rocca, M. (2011). Extended well-posedness of vector optimization problems: The convex case. Taiwan. J. Math., 15, 1545–1559.
Darabi, M., & Zafarani, J. (2015). Tykhonov well-posedness for quasi-equilibrium problems. J. Optim. Theory Appl., 165, 458–479.
Dhingra, M., & Lalitha, C. (2016). Well-setness and scalarization in set optimization. Optim. Lett., 10, 1657–1667.
Dontchev, A. L., & Zolezzi, T. (1993). Well-posed optimization problems. Berlin: Springer.
Doolittle, E. K., Kerivin, H. L., & Wiecek, M. M. (2018). Robust multiobjective optimization with application to internet routing. Ann. Oper. Res., 271, 487–525.
Ehrgott, M. (2005). Multicriteria optimization. Berlin: Springer.
Ehrgott, M., Ide, J., & Schöbel, A. (2014). Minmax robustness for multi-objective optimization problems. Eur. J. Oper. Res., 239, 17–31.
Gutiérrez, C., Miglierina, E., Molho, E., & Novo, V. (2012). Pointwise well-posedness in set optimization with cone proper sets. Nonlinear Anal. Theory Methods Appl., 75, 1822–1833.
Hernández, E., & Rodríguez-Marín, L. (2007). Nonconvex scalarization in set optimization with set-valued maps. J. Math. Anal. Appl., 325, 1–18.
Ide, J., & Köbis, E. (2014). Concepts of efficiency for uncertain multi-objective optimization problems based on set order relations. Math. Methods Oper. Res., 80, 99–127.
Ide, J., Köbis, E., Kuroiwa, D., Schöbel, A., & Tammer, C. (2014). The relationship between multi-objective robustness concepts and set-valued optimization. Fixed Point Theory Appl., 2014(83), 1–20.
Ide, J., & Schöbel, A. (2016). Robustness for uncertain multi-objective optimization: a survey and analysis of different concepts. OR Spect., 38, 235–271.
Jahn, J. (2011). Vector optimization: theory, applications, and extensions. Berlin: Springer.
Jiao, L., & Lee, J. H. (2019). Finding efficient solutions in robust multiple objective optimization with sos-convex polynomial data. Ann. Oper. Res.,. https://doi.org/10.1007/s10479-019-03216-z.
Khan, A. A., Tammer, C., & Zălinescu, C. (2016). Set-valued optimization. Berlin: Springer.
Khoshkhabar-amiranloo, S., & Khorram, E. (2015). Pointwise well-posedness and scalarization in set optimization. Math. Methods Oper. Res., 82, 195–210.
Klamroth, K., Köbis, E., Schöbel, A., & Tammer, C. (2013). A unified approach for different concepts of robustness and stochastic programming via non-linear scalarizing functionals. Optimization, 62, 649–671.
Kuroiwa, D. (1998). The natural criteria in set-valued optimization. RIMS Kokyuroku Kyoto Univ, 1031, 85–90.
Kuroiwa, D. (2015). Generalized minimality in set optimization. In A. H. Hamel, F. Heyde, A. Löhne, B. Rudloff, & C. Schrage (Eds.), Set optimization and applications: the state of the art (pp. 293–311). Berlin: Springer.
Lalitha, C. S., & Bhatia, G. (2012). Levitin-Polyak well-posedness for parametric quasivariational inequality problem of the Minty type. Positivity, 16, 527–541.
Lee, J. H., & Lee, G. M. (2018). On optimality conditions and duality theorems for robust semi-infinite multiobjective optimization problems. Ann. Oper. Res., 269, 419–438.
Miglierina, E., Molho, E., & Rocca, M. (2005). Well-posedness and scalarization in vector optimization. J. Optim. Theory Appl., 126, 391–409.
Morgan, J., & Scalzo, V. (2006). Discontinuous but well-posed optimization problems. SIAM J. Optim., 17, 861–870.
Popescu, I. (2007). Robust mean-covariance solutions for stochastic optimization. Oper. Res., 55, 98–112.
Salahi, M., Torabi, N., & Amiri, A. (2016). An optimistic robust optimization approach to common set of weights in DEA. Measurement, 93, 67–73.
Tanaka, T. (1997). Generalized semicontinuity and existence theorems for cone saddle points. Appl. Math. Optim., 36, 313–322.
Tykhonov, A. N. (1966). On the stability of the functional optimization problem. USSR Comput. Math. Math. Phys., 6, 28–33.
Vui, P. T., Anh, L. Q., & Wangkeeree, R. (2019). Levitin-Polyak well-posedness for set optimization problems involving set order relations. Positivity, 23, 599–616.
Zhang, W., Li, S., & Teo, K. L. (2009). Well-posedness for set optimization problems. Nonlinear Anal. Theory Methods Appl., 71, 3769–3778.
Acknowledgements
The authors wish to thank the Editors and anonymous Referees for their helpful remarks and suggestions that helped us significantly improve the paper. This research is funded by Vietnam National Foundation for Science and Technology Development (NAFOSTED) under Grant Number 101.01-2020.11.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Anh, L.Q., Duy, T.Q. & Hien, D.V. Well-posedness for the optimistic counterpart of uncertain vector optimization problems. Ann Oper Res 295, 517–533 (2020). https://doi.org/10.1007/s10479-020-03840-0
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-020-03840-0