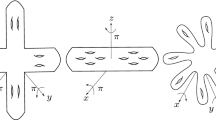
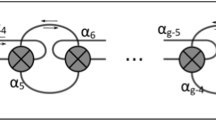
Abstract
Let M be an orientable surface with punctures and/or boundary components. Paris and Rolfsen (J Reine Angew Math 521:47–83, 2000) studied “geometric subgroups” of the mapping class group of M, that is subgroups corresponding to inclusions of connected subsurfaces. In the present paper we extend this analysis to disconnected subsurfaces and to the nonorientable case. We characterise the subsurfaces which lead to virtually abelian geometric subgroups. We provide algebraic and geometric conditions under which two geometric subgroups are commensurable. We also describe the commensurator of a geometric subgroup in terms of the stabiliser of the underlying subsurface. Finally, following the work of Paris (Math Ann 322:301–315, 2002), we show some applications of our analysis to the theory of irreducible unitary representations of mapping class groups.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Birman J.S.: Mapping class groups and their relationship to braid group. Comm. Pure Appl. Math. 22, 213–238 (1969)
Burger M., de la Harpe P.: Constructing irreducible representations of discrete groups. Proc. Indian Acad. Sci. 107, 223–235 (1997)
Chillingworth D.R.J.: A finite set of generators for the homeotopy group of a non-orientable surface. Math. Proc. Cambridge Philos. Soc. 65, 409–430 (1969)
Epstein D.B.A.: Curves on 2-manifolds and isotopies. Acta. Math. 115, 83–107 (1966)
Korkmaz M.: First homology group of mapping class groups of nonorientable surfaces. Math. Proc. Cambridge Philos. Soc. 123(3), 487–499 (1998)
Korkmaz M.: Mapping class groups of nonorientable surfaces. Geom. Dedicata 89, 109–133 (2002)
Labruère C., Paris L.: Presentations for the puctured mapping class groups in terms of Artin groups. Algebr. Geom. Topol. 1, 73–114 (2001)
Lickorish W.B.R.: A representation of orientable combinatorial 3-manifolds. Ann. Math. 76, 531–540 (1962)
Lickorish W.B.R.: Homeomorphisms of non-orientable two-manifolds. Math. Proc. Cambridge Philos. Soc. 59, 307–317 (1963)
Lickorish W.B.R.: A finite set of generators for the homeotopy group of a 2-manifold. Math. Proc. Cambridge Philos. Soc. 60, 769–778 (1964)
Mackey G.W.: The theory of unitary group representations. Chicago Lectures in Mathematics. The Uni- versity of Chicago Press, Chicago (1976)
Paris L.: Actions and irreducible representations of the mapping class group. Math. Ann. 322, 301–315 (2002)
Paris L., Rolfsen D.: Geometric subgroups of mapping class groups. J. Reine. Angew Math. 521, 47–83 (2000)
Spanier E.H.: Algebraic topology. McGraw-Hill Book Co., New York (1966)
Stukow, M.: Generating mapping class groups of nonorientable surfaces with boundary. arXiv:0707. 3497v1 (2007)
Stukow M.: Dehn twists on nonorientable surfaces. Fund. Math. 189, 117–147 (2006)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Stukow, M. Commensurability of geometric subgroups of mapping class groups. Geom Dedicata 143, 117–142 (2009). https://doi.org/10.1007/s10711-009-9377-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10711-009-9377-y