Gravitational lensing and frame dragging of light in the Kerr–Newman and the Kerr–Newman (anti) de Sitter black hole spacetimes

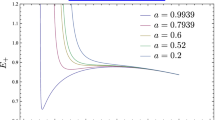
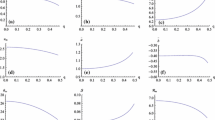
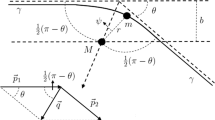
Abstract

The null geodesics that describe photon orbits in the spacetime of a rotating electrically charged black hole (Kerr–Newman) are solved exactly including the contribution from the cosmological constant. We derive elegant closed form solutions for relativistic observables such as the deflection angle and frame dragging effect that a light ray experiences in the gravitational fields (i) of a Kerr–Newman black hole and (ii) of a Kerr–Newman–de Sitter black hole. We then solve the more involved problem of treating a Kerr–Newman black hole as a gravitational lens, i.e. a KN black hole along with a static source of light and a static observer both located far away but otherwise at arbitrary positions in space. For this model, we derive the analytic solutions of the lens equations in terms of Appell and Lauricella hypergeometric functions and the Weierstraßmodular form. The exact solutions derived for null, spherical polar and non-polar orbits, are applied for the calculation of frame dragging for the orbit of a photon around the galactic centre, assuming that the latter is a Kerr–Newman black hole. We also apply the exact solution for the deflection angle of an equatorial light ray in the gravitational field of a Kerr–Newman black hole for the calculation of bending of light from the gravitational field of the galactic centre for various values of the Kerr parameter, electric charge and impact factor. In addition, we derive expressions for the Maxwell tensor components for a zero-angular-momentum-observer (ZAMO) in the Kerr–Newman–de Sitter spacetime.

Appendices

Appendix 1: The roots of the quartic in terms of Weierstraßfunctions

We are going to use the addition theorem for the Weierstraßelliptic function to express the roots of the quartic $P_{4}(x)=x^{4}+ax^{2}+bx+c\in \mathbb {C} [x]$ in terms of the Weierstraßfunctions following [49]. We first write $x$ for a point of the cover $\mathbb {C}$ and $\mathfrak {p}=(\mathbf{x},\mathbf{y})$ for the corresponding point of the cubic determined by $\mathbf{x}=\wp (x)$ and $\mathbf{y} ={\wp }^{\prime }(x)$. Then we study the intersections of the cubic $\mathbf{y}^{2}=4\mathbf{x}^{3}-g_{2}\mathbf{x}-g_{3}$ and the line $\mathbf{y}=a\mathbf{x}+b:\, \mathbf{x}_{1},\mathbf{x}_{2},\mathbf{x}_{3}$ are the roots of

$$\begin{aligned} F(\mathbf{x})&=4 \mathbf{x}^{3}-g_{2}\mathbf{x}-g_{3}-(a\mathbf{x}+b)^{2}\nonumber \\&=4(\mathbf{x}-\mathbf{x}_{1})(\mathbf{x}-\mathbf{x}_{2})(\mathbf{x}-\mathbf{x}_{3}) \end{aligned}$$

(138)

so

$$\begin{aligned} 4(\mathbf{x}_{1}-\mathbf{x}_{2})(\mathbf{x}_{1}-\mathbf{x}_{3})&= F^{\prime }(\mathbf{x}_{1})=12\mathbf{x}_{1}^{2}-g_{2}-2a(a\mathbf{x}_{1}+b)\nonumber \\&=12\mathbf{x}_{1}^{2}-g_{2}-2a\mathbf{y}_{1}. \end{aligned}$$

(139)

and

$$\begin{aligned} (\mathbf{x}_{2}-\mathbf{x}_{1})+(\mathbf{x}_{3}-\mathbf{x}_{1})=\mathbf{x}_{1}+\mathbf{x}_{2}+\mathbf{x}_{3}-3\mathbf{x}_{1}=\frac{a^{2}}{4}-3\mathbf{x}_{1}. \end{aligned}$$

(140)

We note that $a$ is the slope of the line, so for distinct $\mathbf{x}_{1},\mathbf{x}_{2},\mathbf{x}_{3}$ we have

$$\begin{aligned} a=\frac{\mathbf{y}_{2}-\mathbf{y}_{1}}{\mathbf{x}_{2}-\mathbf{x}_{1}}=\frac{\mathbf{y}_{3}-\mathbf{y}_{2}}{\mathbf{x}_{3}-\mathbf{x}_{2}}=\frac{\mathbf{y}_{1}-\mathbf{y}_{3}}{\mathbf{x}_{1}-\mathbf{x}_{3}}. \end{aligned}$$

(141)

Now

$$\begin{aligned}&(140)^{2}-(139)\nonumber \\&=(\mathbf{x}_{2}+\mathbf{x}_{3}-2\mathbf{x}_{1})^{2}-4\mathbf{x}_{1}^{2}+4\mathbf{x}_{1}\mathbf{x}_{3}+4\mathbf{x}_{1}\mathbf{x}_{2}-4\mathbf{x}_{2}\mathbf{x}_{3}\nonumber \\&=(\mathbf{x}_{2}-\mathbf{x}_{3})^{2}=\left( \frac{a^{2}}{4}-3\mathbf{x}_{1}\right) ^{2}-12 \mathbf{x}_{1}^{2}+g_{2}+2a\mathbf{y}_{1}\nonumber \\&=\left( \frac{a}{2}\right) ^{4}-6\mathbf{x}_{1}\left( \frac{a}{2}\right) ^{2}+4\mathbf{y}_{1}\left( \frac{a}{2}\right) -3\mathbf{x}_{1}^{2}+g_{2}. \end{aligned}$$

(142)

Thus

$$\begin{aligned} X&= \frac{a}{2}=\frac{1}{2}\frac{\mathbf{y}_{2}-\mathbf{y}_{1}}{\mathbf{x}_{2}-\mathbf{x}_{1}}=\frac{1}{2}\frac{\wp ^{\prime }(x_{2})-\wp ^{\prime }(x_{1})}{\wp (x_{2} )-\wp (x_{1})}, \end{aligned}$$

(143)

$$\begin{aligned} Y&= \mathbf{x}_{3}-\mathbf{x}_{2}=\wp (-x_1-x_2)-\wp (x_2)=\wp (x_{1}+x_{2})-\wp (x_{2})\Rightarrow \end{aligned}$$

(144)

$$\begin{aligned} Y^{2}&= X^{4}-6\wp (x_{1})X^{2}+4\wp ^{\prime }(x_{1})X-3\wp ^{2}(x_{1})+g_{2}\equiv P_{4}(X). \end{aligned}$$

(145)

In the second equality of (145) we used the addition theorem $x_1+x_2=-x_3$ in $\mathbb {C}/\mathbb {L}$, $\mathbb {L}$ the period lattice, and the fact that the Weierstraßfunction is even. For fixed $x_{1},$ and variable $x_{2},$ $P_{4}(X)=0$ only if $Y=0$. This occurs for $x_{2}=-\frac{x_{1}}{2}$ to which may be added one of the three half-periods producing four roots of $P_{4}(x)=0$ and these must be distinct, see Eqs. (86)–(89).

Appendix 2: Lauricella’s multivariable hypergeometric function $F_D$

In this Appendix 2, we define Lauricella’s 4th hypergeometric function of $m$-variables and its integral representation:

$$\begin{aligned} \boxed {\displaystyle F_D(\alpha ,\varvec{\beta },\gamma ,\mathbf{z})= \sum _{n_1,n_2,\ldots ,n_m=0}^{\infty }\frac{(\alpha )_{n_1+\cdots n_m}(\beta _1)_{n_1} \cdots (\beta _m)_{n_m}}{(\gamma )_{n_1+\cdots +n_m}(1)_{n_1}\cdots (1)_{n_m}} z_1^{n_1}\cdots z_m^{n_m}} \end{aligned}$$

(146)

where

$$\begin{aligned} \mathbf {z}&= (z_{1},\ldots ,z_{m}), \nonumber \\ \varvec{\beta }&= (\beta _{1},\ldots ,\beta _{m}). \end{aligned}$$

(147)

The Pochhammer symbol $\boxed {\displaystyle (\alpha )_m=(\alpha ,m)}$ is defined by

$$\begin{aligned} (\alpha )_{m}=\frac{{\varGamma } (\alpha +m)}{{\varGamma } (\alpha )}=\left\{ \begin{array}{ccc} 1, &{} \mathrm{if} &{} m=0 \\ \alpha (\alpha +1)\cdots (\alpha +m-1) &{} \mathrm{if} &{} m=1,2,3 \end{array} \right. \end{aligned}$$

(148)

With the notations $\mathbf {z}^\mathbf{n}:=z_1^{n_1}\cdots z_m^{n_m},(\varvec{\beta })_{\mathbf {n}}:=(\beta _1)_{n_1}\cdots (\beta _m)_{n_m}, \mathbf {n!}=n_1!\cdots n_m!, |\mathbf {n}|:=~n_1+\cdots +n_m$ for $m$-tuples of numbers in (147) and of non-negative integers $\mathbf {n}=(n_1,\ldots n_m)$ the Lauricella series $F_D$ in compact form is

$$\begin{aligned} F_D(\alpha ,\varvec{\beta },\gamma ,\mathbf{z}):=\sum _{\mathbf {n}}\frac{ (\alpha )_{|\mathbf {n}|}(\varvec{\beta })_{\mathbf {n}}}{(\gamma )_{|\mathbf {n}|}\mathbf {n!}}\mathbf {z}^\mathbf{n} \end{aligned}$$

(149)

The series admits the following integral representation:

$$\begin{aligned} \boxed {\displaystyle F_D(\alpha ,\varvec{\beta },\gamma ,\mathbf{z})= \frac{{\varGamma }(\gamma )}{{\varGamma }(\alpha ){\varGamma }(\gamma -\alpha )} \int _0^1 t^{\alpha -1}(1-t)^{\gamma -\alpha -1}(1-z_1 t)^{-\beta _1}\cdots (1-z_m t)^{-\beta _m} \mathrm{d}t} \end{aligned}$$

(150)

which is valid for $\boxed {\displaystyle \mathrm{Re}(\alpha )>0,\;\mathrm{Re}(\gamma -\alpha )>0.}$. It converges absolutely inside the m-dimensional cuboid:

$$\begin{aligned} |z_{j}|<1,(j=1,\ldots ,m). \end{aligned}$$

(151)

For $m=2\,F_D$ in the notation of Appell becomes the two variable hypergeometric function $F_1(\alpha ,\beta ,\beta ^{\prime },\gamma ,x,y)$ with integral representation:

$$\begin{aligned} \int _0^1 \!u^{\alpha -1}(1-u)^{\gamma -\alpha -1}(1-u x)^{-\beta }(1-u y)^{-\beta ^{\prime }}\mathrm{d}u\!=\!\frac{{\varGamma }(\alpha ){\varGamma }(\gamma \!-\!\alpha )}{{\varGamma }(\gamma )} F_1(\alpha ,\beta ,\beta ^{\prime },\gamma ,x,y) \end{aligned}$$

(152)

Appendix 3: Calculation of the Maxwell tensor components in the ZAMO frame for the KNdS spacetime

The ZAMO basis vectors are determined by the transformation

$$\begin{aligned}&\hat{e}_0=^|g_{tt}-\Omega ^2 g_{\phi \phi }|^{-1/2}\frac{\partial }{\partial t}+\Omega |g_{tt}-\Omega ^2 g_{\phi \phi }|^{-1/2}\frac{\partial }{\partial \phi }, \end{aligned}$$

(153)

$$\begin{aligned}&\hat{e}_{\phi }=\frac{1}{\sqrt{g_{\phi \phi }}}\frac{\partial }{\partial \phi }, \end{aligned}$$

(154)

$$\begin{aligned}&\hat{e}_r=\left( \frac{{\varDelta }_r^{KN}}{\rho ^2}\right) ^{1/2}\frac{\partial }{\partial r}, \end{aligned}$$

(155)

$$\begin{aligned}&\hat{e}_{\theta }=\left( \frac{{\varDelta }_{\theta }}{\rho ^2}\right) ^{1/2}\frac{\partial }{\partial \theta }, \end{aligned}$$

(156)

where the angular velocity $\Omega$ is given in the KNdS case by the expression:

$$\begin{aligned} \Omega =\frac{-ac \sin ^2\theta [{\varDelta }^{KN}_r-{\varDelta }_{\theta }(r^2+a^2)]}{{\varXi }^2 \rho ^2 g_{\phi \phi }}=\frac{-ac [{\varDelta }^{KN}_r-{\varDelta }_{\theta }(r^2+a^2)]}{({\varDelta }_{\theta }(r^2+a^2)^2-a^2\sin ^2\theta {\varDelta }_{r}^{KN})}\nonumber \\ \end{aligned}$$

(157)

and the quantity $g_{tt}g_{\phi \phi }-g^2_{\phi t}=\frac{-c^2 {\varDelta }_r^{KN}{\varDelta }_{\theta }\sin ^2\theta }{{\varXi }^4}$. In addition, we compute for the lapse function $\alpha _{ZAMO}$:

$$\begin{aligned} \boxed {\displaystyle \alpha _{ZAMO}:=|g_{tt}-\Omega ^2 g_{\phi \phi }|^{1/2}=\frac{c({\varDelta }_r^{KN})^{1/2}{\varDelta }_{\theta }^{1/2}\sin \theta }{{\varXi }^2 \sqrt{g_{\phi \phi }}}} \end{aligned}$$

(158)

Equation (158), reduces correctly, assuming ${\varLambda }=0$, to the lapse function derived in [50].

Now our analytic calculation for the electric ($\varvec{E}$) and magnetic fields ($\varvec{B}$) in the presence of the cosmological constant ${\varLambda }$ in the ZAMO frame yields:

$$\begin{aligned}&\boxed {\displaystyle E^{r}=\frac{(r^2+a^2)e[-r^2+a^2 \cos ^2\theta ]{\varDelta }_{\theta }^{1/2}}{\sqrt{(r^2+a^2)^2 {\varDelta }_{\theta }-a^2\sin ^2\theta {\varDelta }_r^{KN}}\rho ^4},} \end{aligned}$$

(159)

$$\begin{aligned}&\boxed { \displaystyle E^{\theta }=\frac{-2era^2 ({\varDelta }_r^{KN})^{1/2}\cos \theta \sin \theta }{\rho ^4 \sqrt{(r^2+a^2)^2 {\varDelta }_{\theta }-a^2\sin ^2\theta {\varDelta }_r^{KN}}},} \end{aligned}$$

(160)

$$\begin{aligned}&\boxed {\displaystyle B^r=\frac{2era (r^2+a^2)\cos \theta {\varDelta }_{\theta }^{1/2}}{\rho ^4 \sqrt{(r^2+a^2)^2 {\varDelta }_{\theta }-a^2\sin ^2\theta {\varDelta }_r^{KN}}},} \end{aligned}$$

(161)

$$\begin{aligned}&\boxed { \displaystyle B^{\theta }=\frac{-({\varDelta }_r^{KN})^{1/2}a\sin \theta e[-r^2+a^2\cos ^2\theta ]}{\rho ^4 \sqrt{(r^2+a^2)^2 {\varDelta }_{\theta }-a^2\sin ^2\theta {\varDelta }_r^{KN}}}.} \end{aligned}$$

(162)

Also it holds:

$$\begin{aligned} E^{\phi }=B^{\phi }=0 \end{aligned}$$

(163)

To the best of our knowledge Eqs. (159)–(162) represent the first calculation of the Maxwell tensor components in the ZAMO frame for the case of the Kerr–Newman–de Sitter spacetime. Our solutions for the electric and magnetic fields, for zero cosmological constant, reduce correctly to the corresponding expressions for the Kerr–Newman spacetime and LNRF observers derived in [51].