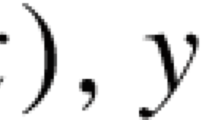Skip to main content

Advertisement

SpringerLink
Log in

An Interior Proximal Method for a Class of Quasimonotone Variational Inequalities

  • Published:
Journal of Optimization Theory and Applications Aims and scope Submit manuscript

Abstract

The Bregman-function-based Proximal Point Algorithm for variational inequalities is studied. Classical papers on this method deal with the assumption that the operator of the variational inequality is monotone. Motivated by the fact that this assumption can be considered to be restrictive, e.g., in the discussion of Nash equilibrium problems, the main objective of the present paper is to provide a convergence analysis only using a weaker assumption called quasimonotonicity. To the best of our knowledge, this is the first algorithm established for this general and frequently studied class of problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Algorithm 1

Similar content being viewed by others

Notes

  1. That is, \(\mathcal {T}\) is the (sub)differential of a quasiconvex function (whose required analytical properties are tacitly assumed to hold here).

  2. Single-valued operators \(\mathcal {T}: \mathbb {R}^{n} \to \mathbb {R}^{n}\) are included in the canonical way.

  3. Although a large class of linearly and nonlinearly constrained sets admits the existence of such a function, it is worthy of mention that there are still some sets K for which the existence of a zone-coercive Bregman-like function (with zone \(\operatorname{int} K\)) is still unknown (see [2, Example 3] and [23] in this regard).

  4. There are some more examples in which this property holds, but the case of strongly convex h is enough for the present paper.

  5. The latter assumption might be considered as a critical one; some related comments are postponed to the discussion of all assumptions made in the convergence analysis below.

  6. For example, in case \(\mathcal {T}\) is single-valued, we might think of Newton-type methods, etc.

  7. Of course, if relative errors are left out by letting σ=0, we obtain y k=x k+1, and thus no such extragradient-like step is necessary.

  8. This will be concretized below, but for instance, it is sufficient that they tend to zero.

  9. Of course, a suitable parameter choice, regularity conditions, etc. are also necessary, but this falls out of the scope of the present discussion.

  10. It is rather easy to see that this property is not necessary if ∇h is Lipschitz continuous (as is the case, e.g., within classical proximal point methods). As Lipschitz continuity of ∇h contradicts the zone coerciveness of h, one might consider this assumption as the price to pay for unconstrained subproblems.

  11. For the sake of simplicity, we leave out technical details here and reduce ourselves to a very pregnant formulation. This also includes that the exact version of the BPPA is dealt with, i.e., there is only one generated sequence that may converge or not.

  12. Anyway, it should be noted that this construction describes the only known Bregman-like functions with a nonlinearly constrained zone.

  13. In fact, this makes the assumption of the existence of nonzero solutions superfluous.

References

  1. Burachik, R., Iusem, A.: A generalized Proximal Point Algorithm for the variational inequality problem in a Hilbert space. SIAM J. Optim. 8, 197–216 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  2. Langenberg, N.: Pseudomonotone operators and the Bregman Proximal Point Algorithm. J. Glob. Optim. 47, 537–555 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  3. Langenberg, N., Tichatschke, R.: Interior proximal methods for quasiconvex optimization. J. Glob. Optim. 52, 641–661 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  4. Aussel, D., Corvellec, J.N., Lassonde, M.: Subdifferential characterization of quasiconvexity and convexity. J. Convex Anal. 1, 195–201 (1994)

    MathSciNet  MATH  Google Scholar 

  5. Hadjisavvas, N., Schaible, S.: Quasimonotone variational inequalities in Banach spaces. J. Optim. Theory Appl. 90, 95–111 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  6. Aussel, D., Hadjisavvas, N.: Technical note: on quasimonotone variational inequalities. J. Optim. Theory Appl. 121, 445–450 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  7. Konnov, I.: On quasimonotone variational inequalities. J. Optim. Theory Appl. 99, 165–181 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  8. Karamardian, S., Schaible, S.: Seven kinds of monotone maps. J. Optim. Theory Appl. 66, 37–46 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  9. Karamardian, S., Schaible, S., Crouzeix, J.P.: Characterizations of generalized monotone maps. J. Optim. Theory Appl. 76, 399–413 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  10. Crouzeix, J.P., Ferland, J.: Criteria for differentiable generalized monotone maps. Math. Program. 75, 399–406 (1996)

    MathSciNet  MATH  Google Scholar 

  11. Hadjisavvas, N., Komlosi, S., Schaible, S. (eds.): Handbook of Generalized Convexity and Generalized Monotonicity. Nonconvex Optimization and Its Applications, vol. 76. Springer, Boston (2005)

    MATH  Google Scholar 

  12. Polyak, B.T.: Introduction to Optimization. Optimization Software, New York (1987)

    Google Scholar 

  13. Facchinei, F., Pang, J.S.: Finite-Dimensional Variational Inequalities and Complementarity Problems. Springer, New York (2003)

    Google Scholar 

  14. Crouzeix, J.P., Eberhard, A., Ralph, D.: A geometrical insight on pseudoconvexity and pseudomonotonicity. Math. Program. 123, 61–83 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  15. Karamardian, S.: Complementarity problems over cones with monotone and pseudomonotone maps. J. Optim. Theory Appl. 18, 445–454 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  16. Rockafellar, R., Wets, R.: Variational Analysis. Springer, Berlin (1997)

    Google Scholar 

  17. Bauschke, H., Borwein, J.: Legendre functions and the method of random Bregman projections. J. Convex Anal. 4, 27–67 (1997)

    MathSciNet  MATH  Google Scholar 

  18. Bregman, L.: The relaxation method of finding the common points of convex sets and its application to the solution of problems in convex programming. USSR Comput. Math. Math. Phys. 7, 200–217 (1967)

    Article  Google Scholar 

  19. Censor, Y., Zenios, S.: Proximal minimization algorithm with D-functions. J. Optim. Theory Appl. 73, 451–464 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  20. Chen, G., Teboulle, M.: Convergence analysis of a proximal-like minimization algorithm using Bregman functions. SIAM J. Optim. 3, 538–543 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  21. Eckstein, J.: Nonlinear proximal point algorithms using Bregman functions, with application to convex programming. Math. Oper. Res. 18, 202–226 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  22. Censor, Y., Iusem, A., Zenios, S.: An interior point method with Bregman functions for the variational inequality problem with paramonotone operators. Math. Program. 81, 373–400 (1998)

    MathSciNet  MATH  Google Scholar 

  23. Kaplan, A., Tichatschke, R.: Note on the paper: interior proximal method for variational inequalities on non-polyhedral sets. Discuss. Math., Differ. Incl. Control Optim. 30, 51–59 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  24. Solodov, M., Svaiter, B.: An inexact hybrid generalized Proximal Point Algorithm and some new results on the theory of Bregman functions. Math. Oper. Res. 25, 214–230 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  25. Kaplan, A., Tichatschke, R.: On inexact generalized proximal methods with a weakened error tolerance criterion. Optimization 53, 3–17 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  26. Eckstein, J.: Approximate iterations in Bregman-function-based proximal algorithms. Math. Program. 83, 113–123 (1998)

    MathSciNet  MATH  Google Scholar 

  27. Han, D.: A new hybrid generalized Proximal Point Algorithm for variational inequality problems. J. Glob. Optim. 26, 125–140 (2003)

    Article  MATH  Google Scholar 

  28. Langenberg, N.: Convergence Analysis of Proximal-Like Methods for Variational Inequalities and Fixed Point Problems Applications to the Nash Equilibrium Problem. Logos, Berlin (2011)

    Google Scholar 

  29. Langenberg, N.: Convergence analysis of an extended Auxiliary Problem Principle with various stopping criteria. Optim. Methods Softw. 26, 127–154 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  30. Langenberg, N.: On the cutting plane property and the Bregman Proximal Point Algorithm. J. Convex Anal. 18, 601–619 (2011)

    MathSciNet  MATH  Google Scholar 

  31. Crouzeix, J.P., Marcotte, P., Zhu, D.: Conditions ensuring the applicability of cutting plane methods for solving variational inequalities. Math. Program. 88, 521–539 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  32. Castellani, M., Giuli, M.: A characterization of the solution set of pseudoconvex extremum problems. J. Convex Anal. 19 (2013)

  33. Kaplan, A., Tichatschke, R.: Interior proximal method for variational inequalities: case of non-paramonotone operators. Set-Valued Anal. 12, 357–382 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  34. Burachik, R., Dutta, J.: Inexact proximal point methods for variational inequality problems. SIAM J. Optim. 20, 2653–2678 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

I am grateful to an anonymous referee whose comments greatly improved the present paper. Moreover, my thanks also go to the associate editor and the editor-in-chief for further very helpful remarks.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Trier, 54286, Trier, Germany

    Nils Langenberg

Authors
  1. Nils Langenberg
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nils Langenberg.

Additional information

Communicated by Nicolas Hadjisavvas.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Langenberg, N. An Interior Proximal Method for a Class of Quasimonotone Variational Inequalities. J Optim Theory Appl 155, 902–922 (2012). https://doi.org/10.1007/s10957-012-0111-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10957-012-0111-9

Keywords

Search

Navigation