Strain-gradient crystal-plasticity modelling of micro-cutting of b.c.c. single crystal

Abstract

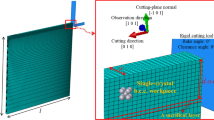
In recent years thanks to enhancements in design of advanced machines, laser metrology and computer control, ultra-precision machining has become increasingly important. In micromachining of metals the depth of cut is usually less than the average grain size of a polycrystalline aggregate; hence, a cutting process can occur entirely within a single crystal. The respective effect of crystallographic anisotropy requires development of machining models that incorporate crystal plasticity for an accurate prediction of micro-scale material removal under such conditions. To achieve this, a 3D finite-element model of orthogonal micro-cutting of a single crystal of b.c.c. brass was implemented in a commercial software ABAQUS/Explicit using a user-defined subroutine VUMAT. Strain-gradient crystal-plasticity theories were used to demonstrate the influence of evolved strain gradients on the cutting process for different cutting directions.

Appendix

An enhanced modelling scheme for a strain-gradient crystal-plasticity (EMSGCP) theory proposed by Demiral [14] was used in the simulations. In the EMSGCP theory, the critical resolved shear stress (CRSS) ($g_{T}^{\alpha } |_{t = 0}$) is governed by the initial strength of slip systems related to SSDs ($g_{S}^{\alpha } |_{t = 0}$) and GNDs ($g_{G}^{\alpha } |_{t = 0}$), linked with initial SSD ($\rho_{S} |_{t = 0}$) and GND ($\rho_{G} |_{t = 0}$) densities via the constant, K:

$$ g_{T}^{\alpha } |_{{{\text{t}} = 0}} = \sqrt {\left( {g_{S}^{\alpha } |_{{{\text{t}} = 0}} } \right)^{2} + \left( {g_{G}^{\alpha } |_{{{\text{t}} = 0}} } \right)^{2} } , $$

(1a)

$$ \begin{aligned} g_{S}^{\alpha } |_{{{\text{t}} = 0}} & = K\sqrt {\rho_{S} |_{{{\text{t}} = 0}} } , \\ g_{G}^{\alpha } |_{{{\text{t}} = 0}} & = K\sqrt {\rho_{G} |_{{{\text{t}} = 0}} } = K\sqrt {\rho |_{{{\text{t}} = 0}} \left( {\bar{S}/\bar{V}} \right)^{2} } . \\ \end{aligned} $$

(1b)

The GND density term was expressed as a function of the normalized surface-to-volume $\left( {{{\bar{S}} \mathord{\left/ {\vphantom {{\bar{S}} {\bar{V}}}} \right. \kern-0pt} {\bar{V}}}} \right)$ ratio (hence, dimensionless) for the component under study [30]. The slip resistance during loading evolves with hardening due to the SSDs ($\Delta g_{S}^{\alpha }$) and GNDs ($\Delta g_{G}^{\alpha }$) on the slip system as follows:

$$ g_{T}^{\alpha } = g_{T}^{\alpha } |_{t = 0} + \sqrt {\left( {\Delta g_{S}^{\alpha } } \right)^{2} + \left( {\Delta g_{G}^{\alpha } } \right)^{2} } , $$

(1c)

$$ \Delta {\text{g}}_{S}^{\alpha } = \sum\limits_{\beta = 1}^{\text{N}} {h_{\alpha \beta }\Delta \gamma^{\beta } ,} \;\Delta {\text{g}}_{G}^{\alpha } = \alpha \mu \sqrt {{\text{bn}}_{G}^{\alpha } } , $$

(1d)

where $h_{\alpha \beta }$, α _T , μ, b and $n_{G}^{\alpha }$ corresponds to the slip-hardening modulus, the Taylor coefficient, the shear modulus, the Burgers vector and the effective density of geometrically necessary dislocations, respectively.

The hardening model proposed by Peirce et al. [24] was used to describe $h_{\alpha \beta }$, as follows:

$$ \begin{aligned} {\text{h}}_{\alpha \alpha } & = {\text{h}}_{0} \text{sech}^{2} \left| {\frac{{{\text{h}}_{0} \tilde{\gamma }}}{{g_{T}^{\alpha } |_{\text{sat}} - g_{T}^{\alpha } |_{{{\text{t}} = 0}} }}} \right|, \\ {\text{h}}_{\alpha \beta } & = q{\text{h}}_{\alpha \alpha } (\alpha \ne \beta ), \\ \tilde{\gamma } & = \sum\limits_{\alpha } {\int\limits_{0}^{t} {\left| {\dot{\gamma }^{\alpha } } \right|dt} } , \\ \end{aligned} $$

(2)

where h ₀ is the initial hardening parameter, $g_{T}^{\alpha } |_{\text{sat}}$ is the saturation stress of the slip system α, q is the latent hardening ratio, and $\tilde{\gamma }$ is the Taylor cumulative shear strain on all slip systems. The effective GND density ($n_{G}^{\alpha }$) is given by

$$ {\text{n}}_{G}^{\alpha } = \left| {{\varvec{m}}^{\alpha } \times \sum\limits_{\beta } {s^{\alpha \beta } \nabla \gamma^{\beta } } \times {\varvec{m}}^{\beta } } \right|, $$

(3)

where $\varvec{s}^{\alpha }$ is the slip direction, $\varvec{m}^{\alpha }$ is the slip-plane normal, $s^{\alpha \beta } = \varvec{s}^{\alpha } .\varvec{s}^{\beta }$ and ∇γ ^β is the gradient of shear strain in each slip system [24].

In Eq. (2), $\dot{\gamma }^{\alpha }$ is the shearing rate on the slip system α expressed by the following power-law equation:

$$ \dot{\gamma }^{\alpha } = \dot{\gamma }_{0}^{\alpha } \text{sgn} (\tau^{\alpha } )\left| {\frac{{\tau^{\alpha } }}{{g_{T}^{\alpha } }}} \right|^{n} , $$

(4)

where $\dot{\gamma }_{0}^{\alpha }$, n, τ ^α and $g_{T}^{\alpha }$ are the reference strain rate, the macroscopic rate-sensitivity parameter, the resolved shear stress, the strength of the slip system α at the current time, respectively, and $\text{sgn} ( * )$ is the signum function of *. In an enhanced model of crystal-plasticity (EMCP) $\Delta g_{G}^{\alpha }$ is assumed to be equal to 0.