Skip to main content
SpringerLink
Log in

In-plane bending vibration analysis of a rotating beam with multiple edge cracks by using the transfer matrix method

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

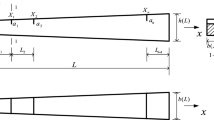
In this study, the effects of cracks on the natural frequencies of a rotating Bernoulli–Euler beam are investigated using a new numerical method in which these effects can be computed simply using the transfer matrix method. The present method using the crack model of a rotating beam developed by considering the distributed mass can determine the desired number of accurate natural frequencies for rotating cracked beams regardless of the size and location of the crack, and this method can produce accurate results by using the minimum number of subdivisions. The Frobenius method is used to determine the roots of the relevant differential equation. An open crack is considered, and each crack is modeled as a rotational spring. With these assumptions, the additional bending displacement generated by the crack is formulated as a separate transfer matrix. The computed results are compared with those discussed of a previous work to demonstrate the accuracy of the proposed method. The effects of the crack on the natural frequencies of rotating beams at different rotational speeds and hub radii are investigated through a parametric study with respect to the size and location of the crack.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Chondros TG, Dimarogonas AD, Yao J (1998) A continuous cracked beam vibration theory. J Sound Vib 215:17–34

    Article  ADS  MATH  Google Scholar 

  2. Datta PK, Ganguli R (1990) Vibration characteristics of a rotating blade with localized damage including the effects of shear deformation and rotary inertia. Comput Struct 36:1129–1133

    Article  Google Scholar 

  3. Lee YS, Chung MJ (2000) A study on crack detection using eigenfrequency test data. Comput Struct 77:327–342

    Article  Google Scholar 

  4. Nahvi H, Jabbari M (2005) Crack detection in beams using experimental modal data and finite element model. Int J Mech Sci 47:1477–1497

    Article  Google Scholar 

  5. Papadopoulos CA, Dimarogonas AD (1987) Coupled longitudinal and bending vibrations of a rotating shaft with an open crack. J Sound Vib 117:81–93

    Article  ADS  Google Scholar 

  6. Liu C, Jiang D (2014) Crack modeling of rotating blades with cracked hexahedral finite element method. Mech Syst Sig Process 46:406–423

    Article  ADS  Google Scholar 

  7. Masoud AA, Al-Said S (2009) A new algorithm crack localization in a rotating Timoshenko beam. J Vib Control 15:1541–1561

    Article  MathSciNet  MATH  Google Scholar 

  8. Sari MS, Butcher EA (2012) Free vibration analysis of non-rotating and rotating Timoshenko beam with damaged boundary using the Chebyshev collocation method. Int J Mech Sci 60:1–11

    Article  Google Scholar 

  9. Behzad M, Ghadami A, Maghsoodi A, Hale JM (2013) Vibration based algorithm for crack detection in cantilever beam containing two different types of cracks. J Sound Vib 332:6312–6320

    Article  ADS  Google Scholar 

  10. Mazanoglu K, Sabuncu M (2010) Vibration analysis of non-uniform beams having multiple edge cracks along the beam’s height. Int J Mech Sci 52:515–522

    Article  Google Scholar 

  11. Wauer J (1990) On the dynamics of cracked rotors: a literature survey. Appl Mech Rev 43:13–17

    Article  ADS  Google Scholar 

  12. Dimogoronas AD (1996) Vibration of cracked structures: a state of the art review. Eng Fract Mech 55:831–857

    Article  Google Scholar 

  13. Kisa M, Gurel MA (2007) Free vibration analysis uniform and stepped cracked beams with circular cross sections. Int J Eng Sci 45:364–380

    Article  MATH  Google Scholar 

  14. Kheim NT, Lien TV (2004) Multi-crack detection for beam by the natural frequencies. J Sound Vib 273:175–184

    Article  ADS  Google Scholar 

  15. Argatov I, Sevostianov I (2010) Health monitoring of bolted joints via electrical conductivity measurement. Int J Eng Sci 48:874–887

    Article  Google Scholar 

  16. Loya JA, Rubio L, Fernández-Sáez J (2006) Natural frequencies for bending vibrations of Timoshenko cracked beams. J Sound Vib 290:640–653

    Article  ADS  Google Scholar 

  17. Chaudhari TD, Maiti SK (1999) Modelling of transverse vibration of beam of linearly variable depth with edge crack. Eng Fract Mech 63:425–445

    Article  Google Scholar 

  18. Donà M, Palmeri A, Lombardo M (2015) Dynamic analysis of multi-cracked Euler–Bernoulli beams with gradient elasticity. Comput Struct 161:64–76

    Article  Google Scholar 

  19. Stafford RO, Giurgiutiu V (1975) Semi-analytic methods for rotating Timoshenko beams. Int J Mech Sci 17:719–727

    Article  MATH  Google Scholar 

  20. Sarkar K, Ganguli R (2014) Analytical test functions for free vibration analysis of rotating non-homogeneous Timoshenko beams. Meccanica 49:1469–1477

    Article  MathSciNet  MATH  Google Scholar 

  21. Banerjee JR (2000) Free vibration of centrifugally stiffened uniform and tapered beams using the dynamic stiffness method. J Sound Vib 233:857–875

    Article  ADS  MATH  Google Scholar 

  22. Ozgumus OO, Kaya MO (2010) Vibration analysis of a rotating tapered Timoshenko beam using DTM. Meccanica 45(1):33–42

    Article  MATH  Google Scholar 

  23. Lee JW, Lee JY (2016) Free vibration analysis using the transfer-matrix method on a tapered beam. Comput Struct 164:75–82

    Article  Google Scholar 

  24. Bhat RB (1986) Transverse vibrations of a rotating uniform cantilever beam with tip mass as predicted by using beam characteristic orthogonal polynomials in the Rayleigh–Ritz method. J Sound Vib 105:199–210

    Article  ADS  Google Scholar 

  25. Wang G, Wereley NM (2004) Free vibration analysis of rotating blades with uniform tapers. AIAA J 42:2429–2437

    Article  ADS  Google Scholar 

  26. Attar M (2012) A transfer matrix method for free vibration analysis and crack identification of stepped beams with multiple edge cracks and different boundary conditions. Int J Mech Sci 57:19–33

    Article  Google Scholar 

  27. Banerjee JR, Su H, Jackson DR (2006) Free vibration of rotating tapered beams using the dynamic stiffness method. J Sound Vib 298:1034–1054

    Article  ADS  Google Scholar 

  28. Ozgumus OO, Kaya MO (2006) Flapwise bending vibration analysis of double tapered rotating Euler–Bernoulli beam by using the differential transform method. Meccanica 41:661–670

    Article  MATH  Google Scholar 

  29. Rezaee M, Hassannejad R (2010) Damped free vibration analysis of a beam with fatigue crack using energy balance method. Int J Phys Sci 5:793–803

    Google Scholar 

  30. Zhao X, Zhao YR, Gao XZ, Li XY, Li YH (2016) Green’s Functions for the forced vibrations of cracked Euler–Bernoulli beams. Mech Syst Signal Process 68–69:155–175

    Article  Google Scholar 

  31. Friswell MI, Penny JET (2002) Crack modelling for structural health monitoring. Struct Health Monit 1:139–148

    Article  Google Scholar 

  32. Sinha JK, Friswell MI, Edwards S (2002) Simplified models for the location of cracks in beam structures using measured vibration data. J Vib Sound 25:13–38

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical System Engineering, Kyonggi University, 154-42, Gwanggyosan-ro, Yeongtong-gu, Suwon-si, Gyeonggi-do, 16227, Republic of Korea

    Jung Woo Lee & Jung Youn Lee

Authors
  1. Jung Woo Lee
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jung Youn Lee
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jung Youn Lee.

Appendix

Appendix

1.1 Components of the C matrix of Eq. (33)

$$C_{1j} = \sum \limits_{j = 1}^{4} f\left( {0,j - 1} \right)$$
(62)
$$C_{2j} = \sum \limits_{j = 1}^{4} f^{\prime}\left( {0,j - 1} \right)$$
(63)
$$C_{3j} = - EI \sum \limits_{j = 1}^{4} f^{\prime\prime}\left( {0,j - 1} \right)$$
(64)
$$C_{4j} = EI\left( { \sum \limits_{j = 1}^{4} f^{\prime\prime\prime}\left( {0,j - 1} \right) + C_{2} \sum \limits_{j = 1}^{4} f^{\prime}\left( {0,j - 1} \right)} \right).$$
(65)

1.2 Components of the H matrix of Eq. (40)

$$H_{1j} = \sum \limits_{j = 1}^{4} f\left( {L_{k} ,j - 1} \right)$$
(66)
$$H_{2j} = \sum \limits_{j = 1}^{4} f^{\prime}\left( {L_{k} ,j - 1} \right)$$
(67)
$$H_{3j} = - EI \sum \limits_{j = 1}^{4} f^{\prime\prime}\left( {L_{k} ,j - 1} \right)$$
(68)
$$H_{4j} = EI\left( { \sum \limits_{j = 1}^{4} f^{\prime\prime\prime}\left( {L_{k} ,j - 1} \right) + \left( {0.5C_{1} L_{k}^{2} + r_{H} C_{1} L_{k} + C_{2} } \right) \sum \limits_{j = 1}^{4} f^{\prime}\left( {L_{k} ,j - 1} \right)} \right)$$
(69)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lee, J.W., Lee, J.Y. In-plane bending vibration analysis of a rotating beam with multiple edge cracks by using the transfer matrix method. Meccanica 52, 1143–1157 (2017). https://doi.org/10.1007/s11012-016-0449-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-016-0449-4

Keywords

Search

Navigation