Skip to main content
SpringerLink
Log in

Stochastic earthquake interevent time modeling from exponentiated Weibull distributions

  • Original Paper
  • Published:
Natural Hazards Aims and scope Submit manuscript

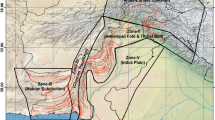
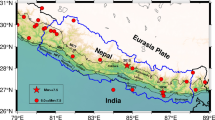
Abstract

In view of the growing importance of stochastic earthquake modeling in disaster preparation, the present study introduces a new family of exponentiated Weibull distribution and examines its performance in earthquake interevent time analysis in a stationary point process. This three-parameter (one scale and two shapes) distribution not only covers the Weibull distribution, exponentiated exponential distribution, Burr-type X distribution, Rayleigh distribution, and exponential distribution as special sub-families, but also offers monotone and non-monotone hazard shapes. Here we first describe some of the exponentiated Weibull distribution properties, such as the survival rate, mode, median, and hazard rate. We then provide statistical inference and goodness-of-fit measures to examine the suitability of exponentiated Weibull model in comparison with other popular models, like exponential, gamma, lognormal, Weibull, and exponentiated exponential. Finally, we conduct real data analysis to assess the usefulness and flexibility of exponentiated Weibull distribution in the context of seismic interevent time modeling and associated applications. Results suggest that the exponentiated Weibull distribution has a comparable performance with other popular distributions of its nature. However, further investigations are necessary to confirm the importance and flexibility of exponentiated Weibull distribution in statistical seismology.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Modified after Yadav et al. (2010) and Pasari and Dikshit (2014)

Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  • Bak P, Christensen K, Danon L, Scanlon T (2002) Unified scaling law for earthquakes. Phys Rev Lett 88(17):178501–178504

    Article  Google Scholar 

  • Bebbington M, Lai CD, Zitikis R (2007) Modeling human mortality using mixtures of bathtub shaped failure distributions. J Theor Bio 245(3):528–538

    Article  Google Scholar 

  • BIS (2002) IS 1893 (part 1)–2002: Indian standard criteria for earthquake resistant design of structures, part I—general provisions and buildings. Bureau of Indian Standards, New Delhi

    Google Scholar 

  • Bray A, Schoenberg FP (2013) Assessment of point process models in earthquake forecasting. Stat Sci 28(4):510–520

    Article  Google Scholar 

  • Chaubey YP (2007) Modeling of distribution for excess-of-loss insurance data. http://stat.unipi.gr/ime2007/papers/paper/Chaubey.pdf

  • Chen C, Wang JP, Wu YM, Chan CH (2013) A study of earthquake inter-occurrence distribution models in Taiwan. Nat Hazards 69(3):1335–1350

    Article  Google Scholar 

  • Gupta RD, Kundu D (1999) Generalized exponential distributions. Aust N Z J Stat 41(2):173–188

    Article  Google Scholar 

  • Gupta HK, Rao NP, Rastogi BK, Sarkar D (2001) The deadliest intraplate earthquake. Science 291:2101–2102

    Article  Google Scholar 

  • IMSL (1991) International Mathematical and Statistical Libraries. User’s manual. IMSL, Houston

    Google Scholar 

  • Jiang R, Murthy DNP (1999) The exponentiated family: a graphical approach. IEEE Trans Reliab 48(1):68–72

    Article  Google Scholar 

  • Johnson NL, Kotz S, Balakrishnan N (1995) Continuous univariate distributions, vol 2, 2nd edn. Wiley, New York, p 756

    Google Scholar 

  • Jordan TH (2006) Earthquake predictability, brick by brick. Seism Res Lett 77:3–6

    Article  Google Scholar 

  • Jordan TH, Jones LM (2010) Operational earthquake forecasting: some thoughts on why and how. Seism Res Lett 81:571–574

    Article  Google Scholar 

  • Kijko A, Sellevoll MA (1981) Triple exponential distribution, a modified model for the occurrence of large earthquakes. Bull Seismol Soc Am 71:2097–2101

    Google Scholar 

  • Lee YT, Turcotte DL, Holliday JR, Sachs MK, Rundle JB, Chen CC, Tiampo KF (2011) Results of the Regional Earthquake Likelihood Models (RELM) test of earthquake forecasts in California. Proc Natl Acad Sci 108(40):16533–16538

    Article  Google Scholar 

  • Lehman EL (1953) The power of rank tests. Ann Math Stat 24:28–32

    Google Scholar 

  • Leiva V, Barros M, Paula GA (2009) Generalized Birnbaum–Saunders models using R. Brazilian Statistical Association, Sao Paulo, p 329

    Google Scholar 

  • Marzochhi W, Lombardi AM (2008) A double branching model for earthquake occurrence. J Geophys Res 113:B08317. https://doi.org/10.1029/2007JB005472

    Google Scholar 

  • MATLAB version 7.10.0 (2010) The MathWorks Inc, Natick, Massachusetts, United States

  • Mudholkar GS, Huston AD (1996) The exponentiated Weibull family: some properties and a flood data application. Commun Stat Theory Methods 25:3059–3083

    Article  Google Scholar 

  • Mudholkar GS, Srivastava DK (1993) Exponentiated Weibull family for analyzing bathtub failure-rate data. IEEE Trans Reliab 42(2):299–302

    Article  Google Scholar 

  • Mudholkar GS, Srivastava DK, Freimer M (1995) The exponentiated Weibull family: a reanalysis of the bus-motor-failure data. Technometrics 37(4):436–445

    Article  Google Scholar 

  • Mulargia F, Tinti S (1985) Seismic sample area defined from incomplete catalogs: an application to the Italian territory. Phys Earth Planet Sci 40(4):273–300

    Article  Google Scholar 

  • Murthy DNP, Xie M, Jiang R (2004) Weibull models, 1st edn. Wiley, Hoboken, p 383

    Google Scholar 

  • Nadarajah S, Cordeiro GM, Ortega EMM (2012) The exponentiated Weibull distribution: a survey. Stat Pap. https://doi.org/10.1007/s00362-012-0466-x

    Google Scholar 

  • NAG–Numerical Algorithms Group (1993) Mark 16 Numerical Algorithms Group, Downers Grove, Illinos

  • Nishenko SP, Bullard R (1987) A generic recurrence interval distribution for earthquake forecasting. Bull Seismol Soc Am 77:1382–1399

    Google Scholar 

  • Ogata Y (1988) Statistical models for earthquake occurrences and residual analysis for point processes. J Am Stat As 83:9–27

    Article  Google Scholar 

  • Ogata Y (1998) Space-time point-process models for earthquake occurrences. Ann Inst Statist Math 50:379–402

    Article  Google Scholar 

  • Pal M, Ali MM, Woo J (2006) Exponentiated Weibull distribution. Statistica LXVI:139–147

    Google Scholar 

  • Pasari S, Dikshit O (2014) Impact of three-parameter Weibull models in probabilistic assessment of earthquake hazards. Pure Appl Geophys 171:1251–1281

    Article  Google Scholar 

  • Pasari S, Dikshit O (2015) Three-parameter generalized exponential distribution in earthquake recurrence interval estimation. Nat Hazards 73:639–656

    Article  Google Scholar 

  • Qian L (2012) The Fischer information matrix for a three-parameter exponentiated Weibull distribution under type-II censoring. Stat Methods 9:320–329

    Article  Google Scholar 

  • Rundle JB, Turcotte DL, Shchebakov R, Klein W, Sammis C (2003) Statistical physics approach to understanding the multiscale dynamics of earthquake fault systems. Rev Geophys 41(4):1019

    Article  Google Scholar 

  • Schorlemmer D, Gerstenberger MC, Wiemer S, Jackson DD, Rhoades DA (2007) Earthquake likelihood model testing. Seismol Res Lett 78:17–29

    Article  Google Scholar 

  • Shao Q, Wong H, Xia J, Ip WC (2004) Models for extreme using the extended three-parameter Burr XII system with application to flood frequency analysis. Hydrol Sci J 49:685–702

    Article  Google Scholar 

  • Sornette D, Knopoff L (1997) The paradox of the expected time until the next earthquake. Bull Seismol Soc Am 87:789–798

    Google Scholar 

  • Talbi A, Nanjo K, Zhuang J, Satake K, Hamadache M (2013) Interevent times in a new alarm-based earthquake forecasting model. Geophys J Int 194(3):1823–1835

    Article  Google Scholar 

  • Tse SK, Yuen HK (2006) A comparison of three families of survival distributions for quantal response data. J Biopharm Stat 16:253–264

    Article  Google Scholar 

  • Utsu T (1984) Estimation of parameters for recurrence models of earthquakes. Bull Earthq Res Inst Univ Tokyo 59:53–66

    Google Scholar 

  • Wang M, Rennolls K (2005) Tree diameter distribution modeling: introducing the logic-logistic distribution. Can J Forest Res 35:1305–1313

    Article  Google Scholar 

  • Wessel P, Smith WHF (1995) New version of the generic mapping tools released. EOS Trans Am Geophys Union 76:329

    Article  Google Scholar 

  • Wong I, Thomas P, Youngs R (2008) Time-dependent seismic hazard in the San Francisco Bay Region, California. In: 14th world conference on earthquake engineering, Beijing, China, pp 07-0110

  • Yadav RBS, Tripathi JN, Rastogi BK, Das MC, Chopra S (2008) Probabilistic assessment of earthquake hazard in Gujarat and adjoining region of India. Pure Appl Geophys 165:1813–1833

    Article  Google Scholar 

  • Yadav RBS, Tripathi JN, Rastogi BK, Das MC, Chopra S (2010) Probabilistic assessment of earthquake recurrence in northeast India and adjoining regions. Pure Appl Geophys 167:1331–1342

    Article  Google Scholar 

  • Zechar JD, Jordan TH (2008) Testing alarm-based earthquake predictions. Geophys J Int 172:715–724

    Article  Google Scholar 

  • Zechar JD, Schorlemmer D, Werner MJ, Gerstenberger MC, Rhoades DA, Jordan TH (2013) Regional earthquake likelihood models I: first order results. Bull Seismol Soc Am 103:787–798

    Article  Google Scholar 

Download references

Acknowledgements

The generic mapping tool (GMT) system (Wessel and Smith 1995) and the MATLAB software (MATLAB 2010) were used for plotting and numerical computation purposes. Constructive comments and important suggestions of two anonymous reviewers are duly acknowledged. This study was partially supported by two grants received from RIG and ACRG of BITS Pilani.

Author information

Authors and Affiliations

  1. Department of Mathematics, Birla Institute of Technology and Science Pilani, Pilani, 333031, India

    Sumanta Pasari

  2. Department of Civil Engineering, Indian Institute of Technology Kanpur, Kanpur, 208016, India

    Onkar Dikshit

Authors
  1. Sumanta Pasari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Onkar Dikshit
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sumanta Pasari.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Pasari, S., Dikshit, O. Stochastic earthquake interevent time modeling from exponentiated Weibull distributions. Nat Hazards 90, 823–842 (2018). https://doi.org/10.1007/s11069-017-3074-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11069-017-3074-1

Keywords

Search

Navigation