Skip to main content
SpringerLink
Log in

Bifurcation analysis of high-speed railway wheel-set

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

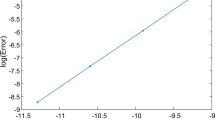
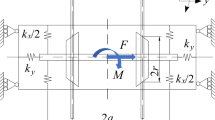
Abstract

In this paper, the lateral mathematical model of a railway wheel-set with two degrees of freedom has been built. To study the effect of yaw damper on the stability of high-speed wheel-set, the constrain between the wheel-set and the bogie is assumed as rigid. In this lateral model, only the nonlinear wheel/rail contact relationship has been taken into consideration in the lateral direction, due to both the nonlinear relationship between lateral displacement and contact angle of the wheel and the rail, and the nonlinear relationship between lateral displacement and the equivalent radius of the wheel at the contact point. And the nonlinear parameters are attained by using polynomial interpolation. By using Center Manifold Theorem, the method of Normal Form and Poincaré method, the model is reduced to a planar dynamical system, and the symbolic expression of the first-order fine focus is given, which can be used to determine which kind of bifurcation will occur at the critical speed. The simulation is established by using the parameters of the wheel-set of Chinese high-speed railway vehicle CRH3, and the result is consistent with the determination of the first-order fine focus. At last, the influence of different parameters on the stability of CRH3 wheel-set is investigated.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Ahmadian, M., Yang, S.: Hopf bifurcation and hunting behaviour in a rail wheel-set with flange contact. Nonlinear Dyn. 15, 15–30 (1998)

    Article  MATH  Google Scholar 

  2. Carr, J.: Applications of Centre Manifold Theory. Springer, New York (1981)

    Book  MATH  Google Scholar 

  3. Chow, S., Li, C., Wang, D.: Normal Forms and Bifurcation of Planar Vector Fields. Cambridge University Press, New York (1994)

    Book  MATH  Google Scholar 

  4. Dong, H., Zeng, J., Xie, J., Jia, L.: Bifurcation\(\backslash \)stability forms of high-speed railway vehicles. Sci. China Technol. Sci. 56, 1685–1696 (2013)

    Article  Google Scholar 

  5. Dukkipati, R.V., Swamy, S.N.: Lateral stability and steady state curving performance of unconventional rail trucks. Mech. Mach. Theory. 36, 577–587 (2001)

    Article  MATH  Google Scholar 

  6. Gan, F., Dai, H., Gao, H., Wei, L.: Calculation of equivalent conicity and wheel-rail contact relationship of differen railway vehicle treads. J. Chin. Railw. Society. 35, 19–24 (2013)

    Google Scholar 

  7. Gao, X.: The “resultant bifurcation diagram” method and its application to bifurcation behaviors of a symmetric railway bogie system. Nonlinear Dyn. 70, 363–380 (2012)

    Article  Google Scholar 

  8. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Springer, New York (1983)

    Book  MATH  Google Scholar 

  9. Hans, T., Engsig-Karup, A., Bigoni, D.: On the numerical and computational aspects of non-smoothnesses that occur in railway vehicle dynamics. Math. Comput. Simul. 95, 78–97 (2014)

    Article  Google Scholar 

  10. Hans, T., Jensen, J.: Chaos and asymmetry in railway vehicle dynamics. Period. Polytech. Transp. Eng. 22, 55–68 (1994)

    Google Scholar 

  11. Haragus, M., Iooss, G.: Local Bifurcations, Center Manifolds, and Normal Forms in Infinite-Dimensional Dynamical Systems. Springer, New York (2011)

    Book  MATH  Google Scholar 

  12. Kalker, J.J.: A fast algorithm for the simplified theory of rolling contact. Int. J. Veh. Styst. Dyn. 11, 1–13 (1982)

    Article  Google Scholar 

  13. Knudsen, C.: Dynamics of a model of a railway wheel-set. Nonlinear Dyn. 6, 215–236 (1994)

    Article  Google Scholar 

  14. Krauskopf, B., Osinga, H.M., Galán-Vioque, J.: Numerical Continuation Methods for Dynamical Systems. Springer, The Netherlands (2007)

    Book  MATH  Google Scholar 

  15. Law, E.H.: Analysis of the nonlinear dynamics of a railway vehicle wheelset. Dyn. Syst. Meas. Control 95, 28–35 (1973)

    Article  Google Scholar 

  16. Park, J., Koh, H., Kim, N.: Parametric study of lateral stability for a railway vehicle. J. Mech. Sci. Technol. 25, 1657–1666 (2011)

    Article  Google Scholar 

  17. Parter, A.D.D.: The approximation determination of the hunting movement of a railway vehicle by aid of the method of Krylov and Bogoljubov. In: Proceedings of the l0th International Congress of Applied Mechanics, Applied Scientific Research. Vol. 10, pp. 205–228 (1960)

  18. Polach, O.: Comparability of the non-linear and linearized stability assessment during railway vehicle design. Int. J. Veh. Styst. Dyn. 44, 129–138 (2006)

    Article  Google Scholar 

  19. Poore, A.B.: On the theory and application of the Hopf-Friedrichs bifurcation theory. Arch. Ration Mech. Anal. 60, 371–393 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  20. Schupp, G.: Computational bifurcation analysis: an efficient method for studying stationary and periodic motions. In: Simpack User Meeting (2004)

  21. Schupp, G.: Bifurcation analysis of railway vehicles. Multibody Syst. Dyn. 15, 25–50 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  22. Sedighi, H., Shirazi, K.: Bifurcation analysis in hunting dynamical behavior in a railway bogie: using novel exact equivalent functions for discontinuous nonlinearities. Sci. Iran. 19, 1493–1501 (2012)

    Article  Google Scholar 

  23. Shabana, A.A., Zaazaa, K.E., Sugiyama, H.: Railroad Vehicle Dynamics: A Computational Approach. Taylor Francis Group, Boca Raton (2008)

    Google Scholar 

  24. Wickens, A.H.: The dynamic stability of railway vehicle wheelsets and bogies having profiled wheels. Int. J. Solids Struct. 1, 319–334 (1965)

    Article  Google Scholar 

  25. Wickens, A.H.: Steering and stability of unsymmetric articulated railway vehicles. Dyn. Syst. Meas. Control 101, 256–262 (1979)

    Article  MATH  Google Scholar 

  26. Zeng, J.: Numerical computations of the hunting bifurcation and limit cycles for railway vehicle system. J. Chin. Railw. Soc. 18, 13–19 (1996)

    Google Scholar 

  27. Zhang, Z., Ding, T., Huang, W., Dong, Z.: Qualitative Theory of Differential Equations. Translations of Mathematical Monographs. American Mathematical Society, Providence (1992)

    Google Scholar 

  28. Zhang, J., Yang, Y., Zeng, J.: An algorithm criterion for Hoopf bifurcation and its applications in vehicle dynamics. Acta. Mech. Sin. 32, 596–605 (2000)

    Google Scholar 

  29. Zobory, I., Nhung, T.: A mathematical investigation of the dynamics of drive-systems of railway traction vehicles under stochastic track excitation. Period. Polytech. Transp. Eng. 20, 101–107 (1992)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. State Key Laboratory of Traction Power, Southwest Jiaotong University, Chengdu, 610031, Sichuan, People’s Republic of China

    Tingting Zhang & Huanyun Dai

Authors
  1. Tingting Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Huanyun Dai
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tingting Zhang.

Additional information

Supported by China Railway Corporation (Grant No. 2014J004-A).

Appendix

Appendix

(1) Expression of \(E_i (i=1,\ldots ,6)\) in Sect. 3.1

$$\begin{aligned} E_1= & {} \displaystyle \frac{3}{8[(\omega ^2-q)^2+p^2\omega ^2]}[p\overline{\mu }_c^2\\&-(p^2-q+\omega ^2)\overline{\mu }_c+p\omega ^2-p],\\ E_5= & {} \displaystyle \frac{3}{8\overline{p}_2^3[(\omega ^2-q)^2+p^2\omega ^2]}\{[\overline{\mu }_c^2\omega ^2(1-\omega ^2)\\&+\,(1-\omega ^2)^3][-p\overline{\mu }_c^2 +(p-q+\omega ^2)\overline{\mu }_c\\&-\,p\omega ^2+p]+[\overline{\mu }_c^3\omega ^2+\overline{\mu }_c(1-\omega ^2)^2][(q-\omega ^2)\overline{\mu }_c^2\\&-\,pq\overline{\mu }_c+(p^2-q+1)\omega ^2+q^2-q]\},\\ E_6= & {} \displaystyle \frac{3p_2p}{8[(\omega ^2-q)^2+p^2\omega ^2]}. \end{aligned}$$

(2) Expression of \(F_i (i=1,\ldots ,6)\) in Sect. 3.2

$$\begin{aligned} E_2= & {} \displaystyle \frac{1}{8\overline{p}_2^2[(\omega ^2-q)^2+p^2\omega ^2]}\{[\overline{\mu }_c^2\omega ^2\\&+3(1-\omega ^2)^2][-p\overline{\mu }_c^2 +(p-q+\omega ^2)\overline{\mu }_c\\&-p\omega ^2+p]+2\overline{\mu }_c(1-\omega ^2)[(q-\omega ^2)\overline{\mu }_c^2\\&-pq\overline{\mu }_c+(p^2-q+1)\omega ^2+q^2-q]\},\\ E_3= & {} \displaystyle \frac{1}{8\overline{p}_2[(\omega ^2-q)^2+p^2\omega ^2]}\{3(1-\omega ^2)\\&\times [-p\overline{\mu }_c^2+(p-q+\omega ^2)\overline{\mu }_c-p\omega ^2+p]\\&+\overline{\mu }_c[(q-\omega ^2)\overline{\mu }_c^2-pq\overline{\mu }_c\\&+(p^2-q+1)\omega ^2+q^2-q]\},\\ E_4= & {} \displaystyle \frac{1}{8\overline{p}_2^2[(\omega ^2-q)^2+p^2\omega ^2]}\{2\overline{\mu }_c\omega ^2(1-\omega ^2) \\&\times [-p\overline{\mu }_c^2+(p-q+\omega ^2)\overline{\mu }_c-p\omega ^2+p]\\&+[3\overline{\mu }_c^2\omega ^2+(1-\omega ^2)^2][(q-\omega ^2)\overline{\mu }_c^2-pq\overline{\mu }_c\\&+(p^2-q+1)\omega ^2+q^2-q]\}, \end{aligned}$$
$$\begin{aligned} F_1= & {} \displaystyle \frac{3}{8[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]} \\&\times (-3\alpha ^2\overline{\mu }_c-2\alpha \overline{\mu }_c^2 -2\alpha \omega _1^2-\overline{\mu }_c\omega _1^2+\overline{\mu }_c\omega _2^2+2\alpha ),\\ F_2= & {} \displaystyle \frac{1}{8\overline{p}_2^2[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]}\\&\times \{[3(1-\omega _1^2)^2+\omega _1^2\overline{\mu }_c^2](3\alpha ^2\overline{\mu }_c +2\alpha \overline{\mu }_c^2+2\alpha \omega _1^2\\&+\overline{\mu }_c\omega _1^2- \overline{\mu }_c\omega _2^2-2\alpha )+2\overline{\mu }_c(1-\omega _1^2) (\alpha ^4\\&+2\alpha ^3\overline{\mu }_c+\alpha ^2\overline{\mu }_c^2+3\alpha ^2\omega _1^2 +2\alpha ^2\omega _2^2\\&+2\alpha \overline{\mu }_c\omega _2^2-\overline{\mu }_c^2\omega _1^2 +\overline{\mu }_c^2\omega _2^2-\omega _1^2\omega _2^2\\&+\omega _2^4-\alpha ^2+\omega _1^2-\omega _2^2)\},\\ F_3= & {} \displaystyle \frac{1}{8\overline{p}_2[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]}\\&\times [3(1-\omega _1^2)(3\alpha ^2\overline{\mu }_c+2\alpha \overline{\mu }_c^2 +2\alpha \omega _1^2+\overline{\mu }_c\omega _1^2\\&-\overline{\mu }_c\omega _2^2-2\alpha )+\overline{\mu }_c (\alpha ^4+2\alpha ^3\overline{\mu }_c+\alpha ^2\overline{\mu }_c^2\\&+3\alpha ^2\omega _1^2 +2\alpha ^2\omega _2^2+2\alpha \overline{\mu }_c\omega _2^2-\overline{\mu }_c^2\omega _1^2\\&+\overline{\mu }_c^2\omega _2^2 -\omega _1^2\omega _2^2+\omega _2^4-\alpha ^2+\omega _1^2-\omega _2^2)],\\ F_4= & {} \displaystyle \frac{1}{8\overline{p}_2^2[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]}\\&\times \{2\omega _1\overline{\mu }_c(1-\omega _1^2)(3\alpha ^2\overline{\mu }_c+2\alpha \overline{\mu }_c^2 +2\alpha \omega _1^2\\&+\overline{\mu }_c\omega _1^2-\overline{\mu }_c\omega _2^2-2\alpha ) +[3\omega _1\overline{\mu }_c^2+(1-\omega _1^2)^2](\alpha ^4\\&+2\alpha ^3\overline{\mu }_c+\alpha ^2\overline{\mu }_c^2+3\alpha ^2\omega _1^2\\&+2\alpha ^2\omega _2^2+2\alpha \overline{\mu }_c\omega _2^2-\overline{\mu }_c^2\omega _1^2 +\overline{\mu }_c^2\omega _2^2-\omega _1^2\omega _2^2\\&+\omega _2^4-\alpha ^2+\omega _1^2-\omega _2^2)\},\\ F_5= & {} \displaystyle \frac{3}{8\overline{p}_2^3[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]}\\&\times \{[(1-\omega _1^2)^3+\omega _1^2\overline{\mu }_c^2(1-\omega _1^2)](3\alpha ^2\overline{\mu }_c+2\alpha \overline{\mu }_c^2\\&+2\alpha \omega _1^2+\overline{\mu }_c\omega _1^2-\overline{\mu }_c\omega _2^2-2\alpha )+[\overline{\mu }_c(1-\omega _1^2)^2\\&+\omega _1^2\overline{\mu }_c^3](\alpha ^4+2\alpha ^3\overline{\mu }_c+\alpha ^2\overline{\mu }_c^2+3\alpha ^2\omega _1^2\\&+2\alpha ^2\omega _2^2+2\alpha \overline{\mu }_c\omega _2^2-\overline{\mu }_c^2\omega _1^2 +\overline{\mu }_c^2\omega _2^2\\&-\omega _1^2\omega _2^2+\omega _2^4-\alpha ^2+\omega _1^2-\omega _2^2)\},\\ F_6= & {} -\displaystyle \frac{3\alpha \overline{p}_2}{4[\alpha ^2+(\omega _1-\omega _2)^2][\alpha ^2+(\omega _1+\omega _2)^2]}. \end{aligned}$$

(3) Parameters of CRH3 wheel-set in Sect. 4

Parameter

Comments

Value

M

Wheel-set mass

5279 kg

I

Yaw moment of wheel-set

5460 N m

\(r_0\)

Wheel radius without lateral displacement

460 mm

a

Half of the rolling cycle gauge

0.7465 m

b

Half of the air spring arm

0.95 m

Parameter

Comments

Value

\(K_{2x}\)

Secondary longitudinal stiffness

4.383\(\mathrm{MN}\,\mathrm{m}^{-1}\)

\(K_{2y}\)

Secondary lateral stiffness

0.133 MN \(\mathrm{m}^{-1}\)

\(C_{2x}\)

Yaw damper

0.33 MN/(m/s)

\(f_{11}\)

Longitudinal creep coefficient

3 MN

\(f_{22}\)

Longitudinal creep coefficient

3 MN

k

Coefficient relative to cosine value of wheel–rail contact angle

1.994

g

Gravitational acceleration

9.81 \(\mathrm{m/s}^{2}\)

\(e_0\)

Coefficient relative to tangent value of wheel–rail contact angle

22.77

\(e_2\)

Coefficient relative to tangent value of wheel–rail contact angle

294502

\(\xi _1\)

Coefficient relative to the equivalent radius of left/right wheel

0.20

\(\xi _2\)

Coefficient relative to the equivalent radius of left/right wheel

11.77

\(\xi _3\)

Coefficient relative to the equivalent radius of left/right wheel

−6140.54

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, T., Dai, H. Bifurcation analysis of high-speed railway wheel-set. Nonlinear Dyn 83, 1511–1528 (2016). https://doi.org/10.1007/s11071-015-2425-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-015-2425-2

Keywords

Search

Navigation