Skip to main content
SpringerLink
Log in

Ising limit of a Heisenberg XXZ magnet and some temperature correlation functions

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

Abstract

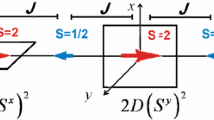
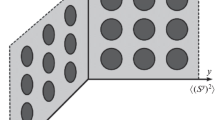
We consider the Heisenberg spin-1/2 XXZ magnet in the case where the anisotropy parameter tends to infinity (the so-called Ising limit). We find the temperature correlation function of a ferromagnetic string above the ground state. Our approach to calculating correlation functions is based on expressing the wave function in the considered limit in terms of Schur symmetric functions. We show that the asymptotic amplitude of the above correlation function at low temperatures is proportional to the squared number of strict plane partitions in a box.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. N. Vasiliev, Functional Methods in Quantum Field Theory and Statistics [in Russian], Leningrad State Univ. Press, Leningrad (1976); English transl.: Functional Methods in Quantum Field Theory and Statistical Physics, Gordon and Breach, Amsterdam (1998).

    Google Scholar 

  2. N. M. Bogolyubov, V. F. Brattsev, A. N. Vasil’ev, A. L. Korzhenevskii, and R. A. Radzhabov, Theor. Math. Phys., 26, 230–237 (1976).

    Article  Google Scholar 

  3. C. N. Yang and C. P. Yang, Phys. Rev., 151, 258–264 (1966).

    Article  ADS  Google Scholar 

  4. M. Gaudin, La fonction d’onde de Bethe, Masson, Paris (1983).

    MATH  Google Scholar 

  5. L. D. Faddeev, Sov. Sci. Rev. C, 1, 107–155 (1980); “Quantum completely integrable models in field theory,” in: 40 Years in Mathematical Physics (World Sci. Ser. 20th Century Math., Vol. 2), World Scientific, River Edge, N. J. (1995), p. 187–235.

    MATH  Google Scholar 

  6. N. M. Bogolyubov, A. G. Izergin, and V. E. Korepin, Correlation Functions of Integrable Systems and the Quantum Inverse Problem Method [in Russian], Nauka, Moscow (1992).

    MATH  Google Scholar 

  7. V. E. Korepin, N. M. Bogoliubov, and A. G. Izergin, Quantum Inverse Scattering Method and Correlation Functions (Cambridge Monogr. Math. Phys., Vol. 13), Cambridge Univ. Press, Cambridge (1993).

    Book  MATH  Google Scholar 

  8. V. E. Korepin, Commun. Math. Phys., 86, 391–418 (1982).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  9. A. G. Izergin and V. E. Korepin, Commun. Math. Phys., 99, 271–302 (1985).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  10. N. Kitanine, J.-M. Maillet, and V. Terras, Nucl. Phys. B, 554, 647–678 (1999); arXiv:math-ph/9807020v1 (1998).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  11. N. Kitanine, J. M. Maillet, N. A. Slavnov, and V. Terras, Nucl. Phys. B, 642, 433–455 (2002).

    Article  MATH  MathSciNet  Google Scholar 

  12. I. G. Macdonald, Symmetric Functions and Hall Polynomials, Clarendon, Oxford (1998).

    MATH  Google Scholar 

  13. M. E. Fisher, J. Statist. Phys., 34, 667–729 (1984).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  14. T. Nagao and P. J. Forrester, Nucl. Phys. B, 620, 551–565 (2002); arXiv:cond-mat/0107221v2 (2001).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  15. C. Krattenthaler, A. J. Guttmann, and X. G. Viennot, J. Phys. A, 33, 8835–8866 (2000); arXiv:cond-mat/0006367v2 (2000).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  16. M. Katori, H. Tanemura, T. Nagao, and N. Komatsuda, Phys. Rev. E, 68, 021112 (2003); arXiv:cond-mat/0303573v2 (2003).

    Article  ADS  Google Scholar 

  17. G. Schehr, S. N. Majumdar, A. Comtet, and J. Randon-Furling, Phys. Rev. Lett., 101, 150601 (2008); arXiv:0807.0522v2 [cond-mat.stat-mech] (2008).

    Article  ADS  MathSciNet  Google Scholar 

  18. D. M. Bressoud, Proofs and Confirmations: The Story of the Alternating Sign Matrix Conjecture (MAA Spectrum Series, Vol. 15), Cambridge Univ. Press, Cambridge (1999).

    Google Scholar 

  19. N. M. Bogoliubov, J. Phys. A, 38, 9415–9430 (2005); arXiv:cond-mat/0503748v1 (2005).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  20. N. M. Bogolyubov, J. Math. Sci. (New York), 138, 5636–5643 (2006).

    Article  MathSciNet  Google Scholar 

  21. N. M. Bogoliubov and C. Malyshev, “A path integration approach to the correlators of XY Heisenberg magnet and random walks,” in: Proc. 9th Intl. Conf. “Path Integrals: New Trends and Perspectives” (Dresden, Germany, 23–28 September 2007, W. Janke and A. Pelster, eds.), World Scientific, Hackensack, N. J. (2008), p. 508–513; arXiv:0810.4816v1 [cond-mat.stat-mech] (2008).

    Chapter  Google Scholar 

  22. N. M. Bogolyubov, Theor. Math. Phys., 155, 523–535 (2008).

    Article  MathSciNet  Google Scholar 

  23. N. M. Bogolyubov and K. L. Malyshev, Theor. Math. Phys., 159, 563–574 (2009); arXiv:0903.3227v2 [condmat. stat-mech] (2009).

    Article  MathSciNet  Google Scholar 

  24. N. M. Bogoliubov and K. Malyshev, St. Petersburg Math. J., 22, 359–377 (2011).

    Article  MATH  MathSciNet  Google Scholar 

  25. E. Lieb, T. Schultz, and D. Mattis, Ann. Phys., 16, 407–466 (1961).

    Article  MATH  ADS  MathSciNet  Google Scholar 

  26. T. Niemeijer, Phys., 36, 377–419 (1967); 39, 313–326 (1968).

    ADS  Google Scholar 

  27. F. Colomo, A. G. Izergin, V. E. Korepin, and V. Tognetti, Theor. Math. Phys., 94, 11–38 (1993).

    Article  MathSciNet  Google Scholar 

  28. K. L. Malyshev, Theor. Math. Phys., 136, 1143–1154 (2003).

    Article  MATH  MathSciNet  Google Scholar 

  29. F. C. Alcaraz and R. Z. Bariev, “An exactly solvable constrained XXZ chain,” in: Statistical Physics on the Eve of the 21st Century (Ser. Adv. Statist. Mech., Vol. 14, M. T. Batchelor and L. T. Wille, eds.), World Scientific, River Edge, N. J. (1999), pp. 412–424; arXiv:cond-mat/9904042v1 (1999).

    Google Scholar 

  30. N. I. Abarenkova and A. G. Pronko, Theor. Math. Phys., 131, 690–703 (2002).

    Article  MATH  MathSciNet  Google Scholar 

  31. A. J. A. James, W. D. Goetze, and F. H. L. Essler, Phys. Rev. B, 79, 214408 (2009); arXiv:0902.2402v2 [cond-mat.str-el] (2009).

    Article  ADS  Google Scholar 

  32. F. R. Gantmakher, Theory of Matrices [in Russian], Nauka, Moscow (1988).

    MATH  Google Scholar 

  33. L. A. Takhtadzhyan and L. D. Faddeev, Russ. Math. Surveys, 34, 11–68 (1979).

    Article  ADS  MathSciNet  Google Scholar 

  34. E. H. Lieb and F. Y. Wu, “Two dimensional ferroelectric models,” in: Phase Transitions and Critical Phenomena (C. Domb and M. Green, eds.), Vol. 1, Acad. Press, London (1972), p. 331–490.

    Google Scholar 

  35. V. O. Tarasov, L. A. Takhtadzhyan, and L. D. Faddeev, Theor. Math. Phys., 57, 1059–1073 (1983).

    Article  MathSciNet  Google Scholar 

  36. M. L. Mehta, Random Matrices, Acad. Press, Boston, Mass. (1991).

    MATH  Google Scholar 

  37. N. Bogoliubov and J. Timonen, Phil. Trans. Roy. Soc. London A, 369, 1319–1333 (2011).

    Article  MATH  ADS  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. St. Petersburg Department of the Steklov Institute of Mathematics, RAS, St. Petersburg, Russia

    N. M. Bogoliubov & C. L. Malyshev

Authors
  1. N. M. Bogoliubov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. C. L. Malyshev
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to C. L. Malyshev.

Additional information

__________

Translated from Teoreticheskaya i Matematicheskaya Fizika, Vol. 169, No. 2, pp. 179–193, November, 2011.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bogoliubov, N.M., Malyshev, C.L. Ising limit of a Heisenberg XXZ magnet and some temperature correlation functions. Theor Math Phys 169, 1517–1529 (2011). https://doi.org/10.1007/s11232-011-0129-4

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11232-011-0129-4

Keywords

Search

Navigation