Skip to main content
SpringerLink
Log in

On solving two higher-order nonlinear PDEs describing the propagation of optical pulses in optic fibers using the \(\left( \frac{G^{\prime }}{G},\frac{1}{G}\right) \)-expansion method

  • Published:
Ricerche di Matematica Aims and scope Submit manuscript

Abstract

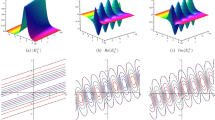
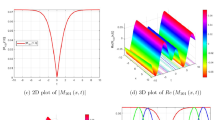
The propagation of the optical solitons is usually governed by the nonlinear Schrödinger equations. In this article, the two variable \((\frac{ G^{\prime }}{G},\frac{1}{G})\)-expansion method is employed to construct the exact traveling wave solutions with parameters of two nonlinear PDEs namely, the (2\(+\)1)-dimensional nonlinear cubic–quintic Ginzburg–Landau equation and the (1\(+\)1)-dimensional resonant nonlinear Schrödinger’s equation with dual-power law nonlinearity which describe the propagation of optical pulses in optic fibers. When the parameters are replaced by special values, the well-known solitary wave solutions of these equations rediscovered from the traveling waves. This method can be thought of as the generalization of well-known original \((\frac{G^{\prime }}{G})\)-expansion method proposed by M. Wang et al. It is shown that the two variable \((\frac{G^{\prime }}{G}, \frac{1}{G})\)-expansion method provides a more powerful mathematical tool for solving many other nonlinear PDEs in mathematical physics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Tian, B., Gao, Y.T., Zhu, H.W.: Variable-coefficient higher-order nonlinear Schrödinger model in optical fibers: variable-coefficient bilinear form, Bäcklund transformation, brightons and symbolic computation. Phys. Lett. A 366, 223–229 (2007)

    Article  MATH  Google Scholar 

  2. Nakazawa, M., Kubota, H., Suzuki, K., Yamada, E., Sahara, A.: Recent progress in soliton transmission technology. Chaos 10, 486–514 (2000)

    Article  Google Scholar 

  3. Peacock, A.C., Kruhlak, R.J., Harvey, J.D., Dudley, J.M.: Solitary pulse propagation in high gain optical fiber amplifiers with normal group velocity dispersion. Opt. Commun. 206, 171–177 (2002)

    Article  Google Scholar 

  4. Tian, B., Gao, Y.T.: Symbolic computation on cylindrical-modified dust-ion-acoustic nebulons in dusty plasmas. Phys. Lett. A 362, 283–288 (2007)

    Article  MATH  Google Scholar 

  5. Hao, R., Li, L., Li, Z., Xue, W., Zhou, G.: A new approach to exact soliton solutions and soliton interaction for the nonlinear Schrödinger equation with variable coefficients. Opt. Commun. 236, 79–86 (2004)

    Article  Google Scholar 

  6. Gedalin, M., Scott, T.C., Band, Y.B.: Optical solitary waves in the higher order nonlinear Schrödinger equation. Phys. Rev. Lett. 78, 448–451 (1997)

    Article  Google Scholar 

  7. Mihalache, D., Torner, L., Moldoveanu, F., Panoiu, N.C., Truta, N.: Inverse scattering approach to femtosecond solitons in monomode optical fibers. Phys. Rev. E 48, 4699–4709 (1993)

    Article  Google Scholar 

  8. Shi, Y., Dai, Z., Li, D.: Application of Exp-function method for 2D cubic-quintic Ginzburg-Landau equation. Appl. Math. Comput. 210, 269–275 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  9. Triki, H., Hayat, T., Aldossary, O.M., Biswas, A.: Bright and dark solitons for the resonant nonlinear Schrödinger’s equation with time-dependent coefficients. Opt. Laser Technol. 44, 2223–2231 (2012)

    Article  Google Scholar 

  10. Ablowitz, M.J., Clarkson, P.A.: Solitons. Nonlinear Evolution Equations and Inverse Scattering Transform. Cambridge University Press, New York (1991)

    MATH  Google Scholar 

  11. Hirota, R.: Exact solutions of the KdV equation for multiple collisions of solutions. Phys. Rev. Lett. 27, 1192–1194 (1971)

    Article  MATH  Google Scholar 

  12. Weiss, J., Tabor, M., Carnevale, G.: The Painlev é property for partial differential equations. J. Math. Phys. 24, 522–526 (1983)

    Article  MATH  MathSciNet  Google Scholar 

  13. Kudryashov, N.A.: On types of nonlinear nonintegrable equations with exact solutions. Phys. Lett. A 155, 269–275 (1991)

    Article  MathSciNet  Google Scholar 

  14. Rogers, C., Shadwick, W.F.: Backlund Transformations and Their Applications. Academic Press, New York (1982)

    Google Scholar 

  15. Zhang, S.: Application of Exp-function method to high-dimensional nonlinear evolution equations. Chaos Solitons Fractals 38, 270–276 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  16. Bekir, A.: Application of the Exp-function method for nonlinear differential-difference equations. Appl. Math. Comput. 215, 4049–4053 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  17. Abdou, M.A.: The extended tanh-method and its applications for solving nonlinear physical models. Appl. Math. Comput. 190, 988–996 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  18. Yusufoglu, E., Bekir, A.: Exact solutions of coupled nonlinear Klein-Gordon equations. Math. Comput. Model. 48, 1694–1700 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  19. Zayed, E.M.E., Alurrfi, K.A.E.: The modified extended tanh-function method and its applications to the generalized KdV-mKdV equation with any-order nonlinear terms. Int. J. Environ. Eng. Sci. Technol. Res. 1(8), 165–170 (2013)

    Google Scholar 

  20. Chen, Y., Wang, Q.: Extended Jacobi elliptic function rational expansion method and abundant families of Jacobi elliptic function solutions to (1+1)-dimensional dispersive long wave equation. Chaos Solitons Fractals 24, 745–757 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  21. Lu, D.: Jacobi elliptic function solutions for two variant Boussinesq equations. Chaos Solitons Fractals 24, 1373–1385 (2005)

    Article  MathSciNet  Google Scholar 

  22. Wang, M.L., Li, X., Zhang, J.: The \((\frac{G}{G}^{\prime })\)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 372, 417–423 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  23. Zhang, S., Tong, J.L., Wang, W.: A generalized \((\frac{G}{G} ^{\prime })\)-expansion method for the mKdV equation with variable coefficients. Phys. Lett. A 372, 2254–2257 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  24. Zayed, E.M.E., Gepreel, K.A.: The \((\frac{G}{G}^{\prime })\)-expansion method for finding traveling wave solutions of nonlinear partial differential equations in mathematical physics. J. Math. Phys. 50, 013502–013512 (2009)

    Article  MathSciNet  Google Scholar 

  25. Zayed, E.M.E.: The \((\frac{G}{G}^{\prime })\)-expansion method and its applications to some nonlinear evolution equations in the mathematical physics. J. Appl. Math. Comput. 30, 89–103 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  26. Zayed, E.M.E.: Traveling wave solutions for higher dimensional nonlinear evolution equations using the \((\frac{G}{G}^{\prime })\)-expansion method. J. Appl. Math. Inform. 28, 383–395 (2010)

    MATH  Google Scholar 

  27. Zayed, E.M.E., Gepreel, K.A.: The modified \((\frac{G}{G} ^{\prime })\)-expansion method and its applications to construct exact solutions for nonlinear PDEs. Wseas Trans. Math. 8, 270–278 (2011)

    Google Scholar 

  28. Zhang, S., SUN, Y.N., Ba, J.M., Dong, L.: The modified \((\frac{G }{G}^{\prime })\)-expansion method for nonlinear evolution equations. Z. Naturforsch. 66a, 33–39 (2011)

  29. Li, L.X., Li, Q.E., Wang, L.M.: The \((\frac{G}{G}^{\prime }, \frac{1}{G})\)-expansion method and its application to travelling wave solutions of the Zakharov equations. Appl. Math. J. Chin. Univ. 25, 454–462 (2010)

    Article  MATH  Google Scholar 

  30. Zayed, E.M.E., Abdelaziz, M.A.M.: The two variables \(( \frac{G}{G}^{\prime },\frac{1}{G})\)-expansion method for solving the nonlinear KdV-mKdV equation. Math. Probl. Eng. 2012, 1–14 (2012). Art. ID 725061

  31. Zayed, E.M.E., Hoda Ibrahim, S.A., Abdelaziz, M.A.M.: Traveling wave solutions of the nonlinear (3+1) dimensional Kadomtsev-Petviashvili equation using the two variables \((\frac{G}{G} ^{\prime },\frac{1}{G})\)-expansion method. J. Appl. Math, 2012, 1–8 (2012). Art. ID 560531

  32. Zayed, E.M.E., Hoda Ibrahim, S.A.: The two variable \((\frac{G}{ G}^{\prime },\frac{1}{G})\)-expansion method for finding exact traveling wave solutions of the (3+1)-dimensional nonlinear potential Yu-Toda-Sasa-Fukuyama equation. In: International Conference on Advanced Computer Science and Electronics Information. Atlantis Press, Amsterdam, pp. 388–392 (2013)

  33. Zayed, E.M.E., Alurrfi, K.A.E.: The \((\frac{G}{G}^{\prime },\frac{1}{G})\)-expansion method and its applications to find the exact solutions of nonlinear PDEs for nanobiosciences. Math. Probl. Eng. 2014, 1–10 (2014). Art. ID 521712

  34. Zayed, E.M.E., Alurrfi, K.A.E.: The \((\frac{G}{G}^{\prime },\frac{1}{G})\)-expansion method and its applications for solving two higher order nonlinear evolution equations. Math. Probl. Eng. 2014, 1–20 (2014). Art. 746538

  35. Zayed, E.M.E., Alurrfi, K.A.E.: On solving the nonlinear Schrödinger-Boussinesq equation and the hyperbolic Schrödinger equation by using the \((\frac{G}{G}^{\prime },\frac{1}{G})\)-expansion method. Int. J. Phys. Sci. 19, 415–429 (2014)

  36. Li, B., Chan, Y., Zhang, H.: Explicit exact solutions for new general two-dimensional KdVtype and two dimensional KdV Burgers type equations with nonlinear terms of any order. J. Phys. A Math. Gen. 35, 8253–8265 (2002)

    Article  MATH  Google Scholar 

Download references

Acknowledgments

The authors wish to thank the referee for his comments on this paper.

Conflict of interest

The authors declare that there is no conflict of interests regarding the publication of this paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Zagazig University, P.O.Box 44519, Zagazig, Egypt

    E. M. E. Zayed & K. A. E. Alurrfi

Authors
  1. E. M. E. Zayed
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. K. A. E. Alurrfi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to E. M. E. Zayed.

Additional information

Communicated by Salvatore Rionero.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zayed, E.M.E., Alurrfi, K.A.E. On solving two higher-order nonlinear PDEs describing the propagation of optical pulses in optic fibers using the \(\left( \frac{G^{\prime }}{G},\frac{1}{G}\right) \)-expansion method. Ricerche mat. 64, 167–194 (2015). https://doi.org/10.1007/s11587-015-0226-z

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11587-015-0226-z

Keywords

Mathematics Subject Classification

Search

Navigation