Skip to main content
SpringerLink
Log in

Solving Volterra’s population growth model of arbitrary order using the generalized fractional order of the Chebyshev functions

  • Published:
Ricerche di Matematica Aims and scope Submit manuscript

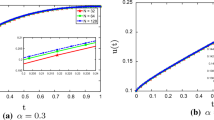
Abstract

Volterra’s model for population growth in a closed system includes an integral term to indicate accumulated toxicity in addition to the usual terms of the logistic equation, that occurs in ecology. In this paper, a new numerical approximation is introduced for solving this model of arbitrary (integer or fractional) order. The proposed numerical approach is based on the generalized fractional order Chebyshev orthogonal functions of the first kind and the collocation method. Accordingly, we employ a collocation approach, by computing through Volterra’s population model in the integro-differential form. This method reduces the solution of a problem to the solution of a nonlinear system of algebraic equations. To illustrate the reliability of this method, we compare the numerical results of the present method with some well-known results in order to show that the new method is efficient and applicable.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Boyd, J.P.: Chebyshev spectral methods and the Lane–Emden problem. Numer. Math. Theor. Methods Appl. 4(2), 142–157 (2011)

    MathSciNet  MATH  Google Scholar 

  2. Parand, K., Nikarya, M., Rad, J.A.: Solving non-linear Lane–Emden type equations using Bessel orthogonal functions collocation method. Celest. Mech. Dyn. Astr. 16(21), 97–107 (2013)

    Article  MathSciNet  Google Scholar 

  3. Shen, J., Tang, T., Wang, L.L.: Spectral Methods Algorithms, Analysis and Applications, 1st edn. Springer, New York (2001)

    MATH  Google Scholar 

  4. Rad, J.A., Parand, K., Ballestra, L.V.: Pricing European and American options by radial basis point interpolation. Appl. Math. Comput. 251, 363–377 (2015)

    MathSciNet  MATH  Google Scholar 

  5. D’Amore, L.: Remarks on numerical algorithms for computing the inverse Laplace transform. Ricerche Mat. 63(2), 239–252 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  6. Parand, K., Dehghan, M., Pirkhedri, A.: The Sinc-collocation method for solving the Thomas–Fermi equation. J. Comput. Appl. Math. 237(1), 244–252 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  7. Shen, J., Tang, T.: High Order Numerical Methods and Algorithms. Chinese Science Press, China (2005)

    Google Scholar 

  8. Rad, J.A., Parand, K., Kazem, S.: A numerical investigation to viscous flow over nonlinearly stretching sheet with chemical reaction, heat transfer and magnetic field. Int. J. Appl. Comput. Math. In press pp. 1–17 (2016)

  9. Rad, J.A., Parand, K., Abbasbandy, S.: Local weak form meshless techniques based on the radial point interpolation (RPI) method and local boundary integral equation (LBIE) method to evaluate European and American options. Commun. Nonlinear Sci. Numer. Simul. 22(1), 1178–1200 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  10. Parand, K., Dehghan, M., Taghavi, A.: Modified generalized Laguerre function Tau method for solving laminar viscous flow: the Blasius equation. Int. J. Numer. Methods. Heat 20(7), 728–743 (2010)

    Article  MathSciNet  Google Scholar 

  11. Kazem, S., Abbasbandy, S., Kumar, S.: Fractional-order Legendre functions for solving fractional-order differential equations. Appl. Math. Model. 37, 5498–5510 (2013)

    Article  MathSciNet  Google Scholar 

  12. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, San Diego (2006)

    MATH  Google Scholar 

  13. Delkhosh, M.: Introduction of derivatives and integrals of fractional order and its applications. Appl. Math. Phys. 1(4), 103–119 (2013)

    Google Scholar 

  14. Odibat, Z., Momani, S.: An algorithm for the numerical solution of differential equations of fractional order. J. Appl. Math. Inf. 26, 15–27 (2008)

    MATH  Google Scholar 

  15. Szego, G.: Orthogonal Polynomials. American Mathematical Society Providence, Rhode Island (1975)

    MATH  Google Scholar 

  16. Ross, R.: The Prevention of Malaria. E.P. Dutton & Company, New York, London (1911)

    Google Scholar 

  17. D’Ancona, U.: La Lotta per l’Esistenza. G. Einaudi, Torino (1942)

    MATH  Google Scholar 

  18. Sharpe, F.R., Lotka, A.J.: Contribution to the analysis of malaria epidemiology IV: Incubation lag. Am. J. Epidemiol. 3, 96–112 (1923)

    Google Scholar 

  19. Lotka, A.: Elements of Physical Biology. Williams & Wilkins Company, New York (1925)

    MATH  Google Scholar 

  20. Scudo, F.M.: Vito Volterra and theoretical ecology. Theor. Popul. Biol. 2(1), 1–23 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  21. TeBeest, K.G.: Numerical and analytical solutions of Volterra’s population model. SIAM Rev. 39(3), 484–493 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  22. Small, R.D.: Population growth in a closed system. SIAM Rev. 25(1), 93–95 (1983)

    Article  MATH  Google Scholar 

  23. Wazwaz, A.M.: Analytical approximation and Pade approximation for Volterra’s population model. Appl. Math. Comput. 100, 13–25 (1999)

    MathSciNet  MATH  Google Scholar 

  24. Parand, K., Rezaei, A., Taghavi, A.: Numerical approximations for population growth model by rational Chebyshev and Hermite functions collocation approach: a comparison. Math. Methods Appl. Sci. 33(17), 2076–2086 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  25. Parand, K., Delafkar, Z., Pakniat, N., Pirkhedri, A., Haji, M.K.: Collocation method using Sinc and Rational Legendre function for solving Volterra’s population model. Commun. Nonlinear Sci. Numer. Simul. 16, 1811–1819 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  26. Parand, K., Abbasbandy, S., Kazem, S., Rad, J.A.: A novel application of radial basis functions for solving a model of first-order integro-ordinary differential equation. Commun. Nonlinear Sci. Num. Simul. 16, 4250–4258 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  27. Parand, K., Rad, J.A., Nikarya, M.: A new numerical algorithm based on the first kind of modified Bessel function to solve population growth in a closed system. Int. J. Comput. Math. 91(6), 1239–1254 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  28. Parand, K., Hojjati, G.: Solving Volterra’s population model using new second derivative multistep methods. Am. J. Appl. Sci. 5(8), 1019–1022 (2008)

    Article  Google Scholar 

  29. Parand, K., Hossayni, S.A., Rad, J.A.: Operation matrix method based on Bernstein polynomials for the Riccati differential equation and Volterra population model. Appl. Math. Model. 40(2), 993–1011 (2016)

    Article  MathSciNet  Google Scholar 

  30. Momani, S., Qaralleh, R.: Numerical approximations and Pade approximations for a fractional population growth model. Appl. Math. Model. 31, 1907–1914 (2007)

    Article  MATH  Google Scholar 

  31. Xu, H.: Analytical approximations for a population growth model with fractional order. Commun. Nonliear Sci. Numer. Simul. 14, 1978–1983 (2009)

    Article  MATH  Google Scholar 

  32. Luca, R.D.: On the long-time dynamics of nonautonomous predator-prey models with mutual interference. Ricerche Mat. 61(2), 275–290 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  33. Messina, E., Russo, E., Vecchio, A.: Volterra integral equations on time scales: stability under constant perturbations via Liapunov direct method. Ricerche Mat. 64(2), 345–355 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  34. Erturk, V.S., Yildirim, A., Momanic, S., Khan, Y.: The differential transform method and Pade approximates for a fractional population growth model. Int. J. Numer. Method. Hydrol. 22(6), 791–802 (2012)

    Article  MathSciNet  Google Scholar 

  35. Momani, S., Qaralleh, R.: Numerical approximations and Pade approximates for a fractional population growth model. Appl. Math. Model. 31, 1907–1914 (2007)

    Article  MATH  Google Scholar 

  36. Yuzbasi, S.: A numerical approximation for Volterra’s population growth model with fractional order. Appl. Math. Model. 37, 3216–3227 (2013)

    Article  MathSciNet  Google Scholar 

  37. Parand, K., Nikarya, M.: Application of Bessel functions for solving differential and integro-differential equations of the fractional order. Appl. Math. Model. 38, 4137–4147 (2014)

    Article  MathSciNet  Google Scholar 

  38. Maleki, M., Kajani, M.T.: Numerical approximations for Volterra’s population growth model with fractional order via a multi-domain pseudospectral method. Appl. Math. Model. 39(15), 4300–4308 (2015)

    Article  MathSciNet  Google Scholar 

  39. Khan, N.A., Mahmood, A., Khan, N.A., Ara, A.: Analytical study of nonlinear fractional-order integro-differential equation: Revisit Volterra’s population model. Int. J. Differ. Equ. 2012, 8 Article ID 845945 (2012)

  40. Ghasemi, M., Fardi, M., Ghazizni, R.K.: A new application of the homotopy analysis method in solving the fractional Volterra’s population system. Appl. Math. 59(3), 319–330 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  41. Eslahchi, M.R., Dehghan, M., Amani, S.: Chebyshev polynomials and best approximation of some classes of functions. J. Numer. Math. 23(1), 41–50 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  42. Bhrawy, A.H., Alofi, A.S.: The operational matrix of fractional integration for shifted Chebyshev polynomials. Appl. Math. Lett. 26, 25–31 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  43. Doha, E.H., Bhrawy, A.H., Ezz-Eldien, S.S.: A Chebyshev spectral method based on operational matrix for initial and boundary value problems of fractional order. Comput. Math. Appl. 62, 2364–2373 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  44. Nkwanta, A., Barnes, E.R.: Two Catalan-type Riordan arrays and their connections to the Chebyshev polynomials of the first kind. J. Integer Seq. 15, 1–19 (2012)

    MathSciNet  MATH  Google Scholar 

  45. Lslie, F., Parker, I.B.: Chebyshev Polynomials in Numerical Analysis, 29th edn. Oxford University Press, London (1968)

    Google Scholar 

  46. Saadatmandi, A., Dehghan, M.: Numerical solution of hyperbolic telegraph equation using the Chebyshev tau method. Numer. Methods. Partial Differ. Equ. 26(1), 239–252 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  47. Parand, K., Taghavi, A., Shahini, M.: Comparison between rational Chebyshev and modified generalized Laguerre functions pseudospectral methods for solving Lane–Emden and unsteady gas equations. Acta Phys. Polon. B 40(12), 1749–1763 (2009)

    MATH  Google Scholar 

  48. Parand, K., Shahini, M., Taghavi, A.: Generalized Laguerre polynomials and rational Chebyshev collocation method for solving unsteady gas equation. Int. J. Contemp. Math. Sci. 4(21), 1005–1011 (2009)

    MathSciNet  MATH  Google Scholar 

  49. Parand, K., Abbasbandy, S., Kazem, S., Rezaei, A.R.: An improved numerical method for a class of astrophysics problems based on radial basis functions. Physica Script. 83, 11, 015011 (2011)

  50. Parand, K., Shahini, M.: Rational Chebyshev pseudospectral approach for solving Thomas–Fermi equation. Phys. Lett. A 373, 210–213 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  51. Parand, K., Khaleqi, S.: The rational Chebyshev of second kind collocation method for solving a class of astrophysics problems. Euro. Phys. J. Plus 131, 1–24 (2016)

    Article  Google Scholar 

  52. Parand, K., Shahini, M., Dehghan, M.: Solution of a laminar boundary layer flow via a numerical method. Commun. Nonlinear Sci. Num. Simul. 15(2), 360–367 (2010)

    Article  MATH  Google Scholar 

  53. Adomian, G.: Solving Frontier problems of Physics: The Decomposition Method. Kluwer Academic Publishers, Kluwer (1994)

    Book  MATH  Google Scholar 

  54. Liao, S.J.: The proposed homotopy analysis technique for the solution of nonlinear problems. PhD thesis, Shanghai Jiao Tong University (1992)

  55. Chowdhury, M.S.H., Hashim, I.: Solution of a class of singular second-order IVPs by Homotopy–Perturbation method. Phys. Lett. A 365, 439–447 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  56. Darani, M.A., Nasiri, M.: A fractional type of the Chebyshev polynomials for approximation of solution of linear fractional differential equations. Comp. Meth. Differ. Equ. 1, 96–107 (2013)

    MATH  Google Scholar 

  57. Butcher, E.A., Ma, H., Bueler, E., Averina, V., Szabo, Z.: Stability of linear time-periodic delay-differential equations via Chebyshev polynomials. Int. J. Numer. Methods. Eng. 59, 895–922 (2004)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

The authors are very grateful to reviewers and editor for carefully reading the paper and for their comments and suggestions which have improved the paper.

Author information

Authors and Affiliations

  1. Department of Computer Sciences, Shahid Beheshti University, G.C., Tehran, Iran

    Kourosh Parand & Mehdi Delkhosh

  2. Department of Cognitive Modelling, Institute for Cognitive and Brain Sciences, Shahid Beheshti University, G.C., Tehran, Iran

    Kourosh Parand

Authors
  1. Kourosh Parand
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mehdi Delkhosh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kourosh Parand.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Parand, K., Delkhosh, M. Solving Volterra’s population growth model of arbitrary order using the generalized fractional order of the Chebyshev functions. Ricerche mat. 65, 307–328 (2016). https://doi.org/10.1007/s11587-016-0291-y

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11587-016-0291-y

Keywords

Mathematics Subject Classification

Search

Navigation