Mobile-to-mobile fading channels in amplify-and-forward relay systems under line-of-sight conditions: statistical modeling and analysis

Abstract

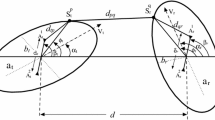
This paper deals with the modeling and analysis of narrowband mobile-to-mobile (M2M) fading channels for amplify-and-forward relay links under line-of-sight (LOS) conditions. It is assumed that a LOS component exists in the direct link between the source mobile station (SMS) and the destination mobile station (DMS), as well as in the links via the mobile relay (MR). The proposed channel model is referred to as the multiple-LOS second-order scattering (MLSS) channel model. The MLSS channel model is derived from a second-order scattering process, where the received signal is modeled in the complex baseband as the sum of a single and a double scattered component. Analytical expressions are derived for the mean value, variance, probability density function (PDF), cumulative distribution function (CDF), level-crossing rate (LCR), and average duration of fades (ADF) of the received envelope of MLSS channels. The PDF of the channel phase is also investigated. It is observed that the LOS components and the relay gain have a significant influence on the statistics of MLSS channels. It is also shown that MLSS channels include various other channel models as special cases, e.g., double Rayleigh channels, double Rice channels, single-LOS double-scattering (SLDS) channels, non-line-of-sight (NLOS) second-order scattering (NLSS) channels, and single-LOS second-order scattering (SLSS) channels. The correctness of all analytical results is confirmed by simulations using a high performance channel simulator. Our novel MLSS channel model is of significant importance for the system level performance evaluation of M2M communication systems in different M2M propagation scenarios. Furthermore, our studies pertaining to the fading behavior of MLSS channels are useful for the design and development of relay-based cooperative wireless networks.

Appendices

Appendix A: Proof of (30)

For ρ ₁ = 0, $A_{\mbox{\tiny MR}}=1$, and $\sigma^2_1\rightarrow0$, (15) reduces to

$$\label{eqnpdfEnvelope2RiceDerivationA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_1=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=&\frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}\int\limits^{\infty}_{0} \int\limits^{\pi}_{-\pi}d\psi d\nu d\omega\\ &&\times \frac{\omega}{\nu} e^{-\frac{\left(\omega/\nu\right)^2+\rho_{2}^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2+\rho_{\tiny A_{\mbox{\tiny MR}}}^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}} I_{0}\left(\sqrt{g_{5} \left(\omega,\nu,\psi\right)}\right) \\ &&\times \underbrace{\lim\limits_{\sigma^2_1\rightarrow0} \frac{e^{-\frac{x^2+\omega^2}{2\sigma_{1}^2}}}{\sigma_{1}^2}I_{0}\left( \frac{x\omega}{\sigma_{1}^2}\right)}_{X_1} \end{array} $$

(54)

for x ≥ 0. Furthermore, using the asymptotic expansions of the zeroth-order modified Bessel function of the first kind $I_{0}\left(\cdot\right)$ [49, Sec. (7.23), Eq. (2)], allows us to write X ₁ as

$$\label{eqnsolution4}\begin{array}{lll} X_1&=&\lim\limits_{\sigma^2_1\rightarrow0} \frac{e^{-\frac{x^2+\omega^2}{2\sigma_{1}^2}}}{\sigma_{1}^2} \frac{e^{\frac{x\omega}{\sigma^2_1}}\sigma_1}{\sqrt{2\pi x\omega}} =\frac{1}{\sqrt{x\omega}} \lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{x^2+\omega^2-2x\omega}{2\sigma_{1}^2}}}{\sqrt{2\pi}\sigma_1}\\ &=&\frac{1}{\sqrt{x\omega}}\delta\left(\omega-x\right)\:. \end{array} $$

(55)

Substituting (55) in (54) gives

$$\label{eqnpdfEnvelope2RiceDerivationB}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_1=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=&\frac{x}{2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\nu\,\frac{1}{\nu}e^{-\frac{\nu^2+\rho_{\tiny A_{\mbox{\tiny MR}}}^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}} \int\limits^{\pi}_{-\pi}d\psi \int\limits^{\infty}_{0}d\omega\\ &&\times \sqrt{\frac{\omega}{x}} e^{-\frac{\left(\omega/\nu\right)^2+\rho_{2}^2}{2\sigma_{2}^2}} I_{0}\left(\sqrt{g_{5} \left(\omega,\nu,\psi\right)}\right) \delta\left(\omega-x\right)\\ \end{array} $$

(56)

for x ≥ 0. Applying the sifting property of the delta function [50] on (56) gives

$$\label{eqnpdfEnvelope2RiceDerivationC}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_1=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=&\frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\nu\,\frac{1}{\nu} e^{-\frac{\left(x/\nu\right)^2+\rho_{2}^2}{2\sigma_{2}^2}}e^{-\frac{\nu^2+\rho_{\tiny A_{\mbox{\tiny MR}}}^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}} \\&&\times \underbrace{\int\limits^{\pi}_{-\pi} I_{0}\left(\sqrt{g_{5} \left(x,\nu,\psi\right)}\right)d\psi}_{X_2},\,x\geq0. \end{array} $$

(57)

Numerical investigations show that it is possible to approximate X ₂ in (57) as

$$ X_2=\left(2\pi\right)I_0\left(\frac{x\rho_2}{\nu\sigma^2_2}\right)I_0\left(\frac{\nu\rho_{\tiny A_{\mbox{\tiny MR}}}}{\sigma^2_{\tiny A_{\mbox{\tiny MR}}}}\right). \label{eqn:solutionX5} $$

(58)

Thus, replacing (58) in (57) gives (30).

Appendix B: Proof of (35)

Substituting ρ ₁ = ρ ₂ = ρ ₃ = 0 and $A_{\mbox{\tiny MR}}=1$ in (15), we can express $p_{\Xi}\left(x\right)\left|_{\begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.$ as follows

$$\label{eqn:pdfEnvelope2Rayleigh_DerivationA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.&=& \frac{x\;e^{-\frac{x^2}{2\sigma_{1}^2}}} {2\pi\sigma_{1}^2\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega\,\omega\,e^{-\frac{\omega^2}{2\sigma_{1}^2}}I_{0}\left(\frac{x\omega}{\sigma_{1}^2}\right) \\ &&\times \underbrace{\int\limits^{\infty}_{0}\frac{1}{\nu}e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\,dv}_{X_3=K_0\left(\frac{w}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)}\underbrace{\int\limits^{\pi}_{-\pi}d\psi}_{X_4=2\pi},\,x\geq0\\ \end{array} $$

(59)

where X ₃ is obtained by using [31, Eq. (3.478.4)]. Taking the limit $\sigma^2_1\rightarrow0$, (59) can be written as

$$\label{eqn:pdfEnvelope2Rayleigh_DerivationB}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=& \frac{x} {\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega\,\omega K_{0}\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)\\ &&\times \lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{x^2+\omega^2}{2\sigma_{1}^2}}}{\sigma_{1}^2} I_{0}\left(\frac{\omega}{\sigma_{1}^2}x\right),\;x\geq0\,. \end{array} $$

(60)

Using the asymptotic expansions of the zeroth-order modified Bessel function of the first kind $I_{0}\left(\cdot\right)$ [49, Sec. (7.23), Eq. (2)], (60) can be expressed as

$$\label{eqn:pdfEnvelope2Rayleigh_DerivationC}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=& \frac{x} {\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega\,\omega K_{0}\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)\\ &&\times \lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{x^2+\omega^2}{2\sigma_{1}^2}}}{\sigma_{1}^2} \frac{e^{\frac{x\omega}{\sigma_{1}^2}}\sigma_1}{\sqrt{2\pi x\:\omega}}\\ &=& \frac{x} {\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega\,\sqrt{\frac{\omega}{x}} K_{0}\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)\\ &&\times \underbrace{\lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{x^2+\omega^2-2x\omega}{2\sigma_{1}^2}}} {\sqrt{2\pi}\sigma_1}}_{X_5},\;x\geq0 \end{array} $$

(61)

where $X_5=\delta\left(\omega-x\right)$ by definition of the delta function [51]. Thus, (61) can be written as

$$\label{eqn:pdfEnvelope2Rayleigh_DerivationD}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=& \frac{x} {\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega \sqrt{\frac{\omega}{x}} K_{0}\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right) \\ &&\times \delta\left(\omega-x\right),x\geq0\,. \end{array} $$

(62)

Applying the sifting property of the delta function [50] on (62), results in (35).

Appendix C: Proof of (40)

Substituting ρ ₂ = ρ ₃ = 0 as well as $A_{\mbox{\tiny MR}}=1$ and integrating (12) over θ from − π to π allows us to write $p_{\Xi}\left(x\right)\left|_{\begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.$ as

$$\label{eqn:pdfSLDS_DerivationAA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.&=& \frac{x}{\left(2\pi\right)^2\sigma^2_2\sigma^2_{\tiny A_{\mbox{\tiny MR}}}} \int\limits^{\infty}_{0}\int\limits^{\pi}_{-\pi}d\theta\,d\omega\\ &&\times \ \underbrace{\int\limits^{\infty}_{0}d\nu\frac{\omega}{\nu} e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma^2_2}}e^{-\frac{\nu^2}{2\sigma^2_{\tiny A_{\mbox{\tiny MR}}}}}} _{X_6=K_0\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)}e^{-\frac{g_{11}\left(x,\theta\right)}{2\sigma^2_1}}\\ &&\times \ \underbrace{\int\limits^{\pi}_{-\pi} e^{-\frac{\omega\left\{\rho_1\cos\left(\theta\right)+x\cos\left(\theta-\psi\right)\right\}}{\sigma^2_1}}d\psi} _{X_7=(2\pi)I_0\left(\frac{\omega}{\sigma^2_1}\sqrt{g_{11}\left(x,\theta\right)}\right)} \end{array} $$

(63)

for x ≥ 0. In (63), X ₆ is evaluated using [31, Eq. (3.478.4)], whereas X ₇ with the help of [31, Eq. (3.338.4)]. Taking the limit $\sigma^2_1\rightarrow0$ in (63), allows us to write

$$\label{eqn:pdfSLDS_DerivationA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.\!&=&\!\frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}\int\limits^{\infty}_{0} \int\limits^{\pi}_{-\pi}d\theta d\nu d\omega\frac{\omega}{\nu} e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\\ &&\times \ \lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{\omega^2}{2\sigma_{1}^2}}e^{-\frac{g_{11}\left(x,\theta\right)} {2\sigma_{1}^2}}}{\sigma_{1}^2} I_{0}\left( \frac{\omega}{\sigma_{1}^2}\sqrt{g_{11} \left(x,\theta\right)}\right)\\ \end{array} $$

(64)

for x ≥ 0. Using the asymptotic expansions of the zeroth-order modified Bessel function of the first kind $I_{0}\left(\cdot\right)$ [49, Sec. (7.23), Eq. (2)], (64) can be expressed as

$$\label{eqn:pdfSLDS_DerivationB}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.\!&=&\!\frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}\int\limits^{\infty}_{0} \int\limits^{\pi}_{-\pi}d\theta d\nu d\omega\frac{\omega}{\nu} e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\\ &&\times \ \lim\limits_{\sigma^2_1\rightarrow0}\frac{e^{-\frac{\omega^2}{2\sigma_{1}^2}}e^{-\frac{g_{11}\left(x,\theta\right)} {2\sigma_{1}^2}}}{\sigma_{1}^2} \frac{e^{\frac{\omega}{\sigma_{1}^2}\sqrt{g_{11} \left(x,\theta\right)}}\sigma_1}{\sqrt{2\pi \omega}g_{11}\left(x,\theta\right)}\\ &=& \frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}\int\limits^{\infty}_{0} \int\limits^{\pi}_{-\pi}d\theta d\nu d\omega \\ &&\times \frac{\sqrt{\omega}}{\nu\,g_{11} \left(x,\theta\right)} e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\\ &&\times \underbrace{\lim\limits_{\sigma^2_1\rightarrow0} \frac{e^{-\frac{\omega^2+\left(\sqrt{g_{11}\left(x,\theta\right)}\right)^2 -2\omega\sqrt{g_{11}\left(x,\theta\right)}} {2\sigma_{1}^2}}}{\sqrt{2\pi}\sigma_{1}}}_{X_8} \end{array} $$

(65)

for x ≥ 0. In (65), $X_8=\delta\left(\omega-g_{11}\left(x,\theta\right)\right)$ by definition of the delta function [51]. Thus, (65) can be written as

$$\label{eqn:pdfSLDS_DerivationC}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=&\frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}\int\limits^{\infty}_{0} \int\limits^{\pi}_{-\pi}d\theta d\nu d\omega\frac{\sqrt{\omega}}{\nu\,g_{11} \left(x,\theta\right)}\\ &&\times \ e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\delta\left(\omega-\sqrt{g_{11} \left(x,\theta\right)}\right) \\ \end{array} $$

(66)

for x ≥ 0. Applying the sifting property of the delta function [50] on (67) results in

$$\label{eqn:pdfSLDS_DerivationC}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1\\ \sigma^2_1\rightarrow0 \end{subarray}}\right.&=& \frac{x} {2\pi\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \\ && \times \int\limits^{\pi}_{-\pi}d\theta \underbrace{\int\limits^{\infty}_{0} \frac{e^{-\frac{g^2_{11} \left(x,\theta\right)}{2\sigma_{2}^2v^2}}e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}}{\nu}\,d\nu}_{X_9=K_0\left(\frac{\sqrt{g_{11}\left(x,\theta\right)}}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)},\,x\geq0\:.\\ \end{array} $$

(67)

where X ₉ is evaluated using [31, Eq. (3.478.4)]. Thus, integrating (67) over θ from − π to π results in the final expression given in (40).

Appendix D: Proof of (46)

Substituting ρ ₂ = ρ ₃ = 0 as well as $A_{\mbox{\tiny MR}}=1$ and integrating (12) over θ from − π to π allows us to write $p_{\Xi}\left(x\right)\left|_{\begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.$ as

$$\label{eqn:pdfSLSS_DerivationA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.&=& \frac{x}{\left(2\pi\right)^2\sigma^2_2\sigma^2_{\tiny A_{\mbox{\tiny MR}}}} \int\limits^{\infty}_{0}\int\limits^{\pi}_{-\pi}d\theta\,d\omega\\ &&\times \ \underbrace{\int\limits^{\infty}_{0}d\nu\frac{\omega}{\nu} e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma^2_2}}e^{-\frac{\nu^2}{2\sigma^2_{\tiny A_{\mbox{\tiny MR}}}}}} _{X_{10}=K_0\left(\frac{\omega}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)}e^{-\frac{g_{11}\left(x,\theta\right)}{2\sigma^2_1}} \\ &&\times \ \underbrace{\int\limits^{\pi}_{-\pi} e^{-\frac{\omega\left\{\rho_1\cos\left(\theta\right)+x\cos\left(\theta-\psi\right)\right\}}{\sigma^2_1}}d\psi} _{X_{11}=(2\pi)I_0\left(\frac{\omega}{\sigma^2_1}\sqrt{g_{11}\left(x,\theta\right)}\right)} \end{array} $$

(68)

for x ≥ 0. In (68), X ₁₀ and X ₁₁ are evaluated using [31, Eq. (3.478.4)] and [31, Eq. (3.338.4)], respectively. Thus, replacing X ₁₀ and X ₁₁ in (68) results in (46).

Appendix E: Proof of (51)

Substituting ρ ₁ = ρ ₂ = ρ ₃ = 0 and $A_{\mbox{\tiny MR}}=1$ in (15), we can express $p_{\Xi}\left(x\right)\left|_{\begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.$ as follows

$$\label{eqn:pdfNLSS_DerivationA}\begin{array}{lll} p_{\Xi}\left(x\right)\left|_{ \begin{subarray}{l} \rho1,\rho_2,\rho_3=0\\ A_{\mbox{\tiny MR}}=1 \end{subarray}}\right.&=& \frac{x\;e^{-\frac{x^2}{2\sigma_{1}^2}}} {2\pi\sigma_{1}^2\sigma_{2}^2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2} \int\limits^{\infty}_{0}d\omega\,\omega\,e^{-\frac{\omega^2}{2\sigma_{1}^2}} I_{0}\left(\frac{x\omega}{\sigma_{1}^2}\right)\\ &&\times \ \underbrace{\int\limits^{\infty}_{0}\frac{1}{\nu}e^{-\frac{\left(\omega/\nu\right)^2}{2\sigma_{2}^2}} e^{-\frac{\nu^2}{2\sigma_{\tiny A_{\mbox{\tiny MR}}}^2}}\,dv}_{X_{12}=K_0\left(\frac{w}{\sigma_2\sigma_{\tiny A_{\mbox{\tiny MR}}}}\right)}\underbrace{\int\limits^{\pi}_{-\pi}d\psi}_{X_{13}=2\pi},\,x\geq0 \\ \end{array} $$

(69)

where X ₁₂ is evaluated using [31, Eq. (3.478.4)]. Thus, replacing X ₁₂ and X ₁₃ in (69) gives (51).