Magnetization of High Density Hadronic Fluid

Abstract

In the present paper the magnetization of a high density relativistic fluid of elementary particles is studied. At very high densities, such as may be found in the interior of a neutron star, when the external magnetic field is gradually increased, the energy of the normal phase of the fluid remains practically constant before extremely high magnetic fields are reached. However, if pion condensation occurs, the energy decreases linearly while the magnetic field strength increases, so that a non vanishing magnetization, independent of the magnetic field, is present. The expression of the magnetization is derived by first considering and solving the Dirac equation of a fermion in interaction with a magnetic field and with a chiral sigma-pion pair. The solution provides the energies of single-particle states. The energy of the system is found by summing up contributions from all particles in the particle fluid. For nuclear densities above 2 to 3ρ ₀, where ρ ₀ is the equilibrium nuclear density, the resulting magnetic field turns out to be rather huge, of the order of 10¹⁷ Gauss.

Appendices

Appendix A

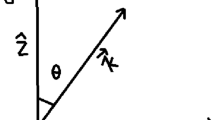
The eigenfunctions associated with (2) read

$$ \begin{array}{rll} &&\phi_1=|\nu\rangle,\quad\phi_2=-{\delta\sqrt{\nu}\over\alpha_-+\beta(\nu-1)}|\nu-1\rangle,\quad \nu\neq0,\\ &&\psi_1={1\over M}\left({1\over2}|q|-k_z+E-{\delta\sqrt{2QB}\nu\over\alpha_-+\beta(\nu-1)}\right)|\nu\rangle,\\ && \psi_2={1\over M}\left(\sqrt{2QB}-{({1\over2}|q|+k_z+E)\delta\over\alpha_-+\beta(\nu-1)}\right)\sqrt{\nu}|\nu-1\rangle. \end{array} $$

We wish to investigate the spin of the lowest branch of positive energy states, $E_-^{(+)}=\sqrt{\left({1\over2}|q|-\sqrt{k_z^2+M^2}\right)^2+2 Q B\nu}$. We may write

$$ \phi_2={\sqrt{ 2|q|^2QB\nu}\over-({1\over2}|q|-E)^2+k_z^2+M^2+2QB\nu}|\nu-1\rangle. $$

The contribution of ψ ₁, ψ ₂ to the average spin is the same as the contribution of ϕ ₁, ϕ ₂,

$$ \overline{\Sigma}(k_z,\nu)={\langle\phi_1|\phi_1\rangle-\langle\phi_2|\phi_2\rangle\over \langle\phi_1|\phi_1\rangle+\langle\phi_2|\phi_2\rangle} ={\langle\psi_1|\psi_1\rangle-\langle\psi_2|\psi_2\rangle\over \langle\psi_1|\psi_1\rangle+\langle\psi_2|\psi_2\rangle}. $$

We find that the average spin reads,

$$ \overline{\Sigma}(k_z,\nu)={\sqrt{k_z^2+M^2}-{1\over2}|q|\over\sqrt{(\sqrt{k_z^2+M^2}-{1\over2}|q|)^2+2QB}}, $$

so that

$$ \begin{array}{rll} &&{L^2|QB|\over(2\pi)}\sum\limits_{k_z\nu}\overline\Sigma(k_z,\nu)\\ &&\approx{L^3\over(2\pi)^2}~{1\over6}\left(\left(2\mu^2-|q|^2-8M^2\right.\right.\\ &&\left.\left.\quad-|q|\mu\right) \sqrt{({1\over2}|q|+\mu)^2-M^2} +6M^2( |q|+\mu)\right.\\ &&\quad\times\left.\left(\log\left({{1\over2}|q|+\mu+\sqrt{\left({1\over2}|q|+\mu\right)^2-M^2}}\right)-\log M\right) \right). \end{array} $$

Although the eigenfunctions of the Dirac hamiltonian are not eigenfunctions of spin (except for the lowest Landau level), the average spin is determined by the components ϕ ₁, ϕ ₂. We might expect that the magnetization would be obtained multiplying the previous expression by Q/( 2M), but that is not really correct since it does not lead to agreement with (16).

Appendix B

Derivation of the formula

$$ \begin{array}{rll} a\sum\limits_{\nu=1}^Nf(a\nu)&\approx&\int_{x=0}^{Na}{\rm d} xf(x)+{1\over2}a(f(Na)-f(0))\\ &&+{1\over12}a^2(f'(Na)-f'(0))+\cdots. \end{array} $$

For a(ν − 1) < x ≤ aν, expand f(x) = f(aν) + (x − aν)f′(aν) + ⋯ and integrate in the interval [a(ν − 1), aν],

$$ \begin{array}{rll} \int_{x=a(\nu-1)}^{a\nu}{\rm d} xf(x)&=&[x-a\nu]_{x=a(\nu-1)}^{x=a\nu}f(a\nu)\\ &&+{1\over2}[(x-a\nu)^2]_{x=a(\nu-1)}^{x=a\nu}f'(a\nu)+\cdots\\ &=&af(a\nu)-{1\over2}a^2f'(a\nu)+{1\over6}a^3f''(a\nu)+\cdots. \end{array} $$

Thus,

$$ \begin{array}{rll} \sum\limits_{\nu=1}^N\int_{x=a(\nu-1)}^{a\nu}{\rm d} xf(x)&=&\int_{x=0}^{aN}{\rm d} xf(x) =a\sum\limits_{\nu=1}^Nf(a\nu)\\ &&-{1\over2}a^2\sum\limits_{\nu=1}^Nf'(a\nu)+\cdots, \end{array} $$

implying

$$ \begin{array}{rll} a\sum\limits_{\nu=1}^Nf(a\nu)&=&\int_{x=0}^{aN}{\rm d} xf(x)+{1\over2}a^2\sum\limits_{\nu=1}^Nf'(a\nu)\\ &&-{1\over6}a^3\sum\limits_{\nu=1}^Nf''(a\nu)+\cdots\\ &\approx&\int_{x=0}^{aN}{\rm d} x f(x)+ {1\over2}a \int_{x=0}^{aN}{\rm d} x f'(x)\\ &&+\left({1\over4}-{1\over6}\right)a^2 \int_{x=0}^{aN}{\rm d} x f''(x)+\cdots\\ &=&\int_{x=0}^{aN}{\rm d} x f(x)+ {1\over2}a (f(Na)-f(0))\\ &&+{1\over12}a^2 (f'(Na)-f'(0))+\cdots. \end{array} $$