Efficacy of Semi-active Absorber for Controlling Self-excited Vibration

Abstract

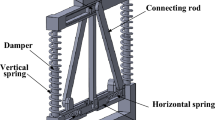
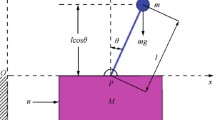
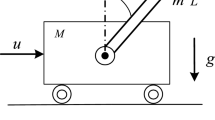
It is shown that a passive vibration absorber can completely quench the self-excited vibration only for certain parameter values, like the strength of instability in the primary system. The present paper numerically explores the performance of semi-active vibration absorber in controlling self-excited vibration. In the proposed semi-active scheme, the damping force of the absorber is switched between the maximum and the minimum values according to certain control logics. Four different control strategies are considered—these are on–off velocity-based ground-hook control (VBG), on–off displacement-based ground-hook control (DBG), continuous VBG and continuous DBG. Numerical simulations are performed in the MATLAB Simulink to explore the efficacy of the control strategies. It is shown that the on–off DBG control is superior to all other control strategies.

Appendix 1

Averaging is employed to system equations (Eqs. (1) and (2)). To this end, the following similarity transformation is defined:

$$\left\{ {\begin{array}{*{20}c} {y_{\text{p}} } \\ {y_{\text{a}} } \\ \end{array} } \right\} = \varPhi \left\{ {\begin{array}{*{20}c} {z_{1} } \\ {z_{2} } \\ \end{array} } \right\},$$

(22)

where $\varPhi = \left[ {\begin{array}{*{20}c} 1 & 1 \\ {\rho_{1} } & {\rho_{2} } \\ \end{array} } \right]$ with $\rho_{i} = \frac{{1 + \mu \varOmega_{\text{a}}^{2} + \omega_{i}^{2} }}{{\mu \varOmega_{\text{a}}^{2} }}$ and

$$\omega_{i} = \left[ {\frac{{(1 + \mu \varOmega_{\text{a}}^{2} + \varOmega_{\text{a}}^{2} ) \pm \sqrt {(1 + \mu \varOmega_{\text{a}}^{2} + \varOmega_{\text{a}}^{2} )^{2} - 4\varOmega_{\text{a}}^{2} } }}{2}} \right]^{1/2}$$

Using the transformation defined in Eq. (16), Eqs. (3) and (4) are transformed to

$$\ddot{z}_{i} + \omega^{2} z_{i} = f_{i} ,\quad i{ = 1,}\; 2$$

(23)

where

$$f_{1} = (\omega^{2} - \omega_{1}^{2} )z_{1} - (c_{11} \dot{z}_{1} + c_{12} \dot{z}_{2} ) - \frac{{\rho_{2} e_{3} }}{{\rho_{2} - \rho_{1} }}(\dot{z}_{1} + \dot{z}_{2} )^{3}$$

and

$$f_{2} = (\omega^{2} - \omega_{2}^{2} )z_{2} - (c_{21} \dot{z}_{1} + c_{22} \dot{z}_{2} ) + \frac{{\rho_{1} e_{3} }}{{\rho_{2} - \rho_{1} }}(\dot{z}_{1} + \dot{z}_{2} )^{3}$$

with

$$C_{11} = \frac{{\rho_{2} (2\mu \xi_{\text{a}} \varOmega_{\text{a}} + h - 2\mu \xi_{\text{a}} \varOmega_{\text{a}} \rho_{1} ) - ( - 2\xi_{\text{a}} \varOmega_{\text{a}} + 2\xi_{\text{a}} \varOmega_{\text{a}} \rho_{1} )}}{{(\rho_{2} - \rho_{1} )}},$$

$$C_{12} = \frac{{(\rho_{2} (2\mu \xi_{\text{a}} \varOmega_{\text{a}} + h - 2\mu \xi_{\text{a}} \varOmega_{\text{a}} \rho_{2} ) - ( - 2\xi_{\text{a}} \varOmega_{\text{a}} + 2\xi_{\text{a}} \varOmega_{\text{a}} \rho_{2} )}}{{(\rho_{2} - \rho_{1} )}},$$

$$C_{21} = \frac{{( - \rho_{1} (2\mu \xi_{\text{a}} \varOmega_{\text{a}} + h - 2\mu \xi_{\text{a}} \varOmega_{\text{a}} \rho_{1} ) + ( - 2\xi_{\text{a}} \varOmega_{\text{a}} + 2\xi_{\text{a}} \varOmega_{\text{a}} \rho_{1} )}}{{(\rho_{2} - \rho_{1} )}},$$

and

$$C_{22} = \frac{{ - \rho_{1} (2\mu \xi_{\text{a}} \varOmega_{\text{a}} + h - 2\mu \xi_{\text{a}} \varOmega_{\text{a}} \rho_{2} ) + ( - 2\xi_{\text{a}} \varOmega_{\text{a}} + 2\xi_{\text{a}} \varOmega_{\text{a}} \rho_{2} )}}{{(\rho_{2} - \rho_{1} )}}.$$

Assume the solutions of Eq. (23) as

$$z_{i} (\tau ) = A_{i} (\tau )\cos (\omega \tau + \theta_{i} (\tau ))$$

(24)

where A_i and θ_i are slowly varying functions of time and ω is the frequency of oscillation of the system.

Assume

$$f_{i} = \varepsilon \tilde{f}_{i} ,\quad \varepsilon { < < 1,}$$

(25)

One finally obtains the slow-flow equations as

$$\dot{A}_{i} = - \frac{\varepsilon }{2\pi }\int_{0}^{{{\raise0.7ex\hbox{${2\pi }$} \!\mathord{\left/ {\vphantom {{2\pi } \omega }}\right.\kern-0pt} \!\lower0.7ex\hbox{$\omega $}}}} {\tilde{f}_{i} \sin (\omega \tau + \theta_{i} )} {\text{d}}\tau$$

(26)

and

$$A_{i} \dot{\theta }_{i} = - \frac{\varepsilon }{2\pi }\int_{0}^{{{\raise0.7ex\hbox{${2\pi }$} \!\mathord{\left/ {\vphantom {{2\pi } \omega }}\right.\kern-0pt} \!\lower0.7ex\hbox{$\omega $}}}} {\tilde{f}_{i} \cos (\omega \tau + \theta_{i} )} {\text{d}}\tau$$

(27)

Equations (26) and (27) can be finally recast as

$$\dot{A}_{1} = \varphi_{1} (A_{1} ,A_{2} ,\theta ,\omega ),$$

(28)

$$\dot{A}_{2} = \varphi_{2} (A_{1} ,A_{2} ,\theta ,\omega ),$$

(29)

$$\dot{\theta }_{1} = \varphi_{3} (A_{1} ,A_{2} ,\theta ,\omega \dot{)},$$

(30)

$$\dot{\theta }_{2} = \varphi_{4} (A_{1} ,A_{2} ,\theta ,\omega ),$$

(31)

The steady-state solutions of Eqs. (28)–(31) can be obtained by solving the following equations:

$$\varphi_{i} (A_{1} ,A_{2} ,\theta ,\omega ) = 0,\quad i{\text{ = 1 to 4}}$$

(32)

Solving Eqs. (1) and (2), one obtains A₁, A₂, θ and ω. Now transforming back to the physical coordinates, one obtains the amplitude of oscillation of the primary mass and absorber mass, respectively, as

$$A_{\text{p}} = \sqrt {A_{1}^{2} + A_{2}^{2} + 2A_{1} A_{2} \cos \theta }$$

(33)

and

$$A_{\text{a}} = \sqrt {\rho_{1}^{2} A_{1}^{2} + \rho_{2}^{2} A_{2}^{2} + 2A_{1} A_{2} \rho_{1} \rho_{2} \cos \theta }$$

(34)