Analytical solution of the electro-mechanical flexural coupling between piezoelectric actuators and flexible-spring boundary structure in smart composite plates

Abstract

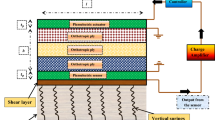
An analytical solution has been developed developed in this research for electro-mechanical flexural response of smart laminated piezoelectric composite rectangular plates encompassing flexible-spring boundary conditions at two opposite edges. Flexible-spring boundary structure is introduced to the system by inclusion of rotational springs of adjustable stiffness which can vary depending on changes in the rotational fixity factor of the springs. To add to the case study complexity, the two other edges are kept free. Three advantages of employing the proposed analytical method include: (1) the electro-mechanical flexural coupling between the piezoelectric actuators and the plate’s rotational springs of adjustable stiffness is addressed; (2) there is no need for trial deformation and characteristic function—therefore, it has higher accuracy than conventional semi-inverse methods; (3) there is no restriction imposed to the position, type, and number of applied loads. The Linear Theory of Piezoelectricity and Classical Plate Theory are adopted to derive the exact elasticity equation. The higher-order Fourier integral and higher-order unit step function differential equations are combined to derive the analytical equations. The analytical results are validated against those obtained from Abaqus Finite Element (FE) package. The results comparison showed good agreement. The proposed smart plates can potentially be applied to real-life structural systems such as smart floors and bridges and the proposed analytical solution can be used to analyze the flexural deformation response.

Appendices

Appendices

1.1 Appendix A

The relation between the global and local stresses in a composite layer is stated in Eq. 32. The transformation matrix [T] is calculated using Eq. 33 [44]:

$$\left[ {\begin{array}{*{20}c} {\sigma_{xx} } & {\sigma_{yy} } & {\tau_{xy} } \\ \end{array} } \right]_{k}^{T} = \left[ T \right]^{ - 1} \left[ {\begin{array}{*{20}c} {\sigma_{11} } & {\sigma_{22} } & {\tau_{12} } \\ \end{array} } \right]_{k}^{T}$$

(32)

where:

$$\left[ T \right] = \left[ {\begin{array}{lll} {c^{2} } & \quad {s^{2} } & \quad {2{\text{c}} s} \\ {s^{2} } & \quad {c^{2} } & \quad { - 2cs} \\ { - cs} & \quad {cs} & \quad {\left( {c^{2} - s^{2} } \right)} \\ \end{array} } \right]$$

(33)

where, c and s stand for cosine and sine of function β and β is the fiber angle of each composite layer.

The terms Q_ij present in Eqs. 3a, b stand for the elastic stiffness in composite and piezoelectric layers as stated in Eqs. 34–37 [45], i.e.

$$Q_{11} = \frac{{E_{11} }}{{1 - \nu_{12} \nu_{21} }}$$

(34)

$$Q_{22} = \frac{{E_{22} }}{{1 - \nu_{12} \nu_{21} }}$$

(35)

$$Q_{12} = \frac{{\nu_{12} E_{22} }}{{1 - \nu_{12} \nu_{21} }}$$

(36)

$$Q_{66} = G_{12}$$

(37)

where, E₁₁, E₂₂ are elastic modules along and perpendicular to fibers, respectively and v₁₂, and G₁₂ are the Poisson’s ratio and shear modules, respectively. The global stresses-strains in a composite layer is calculated using Eq. 38 [46]:

$$\left[ {\begin{array}{*{20}c} {\sigma_{xx} } \\ {\sigma_{yy} } \\ {\tau_{xy} } \\ \end{array} } \right]^{k} = \left[ {\begin{array}{lll} {\overline{Q}_{11} } & \quad{\overline{Q}_{12} } & \quad {\overline{Q}_{16} } \\ {\overline{Q}_{12} } & \quad {\overline{Q}_{22} } &\quad {\overline{Q}_{26} } \\ {\overline{Q}_{16} } &\quad {\overline{Q}_{26} } & \quad {\overline{Q}_{66} } \\ \end{array} } \right]^{k} \left[ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\varepsilon_{yy} } \\ {\gamma_{xy} } \\ \end{array} } \right]^{k}$$

(38)

where, σ_xx, σ_yy, and τ_xy are the global stress and strain components in the x and y directions, respectively. $\overline{Q}$ _ij^k in a composite layer are the transformed stiffness matrix terms which are calculated using Eqs. 39–44 [46]:

$$\overline{Q}_{11} = Q_{11} c^{4} + 2(Q_{12} + 2Q_{66} )c^{2} s^{2} + Q_{22} s$$

(39)

$$\overline{Q}_{12} = (Q_{11} + Q_{22} - 4Q_{66} )c^{2} s^{2} + Q_{12} (c^{4} + s^{4} )$$

(40)

$$\overline{Q}_{22} = Q_{11} s^{4} + 2(Q_{12} + 2Q_{66} )c^{2} s^{2} + Q_{22} c^{4}$$

(41)

$$\overline{Q}_{16} = - Q_{22} cs^{3} + Q_{11} c^{3} s - (Q_{12} + 2Q_{66} )(c^{2} - s^{2} )cs$$

(42)

$$\overline{Q}_{26} = - Q_{22} c^{3} s + Q_{11} cs^{3} - (Q_{12} + 2Q_{66} )(c^{2} - s^{2} )cs$$

(43)

$$\overline{Q}_{66} = (Q_{11} + Q_{22} - 2Q_{12} )c^{2} s^{2} + Q_{66} (c^{2} - s^{2} )^{2}$$

(44)

The piezoelectric modules in a piezoelectric layer are calculated using Eqs. 45–47 [24, 47]:

$$e_{31} = Q_{11} d_{31} + Q_{12} d_{32}$$

(45)

$$e_{32} = Q_{12} d_{31} + Q_{22} d_{32}$$

(46)

$$e_{36} = 0$$

(47)

where, d_ij stand for the piezoelectric dielectric constants under constant stress in a piezoelectric layer.

The electro-mechanical bending-twisting couplings are calculated using Eq. 48 [28]:

$$\left[ {\begin{array}{*{20}c} {M_{xx} } & {M_{yy} } & {M_{xy} } \\ \end{array} } \right]^{T} = \int\limits_{ - H/2}^{H/2} z \left( {\sigma_{xx} ,\sigma_{yy} ,\tau_{xy} } \right)dz - \left[ {\begin{array}{*{20}c} {M_{xx}^{P} } & {M_{yy}^{P} } & {M_{xy}^{P} } \\ \end{array} } \right]^{T}$$

(48)

where, [M_xx]^P and [M_yy]^P are defined as the bending moments and [M_xy]^P is the twisting moment induced by electrical load, respectively [48].

1.2 Appendix B

The twenty coefficients (Sⁱ_mn), i = {1,2,…,20} in four finite systems of the linear equations (Eqs. 31a–d) are as follows:

$$S_{mn}^{1} = \delta_{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {\frac{{\alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }} - 2H\alpha_{m}^{2} - D_{22} \beta_{n}^{2} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}} + 1} \right]$$

(49)

$$S_{mn}^{2} = \delta_{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {2H\alpha_{m}^{2} ( - 1)^{n} + D_{22} ( - 1)^{n} \beta_{n}^{2} - \frac{{( - 1)^{n} \alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}} - ( - 1)^{n} } \right]$$

(50)

$$S_{mn}^{3} = \delta_{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( { - D_{11} \alpha_{m} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}}} \right]$$

(51)

$$S_{mn}^{4} = \delta_{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {D_{11} \alpha_{m} ( - 1)^{m} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}}} \right]$$

(52)

$$\begin{aligned} S_{mn}^{5} & = - \delta_{n} \left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left \{ {\sum\limits_{L = 1}^{Mn} {C_{mn}^{1} \left( {\frac{{ - P_{o} }}{{\alpha_{m} \beta_{n} }}} \right)_{{}} } \left[ {\cos (\alpha_{m} x_{1M} )} \right. - \left. {\cos (\alpha_{m} x_{2M} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1M} ) - \sin (\beta_{n} y_{2M} )} \right]_{L} } \right. \hfill \\ & \quad + \sum\limits_{L = 1}^{Tn} {C_{mn}^{2} } \left. {\left[ {\frac{{\left[ {M_{x}^{P} } \right]^{\Theta } \alpha_{m}^{2} + \left[ {M_{y}^{P} } \right]^{\Theta } \beta_{n}^{2} }}{{\alpha_{m} \beta_{n} }}} \right]\left[ {\cos (\alpha_{m} x_{1P} )} \right. - \left. {\cos (\alpha_{m} x_{2P} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1P} ) - \sin (\beta_{n} y_{2P} )} \right]_{L} } \right\} \hfill \\ & \quad \times \left[ {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right]^{ - 1} \hfill \\ \end{aligned}$$

(53)

$$S_{mn}^{6} = \delta_{n} ( - 1)^{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {\frac{{\alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }} - 2H\alpha_{m}^{2} - D_{22} \beta_{n}^{2} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}} + 1} \right]$$

(54)

$$S_{mn}^{7} = \delta_{n} ( - 1)^{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {2H\alpha_{m}^{2} ( - 1)^{n} + D_{22} ( - 1)^{n} \beta_{n}^{2} - \frac{{( - 1)^{n} \alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}} - ( - 1)^{n} } \right]$$

(55)

$$S_{mn}^{8} = \delta_{n} ( - 1)^{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( { - D_{11} \alpha_{m} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}}} \right]$$

(56)

$$S_{mn}^{9} = \delta_{n} ( - 1)^{n} \left[ {\frac{{\left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left( {D_{11} \alpha_{m} ( - 1)^{m} } \right)}}{{\left( {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right)}}} \right]$$

(57)

$$\begin{aligned} S_{mn}^{10} & = - \delta_{n} ( - 1)^{n} \left( {\frac{{D_{12} }}{{D_{22} }}\alpha_{m}^{2} + \beta_{n}^{2} } \right)\left\{ {\sum\limits_{L = 1}^{Mn} {C_{mn}^{1} \left( {\frac{{ - P_{o} }}{{\alpha_{m} \beta_{n} }}} \right)_{{}} } \left[ {\cos (\alpha_{m} x_{1M} )} \right. - \left. {\cos (\alpha_{m} x_{2M} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1M} ) - \sin (\beta_{n} y_{2M} )} \right]_{L} } \right. \hfill \\ & \quad + \sum\limits_{L = 1}^{Tn} {C_{mn}^{2} } \left. {\left[ {\frac{{\left[ {M_{x}^{P} } \right]^{\Theta } \alpha_{m}^{2} + \left[ {M_{y}^{P} } \right]^{\Theta } \beta_{n}^{2} }}{{\alpha_{m} \beta_{n} }}} \right]\left[ {\cos (\alpha_{m} x_{1P} )} \right. - \left. {\cos (\alpha_{m} x_{2P} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1P} ) - \sin (\beta_{n} y_{2P} )} \right]_{L} } \right\} \hfill \\ & \quad \times \left[ {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right]^{ - 1} \hfill \\ \end{aligned}$$

(58)

$$S_{mn}^{11} = \alpha_{m} \left[ {\frac{{\left( {\frac{{\alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right) - 2H\alpha_{m}^{2} - D_{22} \beta_{n}^{2} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(59)

$$S_{mn}^{12} = \alpha_{m} \left[ {\frac{{2H\alpha_{m}^{2} ( - 1)^{n} + D_{22} ( - 1)^{n} \beta_{n}^{2} - \left( {\frac{{( - 1)^{n} \alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right)}}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(60)

$$S_{mn}^{13} = \alpha_{m} \left[ {\frac{{ - D_{11} \alpha_{m} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(61)

$$S_{mn}^{14} = \alpha_{m} \left[ {\frac{{D_{11} \alpha_{m} ( - 1)^{m} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(62)

$$\begin{aligned} S_{mn}^{15} & = - \alpha_{m} \left\{ {\sum\limits_{L = 1}^{Mn} {C_{mn}^{1} \left( {\frac{{ - P_{o} }}{{\alpha_{m} \beta_{n} }}} \right)_{{}} } \left[ {\cos (\alpha_{m} x_{1M} )} \right. - \left. {\cos (\alpha_{m} x_{2M} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1M} ) - \sin (\beta_{n} y_{2M} )} \right]_{L} } \right. + \sum\limits_{L = 1}^{Tn} {C_{mn}^{2} } \hfill \\ & \quad \times \left. {\left[ {\frac{{\left[ {M_{x}^{P} } \right]^{\Theta } \alpha_{m}^{2} + \left[ {M_{y}^{P} } \right]^{\Theta } \beta_{n}^{2} }}{{\alpha_{m} \beta_{n} }}} \right]\left[ {\cos (\alpha_{m} x_{1P} )} \right. - \left. {\cos (\alpha_{m} x_{2P} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1P} ) - \sin (\beta_{n} y_{2P} )} \right]_{L} } \right\} \hfill \\ & \quad \times \left[ {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right]^{ - 1} \hfill \\ \end{aligned}$$

(63)

$$S_{mn}^{16} = \alpha_{m} ( - 1)^{m} \left[ {\frac{{\left( {\frac{{\alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right) - 2H\alpha_{m}^{2} - D_{22} \beta_{n}^{2} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(64)

$$S_{mn}^{17} = \alpha_{m} ( - 1)^{m} \left[ {\frac{{2H\alpha_{m}^{2} ( - 1)^{n} + D_{22} ( - 1)^{n} \beta_{n}^{2} - \left( {\frac{{( - 1)^{n} \alpha_{m}^{2} (H + 2D_{66} )}}{{D_{22} }}} \right)}}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(65)

$$S_{mn}^{18} = \alpha_{m} ( - 1)^{m} \left[ {\frac{{ - D_{11} \alpha_{m} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(66)

$$S_{mn}^{19} = \alpha_{m} ( - 1)^{m} \left[ {\frac{{D_{11} \alpha_{m} ( - 1)^{m} }}{{D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} }}} \right]$$

(67)

$$\begin{aligned} S_{mn}^{20} & = - \alpha_{m} ( - 1)^{m} \left\{ {\sum\limits_{L = 1}^{Mn} {C_{mn}^{1} \left( {\frac{{ - P_{o} }}{{\alpha_{m} \beta_{n} }}} \right)_{{}} } \left[ {\cos (\alpha_{m} x_{1M} )} \right. - \left. {\cos (\alpha_{m} x_{2M} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1M} ) - \sin (\beta_{n} y_{2M} )} \right]_{L} } \right. + \sum\limits_{L = 1}^{Tn} {C_{mn}^{2} } \hfill \\ & \quad \times \left. {\left[ {\frac{{\left[ {M_{x}^{P} } \right]^{\Theta } \alpha_{m}^{2} + \left[ {M_{y}^{P} } \right]^{\Theta } \beta_{n}^{2} }}{{\alpha_{m} \beta_{n} }}} \right]\left[ {\cos (\alpha_{m} x_{1P} )} \right. - \left. {\cos (\alpha_{m} x_{2P} )} \right]_{L} \left[ {\sin (\beta_{n} y_{1P} ) - \sin (\beta_{n} y_{2P} )} \right]_{L} } \right\} \hfill \\ & \quad \times \left[ {D_{11} \alpha_{m}^{4} + 2H\alpha_{m}^{2} \beta_{n}^{2} + D_{22} \beta_{n}^{4} } \right]^{ - 1} \hfill \\ \end{aligned}$$

(68)