Abstract
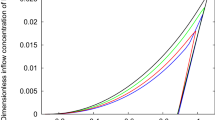
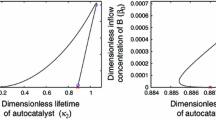
Semi-analytical solutions for cubic autocatalytic reactions are considered in a circularly symmetric reaction–diffusion annulus. The Galerkin method is used to approximate the spatial structure of the reactant and autocatalyst concentrations. Ordinary differential equations are then obtained as an approximation to the governing partial differential equations and analyzed to obtain semi-analytical results for this novel geometry. Singularity theory is used to determine the regions of parameter space in which the different types of steady-state diagram occur. The region of parameter space, in which Hopf bifurcations can occur, is found using a degenerate Hopf bifurcation analysis. A novel feature of this geometry is the effect, of varying the width of the annulus, on the static and dynamic multiplicity. The results show that for a thicker annulus, Hopf bifurcations and multiple steady-state solutions occur in a larger portion of parameter space. The usefulness and accuracy of the semi-analytical results are confirmed by comparison with numerical solutions of the governing partial differential equations.
Similar content being viewed by others
References
Corbel J.M.L., Van Lingen J.N.J., Zevenbergen J.F., Gijzeman O.L.J., Meijerink A.: Strobes: pyrotechnic compositions that show a curious oscillatory combustion. Angew. Chem. Int. Ed. 52, 290–303 (2013)
Sagues F., Epstein I.R.: Nonlinear chemical dynamics. Dalton Trans. 7, 1201–1217 (2003)
Gray P., Scott S.K.: Autocatalytic reactions in the isothermal continuous, stirred tank reactor: isolas and other forms of multistability. Chem. Eng. Sci. 38, 29–43 (1983)
Gray P., Scott P.: Autocatalytic reactions in the isothermal continuous, stirred tank reactor: oscillations and instabilities in the system a + 2b to 3b; b to c. Chem. Eng. Sci. 39, 1087–1097 (1984)
Kay S.R., Scott S.K., Lignola P.G.: The application of singularity theory to isothermal autocatalytic reactions: the influence of uncatalysed reactions. Proc. R. Soc. Lond. A 409, 433–448 (1987)
Scott S.K.: Isolas, mushrooms and oscillations in isothermal, autocatalytic reaction–diffusion equations. Chem. Eng. Sci. 42, 307–315 (1987)
Kay S.R., Scott S.K.: Multiple stationary states, sustained oscillations and transient behavior in autocatalytic reaction–diffusion equations. Proc. R. Soc. Lond. A 418, 345–364 (1988)
Marchant T.R.: Cubic autocatalytic reaction–diffusion equations: semi-analytical solutions. Proc. R. Soc. Lond. A 458, 873–888 (2002)
Tam W., Horstemke W., Noszticzius Z., Swinney H.L.: Sustained spiral waves in a continuously fed unstirred chemical reactor. J. Chem. Phys. 88, 3395–3396 (1988)
Bagyan S., Mair T., Dulos E., Boissonade J., DeKepper P., Muller S.: Glycolytic oscillations and waves in an open spatial reactor: Impact of feedback regulation of phosphofructokinase. Biophys. Chem. 116, 67–76 (2005)
Lavrova A., Bagyan S., Mair T., Hauser M., Schimansky-Geier L.: Modeling of glycolytic wave propagation in an open spatial reactor with inhomogeneous substrate flux. Biosystems 97, 127–133 (2009)
Chaplain M.J., Ganesh M., Graham I.G.: Spatio-temporal pattern formation on spherical surfaces: numerical simulation and application to solid tumour growth. J. Math. Biol. 42, 387–423 (2001)
Roose T., Chapman S.J., Maini P.K.: Mathematical models of avascular tumor growth. SIAM Rev. 49, 179–208 (2007)
Nie Q., Zhang Y.T., Zhao R.: Efficient semi-implicit schemes for stiff systems. J. Comput. Phys. 214, 521–537 (2006)
Jiangguo L., Simon T.: Semi-implicit spectral collocation methods for reaction–diffusion equations on annuli. Numer. Methods Partial Differ. Equ. 27, 1113–1129 (2011)
Farr W.W., Golubitsky M.: Rotating chemical waves in the Gray–Scott model. SIAM J. Appl. Math. 52, 181–221 (1992)
Lubkin S., Rand R.: Oscillatory reaction–diffusion equations on rings. J. Math. Biol. 32, 617–632 (1994)
Bar M., Bangia A.K., Kevrekidis I.G.: Bifurcation and stability analysis of rotating chemical spirals in circular domains: boundary-induced meandering and stabilization. Phys. Rev. E 67, 056126 (2003)
Tsai J.C.: Rotating spiral waves in lambda-omega systems on circular domains. Phys. D 239, 1007–1025 (2010)
Gray B.G., Roberts M.J.: A method for the complete qualitative analysis of two coupledordinary differential equations dependent on three parameters. Proc. R. Soc. Lond. A 416, 361–389 (1988)
Balakotaiah V., Luss D.: Multiplicity features of reacting systems. Chem. Eng. Sci. 38, 1709–1721 (1983)
Guckenheimer J., Holmes P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Springer, New York (1983)
Golubitsky M., Schaeffer D.G.: Singularities and Groups in Bifurcation Theory. Springer, New York (1985)
Author information
Authors and Affiliations
Corresponding author
Additional information
This paper is dedicated to Professor James Hill on the occasion of his 70th birthday. Prof. Hill has been a source of inspiration to the authors, over many years, due to his significant contributions to Applied Mathematics and leadership of the field within Australia. He has also played a key role in the support and mentoring of a new generation of Applied Mathematicians that will influence the field for many years to come.
This article is part of the topical collection “James Hill” guest edited by Scott McCue and Natalie Thamwattana.
Rights and permissions
About this article
Cite this article
Alharthi, M.R., Marchant, T.R. & Nelson, M.I. Cubic autocatalysis in a reaction–diffusion annulus: semi-analytical solutions. Z. Angew. Math. Phys. 67, 65 (2016). https://doi.org/10.1007/s00033-016-0660-0
Received:
Revised:
Published:
DOI: https://doi.org/10.1007/s00033-016-0660-0