On the estimability of parameters in undifferenced, uncombined GNSS network and PPP-RTK user models by means of $\mathcal {S}$-system theory

Abstract

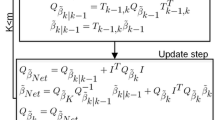
The concept of integer ambiguity resolution-enabled Precise Point Positioning (PPP-RTK) relies on appropriate network information for the parameters that are common between the single-receiver user that applies and the network that provides this information. Most of the current methods for PPP-RTK are based on forming the ionosphere-free combination using dual-frequency Global Navigation Satellite System (GNSS) observations. These methods are therefore restrictive in the light of the development of new multi-frequency GNSS constellations, as well as from the point of view that the PPP-RTK user requires ionospheric corrections to obtain integer ambiguity resolution results based on short observation time spans. The method for PPP-RTK that is presented in this article does not have above limitations as it is based on the undifferenced, uncombined GNSS observation equations, thereby keeping all parameters in the model. Working with the undifferenced observation equations implies that the models are rank-deficient; not all parameters are unbiasedly estimable, but only combinations of them. By application of $\mathcal {S}$-system theory the model is made of full rank by constraining a minimum set of parameters, or S-basis. The choice of this S-basis determines the estimability and the interpretation of the parameters that are transmitted to the PPP-RTK users. As this choice is not unique, one has to be very careful when comparing network solutions in different $\mathcal {S}$-systems; in that case the S-transformation, which is provided by the $\mathcal {S}$-system method, should be used to make the comparison. Knowing the estimability and interpretation of the parameters estimated by the network is shown to be crucial for a correct interpretation of the estimable PPP-RTK user parameters, among others the essential ambiguity parameters, which have the integer property which is clearly following from the interpretation of satellite phase biases from the network. The flexibility of the $\mathcal {S}$-system method is furthermore demonstrated by the fact that all models in this article are derived in multi-epoch mode, allowing to incorporate dynamic model constraints on all or subsets of parameters.

Appendices

Appendix 1: The Kronecker product

If ${A}={\left[ \begin{array}{c@{\quad }c@{\quad }c} a_{11} &{} \cdots &{} a_{1n} \\ \vdots &{} \ddots &{} \vdots \\ a_{m1} &{} \cdots &{} a_{mn} \\ \end{array} \right] }$ is an $m \times n$ matrix and B is a $p \times q$ matrix, then their Kronecker product ${A} \otimes {B}$ is defined as the following $mp \times nq$ matrix (Rao 1973):

$$\begin{aligned} {A} \otimes {B}~=~\left[ \begin{array}{c@{\quad }c@{\quad }c} a_{11} {B} &{} \cdots &{} a_{1n} {B} \\ \vdots &{} \ddots &{} \vdots \\ a_{m1} {B} &{} \cdots &{} a_{mn} {B} \\ \end{array} \right] \end{aligned}$$

(47)

Note: $A \otimes B \ne B \otimes A$. Some of its properties are (assuming that all matrices involved have appropriate dimensions):

$$\begin{aligned} \begin{array}{lll} ({A}+{C}) \otimes {B} &{} = &{} {A} \otimes {B} + {C} \otimes {B} \\ {A} \otimes ({B} \otimes {C}) &{} = &{} ({A} \otimes {B}) \otimes {C} \\ ({A} \otimes {B})({C} \otimes {D}) &{} = &{} ({A} {C}) \otimes ({B} {D}) \\ ({A} \otimes {B})^T &{} = &{} {A}^T \otimes {B}^T \\ ({A} \otimes {B})^{-1} &{} = &{} {A}^{-1} \otimes {B}^{-1} \\ \end{array} \end{aligned}$$

(48)

In the last equation both A and B are assumed to be square and invertible.

Appendix 2: Full-rank design matrices and S-transformation matrices for the network model

In this section, the full-rank design matrix as well as the S-transformation matrix for the different network S-basis choices in this article are derived, where in all cases it is assumed that random-walk temporal constraints are incorporated for all parameters (except the ambiguities that are assumed to be time constant).

1.1 CC-R S-basis

In case of the CC-R S-basis, the S-basis constraints can be casted in the matrix $(S_{\text {CC-R}}^\perp )^T$ as follows, where each of the five ’columns’ represents a parameter group (i.e., receiver positions/ZTDs, receiver clocks/hardware biases, satellite clocks/hardware biases, ionospheric delays and ambiguities):

(49)

Its orthogonal complement $S_{\text {CC-R}}$, such that $(S_{\text {CC-R}}^\perp )^T S_{\text {CC-R}} = 0$, can be constructed as the following block-diagonal matrix:

$$\begin{aligned}&S_{\text {CC-R}}\nonumber \\&\quad =\text {blkdiag}\left\{ I_k \otimes (I_n \otimes I_\nu ),~ \left[ \left( \begin{array}{c@{\quad }c@{\quad }c} 1 &{} 0 &{} 0 \\ 0 &{} I_f &{} 0 \\ 0 &{} 0 &{} F_f \\ \end{array} \right) \otimes C_n,~ I_{k-1}\right. \right. \nonumber \\&\qquad \left. \left. \otimes \left\{ \left( \begin{array}{c@{\quad }c@{\quad }c} 1 &{} 0 &{} 0 \\ 0 &{} I_f &{} 0 \\ 0 &{} 0 &{} I_f \\ \end{array} \right) \right\} \otimes I_n \right] ,~\right. \nonumber \\&\qquad \left[ \left( \begin{array}{c@{\quad }c@{\quad }c} 1 &{} 0 &{} 0 \\ 0 &{} I_f &{} 0 \\ 0 &{} 0 &{} F_f \\ \end{array} \right) \otimes I_m,~ I_{k-1} \otimes \left\{ \left( \begin{array}{c@{\quad }c@{\quad }c} 1 &{} 0 &{} 0 \\ 0 &{} I_f &{} 0 \\ 0 &{} 0 &{} I_f \\ \end{array} \right) \right\} \otimes I_m \right] ,\nonumber \\&\left. \qquad I_k \otimes I_m,~ I_f \otimes (C_n \otimes C_m) \right\} \end{aligned}$$

(50)

Here the $f \times (f-1)$ matrix $F_f$ is defined as: $F_f = {\left( \begin{array}{c@{\quad }c} \mu _1 &{} 0 \\ \mu _2 &{} 0 \\ 0 &{} I_{f-2} \\ \end{array}\right) }$, for which it holds that $\mu _{\text {IF}}^T F_f = 0$. The full-rank design matrix, corresponding to the CC-R S-basis, is obtained by post-multiplying the rank-deficient design matrix $A_\text {net}$ in Eq. (20) with the above $S_{\text {CC-R}}$ and reads as follows:

(51)

where $F_{\text {geo}} = \text{ blkdiag }[F_{\text {geo}}(1),\ldots ,F_{\text {geo}}(k)]$ and $F_{\text {geo}}(i)$ given as in Eq. (21), whereas $F_{\text {ion}} = \text{ blkdiag }[F_{\text {ion}}(1),\ldots ,F_{\text {ion}}(k)]$ and $F_{\text {ion}}(i)$ given as in Eq. (22).

We will now derive the S-transformation matrix corresponding to the CC-R S-basis. Multiplication of $(S_{\text {CC-R}}^\perp )^T$ with the null space matrix $V_\text {net}$ as given in Eq. (23) yields the following block-diagonal matrix:

$$\begin{aligned}&(S_{\text {CC-R}}^\perp )^T V_\text {net} = \text{ blkdiag }\nonumber \\&\quad \left\{ \left( \begin{array}{ccc} 1 &{} 0 &{} 0 \\ 0 &{} I_f &{} 0 \\ 0 &{} 0 &{} I_f \\ \end{array} \right) , I_{n-1}, I_{m}, I_f \otimes I_{n-1}, I_f \otimes I_m \right\} . \end{aligned}$$

(52)

Note that this result is simply an identity matrix. Its inverse matrix $[(S_{\text {CC-R}}^\perp )^T V_\text {net}]^{-1}$ is therefore an identity matrix as well, such that $V_\text {net} [(S_{\text {CC-R}}^\perp )^T V_\text {net}]^{-1} = V_\text {net}$ again. Finally, this results in the following S-transformation matrix for the CC-R S-basis:

(53)

In the above matrix, $E_k$ denotes a matrix of dimension k that is computed as $E_k = C_k D_k^T = I_k - e_k c_k^T$ (similar for $E_n$ and $E_m$). Some of its properties are that $E_k e_k = 0$, $E_k C_k = C_k$ and $E_k E_k = E_k$. Note that the above matrix indeed fulfills the properties of an S-transformation matrix, i.e., $\mathcal {S}_{\text {CC-R}} V_\text {net} = 0$, $\mathcal {S}_{\text {CC-R}} S_{\text {CC-R}} = S_{\text {CC-R}}$ and $\mathcal {S}_{\text {CC-R}} \mathcal {S}_{\text {CC-R}} = \mathcal {S}_{\text {CC-R}}$.

1.2 CC-S S-basis

In case of the CC-S S-basis, the S-basis constraints can be casted in the matrix $(S_{\text {CC-S}}^\perp )^T$ as follows, where each of the five ’columns’ represents a parameter group (i.e., receiver positions/ZTDs, receiver clocks/hardware biases, satellite clocks/hardware biases, ionospheric delays and ambiguities):

(54)

Its orthogonal complement $S_{\text {CC-S}}$, such that $(S_{\text {CC-S}}^\perp )^T S_{\text {CC-S}} = 0$, can be constructed as the following matrix:

(55)

where use is made of the property that $e_m^T D_m = 0$. The full-rank design matrix, corresponding to the CC-S S-basis, is obtained by post-multiplying the rank-deficient design matrix $A_\text {net}$ in Eq. (20) with the above $S_{\text {CC-S}}$ and reads as follows:

(56)

We will now derive the S-transformation matrix corresponding to the CC-S S-basis. Multiplication of $(S_{\text {CC-S}}^\perp )^T$ with the null space matrix $V_\text {net}$ as given in Eq. (23) yields the following matrix plus its inverse:

(57)

This leads to:

(58)

Finally, this results in the following S-transformation matrix for the CC-S S-basis:

(59)

In the above S-transformation matrix, the $m \times m$ matrix $Z_m = I_m-\frac{1}{m} e_m e_m^T$ denotes the “zero-mean” matrix. Some of its properties are that $Z_m e_m = 0$ and $Z_m Z_m = Z_m$. Note that the above matrix indeed fulfills the properties of an S-transformation matrix, i.e., $\mathcal {S}_{\text {CC-S}} V_\text {net} = 0$, $\mathcal {S}_{\text {CC-S}} S_{\text {CC-S}} = S_{\text {CC-S}}$ and $\mathcal {S}_{\text {CC-S}} \mathcal {S}_{\text {CC-S}} = \mathcal {S}_{\text {CC-S}}$.

Appendix 3: Square and invertible transformation between CC-R and CC-S systems

The full-rank transformation from the estimable parameters in the CC-S system to their counterparts in the CC-R system reads:

(60)

Here we made distinction between the estimable parameters at the first epoch, denoted using time index 1, and those for all other epochs, which are collected in one vector without any time index. For example, vector $\mathrm{d}\tilde{t}^s(1)$ denotes the satellite clock parameters at epoch 1 in the CC-R system, whereas vector $\mathrm{d}\tilde{t}^s$ denotes the satellite clock parameters in the same $\mathcal {S}$-system at all other epochs (2 to k).

The inverse transformation, i.e., the transformation from the estimable parameters in the CC-R system to the CC-S system, reads:

(61)

Here use is made of the following properties: $D_m^T C_m = I_{m-1}$, $C_m D_m^T = I_m-e_m c_m^T$, $D_m^T e_m = 0$, $e_m^T D_m = 0$ and $(D_m^T D_m)^{-1} = I_{m-1}-\frac{1}{m}e_{m-1} e_{m-1}^T$.