Skip to main content
SpringerLink
Log in

A novel RBF-based meshless method for solving time-fractional transport equations in 2D and 3D arbitrary domains

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

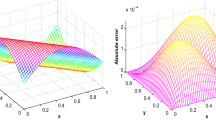
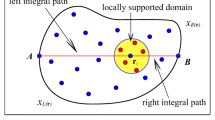
In this paper, we develop a new meshless method for solving a wide class of time-fractional partial differential equations with general space operators in 2D and 3D regular and irregular domains. These equations are usually used to model transport processes in anisotropic media with sub-diffusive phenomena. In this method, the spatial approximation is given in the form of the truncated series over a set of linearly independent functions. Then the system is solved by the use of an efficient backward substitution method which is based on the collocation procedure using modified basis functions. The main aim of the research is to show the accuracy and efficiency of the proposed algorithm over some of the existing methods. The numerical results of ten examples on 2D and 3D domains demonstrate the advantages of the presented approach.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Sun HG, Zhang Y, Baleanu D et al (2018) A new collection of real world applications of fractional calculus in science and engineering. Commun Nonlinear Sci Numer Simul 64:213–231

    MATH  Google Scholar 

  2. Diethelm K (2010) The analysis of fractional differential equations: an application-oriented exposition using differential operators of Caputo type. Springer Science & Business Media, Berlin

    MATH  Google Scholar 

  3. Luchko Y, Gorenflo R (1999) An operational method for solving fractional differential equations with the Caputo derivatives. Acta Mathematica Vietnam 24(2):207–233

    MathSciNet  MATH  Google Scholar 

  4. Huang F, Liu F (2005) The time fractional diffusion equation and the advection-dispersion equation. ANZIAM J 46(3):317–330

    MathSciNet  MATH  Google Scholar 

  5. Das S (2009) Analytical solution of a fractional diffusion equation by variational iteration method. Comput Math Appl 57(3):483–487

    MathSciNet  MATH  Google Scholar 

  6. El-Sayed AMA, El-Kalla IL, Ziada EAA (2010) Analytical and numerical solutions of multi-term nonlinear fractional orders differential equations. Appl Numer Math 60(8):788–797

    MathSciNet  MATH  Google Scholar 

  7. Jiang H, Liu F, Turner I et al (2012) Analytical solutions for the multi-term time-fractional diffusion-wave/diffusion equations in a finite domain. Comput Math Appl 64(10):3377–3388

    MathSciNet  MATH  Google Scholar 

  8. Jiang H, Liu F, Turner I et al (2012) Analytical solutions for the multi-term time-space Caputo–Riesz fractional advection-diffusion equations on a finite domain. J Math Anal Appl 389(2):1117–1127

    MathSciNet  MATH  Google Scholar 

  9. Chen J, Liu F, Anh V et al (2012) The analytical solution and numerical solution of the fractional diffusion-wave equation with damping. Appl Math Comput 219(4):1737–1748

    MathSciNet  MATH  Google Scholar 

  10. Xu Y, He Z, Agrawal OP (2013) Numerical and analytical solutions of new generalized fractional diffusion equation. Comput Math Appl 66(10):2019–2029

    MathSciNet  MATH  Google Scholar 

  11. Zhao YM, Zhang YD, Liu F et al (2016) Analytical solution and nonconforming finite element approximation for the 2D multi-term fractional subdiffusion equation. Appl Math Model 40(19–20):8810–8825

    MathSciNet  MATH  Google Scholar 

  12. Chen JS, Liu CW (2011) Generalized analytical solution for advection-dispersion equation in finite spatial domain with arbitrary time-dependent inlet boundary condition. Hydrol Earth Syst Sci 15(8):2471–2479

    MathSciNet  Google Scholar 

  13. Lin Y, Xu C (2007) Finite difference/spectral approximations for the time-fractional diffusion equation. J Comput Phys 225(2):1533–1552

    MathSciNet  MATH  Google Scholar 

  14. Sousa E, Li C (2015) A weighted finite difference method for the fractional diffusion equation based on the Riemann–Liouville derivative. Appl Numer Math 90:22–37

    MathSciNet  MATH  Google Scholar 

  15. Alikhanov AA (2015) A new difference scheme for the time fractional diffusion equation. J Comput Phys 280:424–438

    MathSciNet  MATH  Google Scholar 

  16. Vong S, Lyu P, Wang Z (2016) A compact difference scheme for fractional sub-diffusion equations with the spatially variable coefficient under Neumann boundary conditions. J Sci Comput 66(2):725–739

    MathSciNet  MATH  Google Scholar 

  17. Fazio R, Jannelli A (2018) A finite difference method on quasi-uniform mesh for time-fractional advection-diffusion equations with source term. Appl Sci 8:960–976

    Google Scholar 

  18. Liu F, Zhuang P, Anh V et al (2007) Stability and convergence of the difference methods for the space-time fractional advection-diffusion equation. Appl Math Comput 191(1):12–20

    MathSciNet  MATH  Google Scholar 

  19. Gao GH, Sun HW, Sun ZZ (2015) Stability and convergence of finite difference schemes for a class of time-fractional sub-diffusion equations based on certain superconvergence. J Comput Phys 280:510–528

    MathSciNet  MATH  Google Scholar 

  20. Safdari H, Mesgarani H, Javidi M et al (2020) Convergence analysis of the space fractional-order diffusion equation based on the compact finite difference scheme. Comput Appl Math 39(2):1–15

    MathSciNet  MATH  Google Scholar 

  21. Li X, Rui H (2020) Stability and convergence based on the finite difference method for the nonlinear fractional cable equation on non-uniform staggered grids. Appl Numer Math 152:403–421

    MathSciNet  MATH  Google Scholar 

  22. Zhao X, Sun Z, Karniadakis GE (2015) Second-order approximations for variable order fractional derivatives: algorithms and applications. J Comput Phys 293:184–200

    MathSciNet  MATH  Google Scholar 

  23. Ren J, Gao G (2015) Efficient and stable numerical methods for the two-dimensional fractional Cattaneo equation. Numer Algorithms 69(4):795–818

    MathSciNet  MATH  Google Scholar 

  24. Zhang J, Zhang X, Yang B (2018) An approximation scheme for the time fractional convection-diffusion equation. Appl Math Comput 335:305–312

    MathSciNet  MATH  Google Scholar 

  25. Jiang Y, Ma J (2011) High-order finite element methods for time-fractional partial differential equations. J Comput Appl Math 235(11):3285–3290

    MathSciNet  MATH  Google Scholar 

  26. Ford NJ, Xiao J, Yan Y (2011) A finite element method for time fractional partial differential equations. Fract Calc Appl Anal 14(3):454–474

    MathSciNet  MATH  Google Scholar 

  27. Bu W, Tang Y, Yang J (2014) Galerkin finite element method for two-dimensional Riesz space fractional diffusion equations. J Comput Phys 276:26–38

    MathSciNet  MATH  Google Scholar 

  28. Patnaik S, Sidhardh S, Semperlotti F (2020) A Ritz-based finite element method for a fractional-order boundary value problem of nonlocal elasticity. Int J Solids Struct 202:398–417

    Google Scholar 

  29. Li M, Gu XM, Huang C et al (2018) A fast linearized conservative finite element method for the strongly coupled nonlinear fractional Schrodinger equations. J Comput Phys 358:256–282

    MathSciNet  MATH  Google Scholar 

  30. Wu L, Zhai S (2020) A new high order ADI numerical difference formula for time-fractional convection-diffusion equation. Appl Math Comput 387:124564

    MathSciNet  MATH  Google Scholar 

  31. Pandey P, Das S, Craciun EM et al (2021) Two-dimensional nonlinear time fractional reaction-diffusion equation in application to sub-diffusion process of the multicomponent fluid in porous media. Meccanica 56(1):99–115

    MathSciNet  Google Scholar 

  32. Zada L, Aziz I (2020) Numerical solution of fractional partial differential equations via Haar wavelet. Numer Methods Partial Differ Equ. https://doi.org/10.1002/num.22658

    Article  MATH  Google Scholar 

  33. Liu GR (2009) Meshfree methods: moving beyond the finite element method. CRC Press, Boca Raton

    Google Scholar 

  34. Gu Y, Sun HG (2020) A meshless method for solving three-dimensional time fractional diffusion equation with variable-order derivatives. Appl Math Model 78:539–549

    MathSciNet  MATH  Google Scholar 

  35. Liu Q, Gu YT, Zhuang P et al (2011) An implicit RBF meshless approach for time fractional diffusion equations. Comput Mech 48(1):1–12

    MathSciNet  MATH  Google Scholar 

  36. Dehghan M, Abbaszadeh M, Mohebbi A (2015) Error estimate for the numerical solution of fractional reaction-subdiffusion process based on a meshless method. J Comput Appl Math 280:14–36

    MathSciNet  MATH  Google Scholar 

  37. Zhuang P, Gu YT, Liu F et al (2011) Time-dependent fractional advection-diffusion equations by an implicit MLS meshless method. Int J Numer Meth Eng 88(13):1346–1362

    MathSciNet  MATH  Google Scholar 

  38. Tayebi A, Shekari Y, Heydari MH (2017) A meshless method for solving two-dimensional variable-order time fractional advection-diffusion equation. J Comput Phys 340:655–669

    MathSciNet  MATH  Google Scholar 

  39. Arqub OA, Shawagfeh N (2019) Application of reproducing kernel algorithm for solving Dirichlet time-fractional diffusion-Gordon types equations in porous media. J Porous Media 22(4):411–434

    Google Scholar 

  40. Abu Arqub O (2019) Application of residual power series method for the solution of time-fractional Schrodinger equations in one-dimensional space. Fund Inform 166(2):87–110

    MathSciNet  MATH  Google Scholar 

  41. Djennadi S, Shawagfeh N, Arqub OA (2021) A fractional Tikhonov regularization method for an inverse backward and source problems in the time-space fractional diffusion equations. Chaos, Solitons & Fractals 150:111127

    MathSciNet  MATH  Google Scholar 

  42. Arqub OA (2019) Numerical simulation of time-fractional partial differential equations arising in fluid flows via reproducing Kernel method. Int J Numer Methods Heat Fluid Flow 30(11):4711–4733

    Google Scholar 

  43. Abbasbandy S, Shirzadi A (2011) MLPG method for two-dimensional diffusion equation with Neumann’s and non-classical boundary conditions. Appl Numer Math 61(2):170–180

    MathSciNet  MATH  Google Scholar 

  44. Shirzadi A, Ling L, Abbasbandy S (2012) Meshless simulations of the two-dimensional fractional-time convection-diffusion-reaction equations. Eng Anal Bound Elem 36(11):1522–1527

    MathSciNet  MATH  Google Scholar 

  45. Kumar A, Bhardwaj A (2020) A local meshless method for time fractional nonlinear diffusion wave equation. Numer Algorithms 85(4):1311–1334

    MathSciNet  MATH  Google Scholar 

  46. Kumar A, Bhardwaj A, Kumar BVR (2019) A meshless local collocation method for time fractional diffusion wave equation. Comput Math Appl 78(6):1851–1861

    MathSciNet  MATH  Google Scholar 

  47. Karamali G, Dehghan M, Abbaszadeh M (2019) Numerical solution of a time-fractional PDE in the electroanalytical chemistry by a local meshless method. Eng Comput 35(1):87–100

    Google Scholar 

  48. Wang C, Wang F, Gong Y (2021) Analysis of 2D heat conduction in nonlinear functionally graded materials using a local semi-analytical meshless method. AIMS Math 6(11):12599–12618

    MathSciNet  MATH  Google Scholar 

  49. Wang F, Fan CM, Zhang C, Lin JA (2020) Localized space-time method of fundamental solutions for diffusion and convection-diffusion problems. Adv Appl Math Mech 12:940–958

    MathSciNet  MATH  Google Scholar 

  50. Reutskiy SY (2017) A new semi-analytical collocation method for solving multi-term fractional partial differential equations with time variable coefficients. Appl Math Model 45:238–254

    MathSciNet  MATH  Google Scholar 

  51. Reutskiy S, Fu ZJ (2018) A semi-analytic method for fractional-order ordinary differential equations: testing results. Fract Calc Appl Anal 21(6):1598–1618

    MathSciNet  MATH  Google Scholar 

  52. Lin J, Hong YX, Lu J (2021) New method for the determination of convective heat transfer coefficient in fully-developed laminar pipe flow. Acta Mechanica Sinica

  53. Lin J, Feng W, Reutskiy S et al (2021) A new semi-analytical method for solving a class of time fractional partial differential equations with variable coefficients. Appl Math Lett 112:106712

    MathSciNet  MATH  Google Scholar 

  54. Esmaeili S, Shamsi M, Luchko Y (2011) Numerical solution of fractional differential equations with a collocation method based on Müntz polynomials. Comput Math Appl 62(3):918–929

    MathSciNet  MATH  Google Scholar 

  55. Mokhtary P, Ghoreishi F, Srivastava HM (2016) The Müntz-Legendre Tau method for fractional differential equations. Appl Math Model 40(2):671–684

    MathSciNet  MATH  Google Scholar 

  56. Bahmanpour M, Tavassoli-Kajani M, Maleki M (2018) A Müntz wavelets collocation method for solving fractional differential equations. Comput Appl Math 37(4):5514–5526

    MathSciNet  MATH  Google Scholar 

  57. Safari F, Azarsa P (2020) Backward substitution method based on Müntz polynomials for solving the nonlinear space fractional partial differential equations. Math Methods Appl Sci 43(2):847–864

    MathSciNet  MATH  Google Scholar 

  58. Maleknejad K, Rashidinia J, Eftekhari T (2021) Numerical solutions of distributed order fractional differential equations in the time domain using the Müntz-Legendre wavelets approach. Numer Methods Partial Differ Equ 37(1):707–731

    Google Scholar 

  59. Liu J, Li X, Hu X (2019) A RBF-based differential quadrature method for solving two-dimensional variable-order time fractional advection-diffusion equation. J Comput Phys 384:222–238

    MathSciNet  MATH  Google Scholar 

  60. Qiao Y, Zhao J, Feng X (2019) A compact integrated RBF method for time fractional convection-diffusion-reaction equations. Comput Math Appl 77(9):2263–2278

    MathSciNet  MATH  Google Scholar 

  61. David W, Hahn M (2012) Necati Özişik, heat conduction, 3rd edn. Wiley, Amsterdam

    Google Scholar 

  62. Lin J, Reutskiy SY, Lu J (2018) A novel meshless method for fully nonlinear advection-diffusion-reaction problems to model transfer in anisotropic media. Appl Math Comput 339:459–476

    MathSciNet  MATH  Google Scholar 

  63. Lin J, Chen F, Zhang Y et al (2019) An accurate meshless collocation technique for solving two-dimensional hyperbolic telegraph equations in arbitrary domains. Eng Anal Bound Elem 108:372–384

    MathSciNet  MATH  Google Scholar 

  64. Reutskiy S, Lin J (2020) A RBF-based technique for 3D convection-diffusion-reaction problems in an anisotropic inhomogeneous medium. Comput Math Appl 79(6):1875–1888

    MathSciNet  MATH  Google Scholar 

  65. Lin J, Reutskiy S (2020) A cubic B-spline semi-analytical algorithm for simulation of 3D steady-state convection-diffusion-reaction problems. Appl Math Comput 371:124944

    MathSciNet  MATH  Google Scholar 

  66. Reutskiy S, Zhang Y, Lin J et al (2020) A novel B-spline method to analyze convection-diffusion-reaction problems in anisotropic inhomogeneous medium. Eng Anal Bound Elem 118:216–224

    MathSciNet  MATH  Google Scholar 

  67. Lin J, Zhang Y, Reutskiy S et al (2021) A novel meshless space-time backward substitution method and its application to nonhomogeneous advection-diffusion problems. Appl Math Comput 398:125964

    MathSciNet  MATH  Google Scholar 

  68. Rippa S (1999) An algorithm for selecting a good value for the parameter c in radial basis function interpolation. Adv Comput Math 11(2):193–210

    MathSciNet  MATH  Google Scholar 

  69. Liu CS, Liu D (2018) Optimal shape parameter in the MQ-RBF by minimizing an energy gap functional. Appl Math Lett 86:157–165

    MathSciNet  MATH  Google Scholar 

  70. Chen W, Hong Y, Lin J (2018) The sample solution approach for determination of the optimal shape parameter in the multiquadric function of the Kansa method. Comput Math Appl 75(8):2942–2954

    MathSciNet  MATH  Google Scholar 

  71. Cavoretto R, De Rossi A, Mukhametzhanov MS et al (2021) On the search of the shape parameter in radial basis functions using univariate global optimization methods. J Glob Optim 79(2):305–327

    MathSciNet  MATH  Google Scholar 

  72. Fasshauer GE, McCourt MJ (2015) Kernel-based approximation methods using Matlab. World Scientific Publishing Company, London

    MATH  Google Scholar 

  73. Chen J, Liu F, Liu Q et al (2014) Numerical simulation for the three-dimension fractional sub-diffusion equation. Appl Math Model 38(15–16):3695–3705

    MathSciNet  MATH  Google Scholar 

  74. Lin J, Zhang Y, Reutskiy S (2021) A semi-analytical method for 1D, 2D and 3D time fractional second order dual-phase-lag model of the heat transfer. Alex Eng J 60(6):5879–5896

    Google Scholar 

Download references

Acknowledgements

We would like to thank the editor and referees for giving valuable improvements to the paper. The work was supported by the National Key Research and Development Program of China (No. 2021YFB2600700), the National Natural Science Foundation of China (Nos. 12072103, 52171272), the Natural Science Foundation of Jiangsu Province (No. BK20190073), the State Key Laboratory of Acoustics, Chinese Academy of Sciences (No. SKLA202001), the Key Laboratory of Intelligent Materials and Structural Mechanics of Hebei Province (No. KF2021-01), and the China Postdoctoral Science Foundation (Nos. 2017M611669, 2018T110430).

Author information

Authors and Affiliations

  1. Key Laboratory of Coastal Disaster and Defence (Ministry of Education), College of Mechanics and Materials, Hohai University, Nanjing, 210098, China

    Ji Lin & Jinge Bai

  2. A. Pidhornyi Institute of Mechanical Engineering Problems of NAS of Ukraine, 2/10 Pozharsky street, 61046, Kharkiv, Ukraine

    Sergiy Reutskiy

  3. Nanjing Hydraulic Research Institute, Nanjing, 210029, China

    Jun Lu

Authors
  1. Ji Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jinge Bai
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sergiy Reutskiy
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jun Lu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Ji Lin or Jun Lu.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lin, J., Bai, J., Reutskiy, S. et al. A novel RBF-based meshless method for solving time-fractional transport equations in 2D and 3D arbitrary domains. Engineering with Computers 39, 1905–1922 (2023). https://doi.org/10.1007/s00366-022-01601-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-022-01601-0

Keywords

Search

Navigation