Nonlocal Euler–Bernoulli beam theories with material nonlinearity and their application to single-walled carbon nanotubes

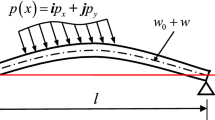
Abstract

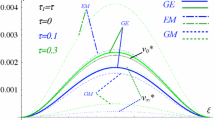
Although the small-scale effect and the material nonlinearity significantly impact the mechanical properties of nanobeams, their combined effects have not attracted the interest of researchers. The present paper proposes two new nonlinear nonlocal Euler–Bernoulli theories to model mechanical properties corresponding to extensible or inextensible nanobeams. Two new theories consider the material nonlinearity and the small-scale effect induced by the nonlocal effect. The new models are used to analyze the static bending and the forced vibrations for single-walled carbon nanotubes (SWCNTs). The results indicate that the material nonlinearity and the nonlocal effect significantly impact SWCNT’s mechanical properties. Therefore, neglecting the two factors may cause qualitative mistakes.

Appendix: Derivation of Eq. (25)

The principle of virtual work for the present dynamical problem is written as [34]

$$ \begin{aligned} &\int\limits_{{t_{1} }}^{{t_{2} }} {\left\{ {\iiint\limits_{V} {\sigma_{xx} \delta \varepsilon_{xx} }dxdydz} \right.} - \delta \iiint\limits_{V} {\frac{\rho }{2}\left[ {\left( {\frac{\partial u}{{\partial t}}} \right)^{2} } \right.} \hfill \\ &\quad \left. {\left. { + \left( {\frac{\partial w}{{\partial t}}} \right)^{2} } \right]dxdydz - \int\limits_{0}^{l} {\overline{F}\delta wdx} - N\delta u} \right\}dt = 0. \hfill \\ \end{aligned} $$

(A1)

here $\rho$ is the density of the beam. For an inextensional beam, the longitudinal deformation $u$ is mainly induced by the transverse deformation $w$ and can be written as [37]: $\partial u/\partial x \approx - \left( {\partial w/\partial x} \right)^{2} /2$. Integrating this equation with respect to $x$ and using the boundary condition $u = 0$ at $x = 0$, we have $u \approx - \int\limits_{0}^{x} {\left[ {\left( {\partial w/\partial s} \right)^{2} /2} \right]\,} ds.$ Therefore, the virtual work of the axial load and the longitudinal velocity is

$$ N\delta u = - N\delta \int\limits_{0}^{x} {\frac{1}{2}\left( {\frac{\partial w}{{\partial s}}} \right)^{2} \,} ds $$

(A2)

$$ \frac{\partial u}{{\partial t}} \approx - \frac{1}{2}\frac{\partial }{\partial t}\int\limits_{0}^{s} {\left( {\frac{\partial w}{{\partial s}}} \right)^{2} \,} ds. $$

(A3)

Substituting Eqs. (A2), (A3) and Eq. (21) into Eq. (A1), and considering $M = \iint\limits_{A} {z\sigma_{xx} dA}$, $N = \iint\limits_{A} {\sigma_{xx} dA}$, we have

$$ \begin{gathered} - \int\limits_{{t_{1} }}^{{t_{2} }} {\left\{ {\int\limits_{0}^{l} {M\delta \left[ {\frac{{\partial^{2} w}}{{\partial x^{2} }} + \frac{1}{2}\left( {\frac{\partial w}{{\partial x}}} \right)^{2} \frac{{\partial^{2} w}}{{\partial x^{2} }}} \right]dx} } \right.} \hfill \\ \quad + \delta \int\limits_{0}^{l} {\frac{m}{2}\left\{ {\frac{1}{4}\left[ { - \frac{\partial }{\partial t}\int\limits_{0}^{s} {\left( {\frac{\partial w}{{\partial s}}} \right)^{2} \,} ds} \right]^{2} } \right.} \hfill \\ \quad + \left. {\left( {\frac{\partial w}{{\partial t}}} \right)^{2} } \right\}dx + \int\limits_{0}^{l} {\overline{F}\delta wdx} \left. { + N\delta \left[ {\frac{1}{2}\left( {\frac{\partial w}{{\partial x}}} \right)^{2} } \right]} \right\}dt = 0 \hfill \\ \end{gathered} $$

(A4)

By performing complex but straightforward calculations, including integrations by parts on Eq. (A4), we have

$$ \begin{gathered} \int\limits_{{t_{1} }}^{{t_{2} }} {\left\{ {\int\limits_{0}^{l} {\left\{ {\frac{{\partial^{2} M}}{{\partial x^{2} }} + \frac{1}{2}\frac{\partial }{\partial x}\left[ {\frac{\partial M}{{\partial x}}\left( {\frac{\partial w}{{\partial x}}} \right)^{2} } \right] + N\frac{{\partial^{2} w}}{{\partial x^{2} }}} \right.} } \right.} - m\frac{{\partial^{2} w}}{{\partial t^{2} }} - \frac{m}{2}\frac{\partial }{\partial x}\left\{ {\frac{\partial w}{{\partial x}}} \right. \hfill \\ \left. {\left. {\left. {\int\limits_{l}^{x} {\left[ {\frac{{\partial^{2} }}{{\partial t^{2} }}\int\limits_{0}^{x} {\left( {\frac{\partial w}{{\partial s}}} \right)^{2} ds} } \right]ds} } \right\} - \overline{F}} \right\}\delta w\,dx + {\text{boundary}}\,{\text{terms}}} \right\}dt = 0. \hfill \\ \end{gathered} $$

(A5)

Since the quantity $\delta w$ is arbitrary, we have

$$ \begin{aligned} &\frac{{\partial^{2} M}}{{\partial x^{2} }} + \frac{1}{2}\frac{\partial }{\partial x}\left[ {\frac{\partial M}{{\partial x}}\left( {\frac{\partial w}{{\partial x}}} \right)^{2} } \right] + N\frac{{\partial^{2} w}}{{\partial x^{2} }} - m\frac{{\partial^{2} w}}{{\partial t^{2} }} \hfill \\ &\quad - \frac{m}{2}\frac{\partial }{\partial x}\left\{ {\frac{\partial w}{{\partial x}}\int\limits_{l}^{x} {\left[ {\frac{{\partial^{2} }}{{\partial t^{2} }}\int\limits_{0}^{x} {\left( {\frac{\partial w}{{\partial s}}} \right)^{2} ds} } \right]ds} } \right\} - \overline{F} = 0. \hfill \\ \end{aligned} $$

(A6)

Equation (25) can be obtained by moving the inertia and load terms to the right side of Eq. (A6).