Skip to main content
SpringerLink
Log in

Topological recursion for Gaussian means and cohomological field theories

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

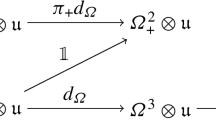
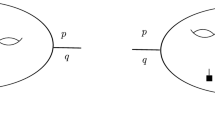
Abstract

We introduce explicit relations between genus-filtrated s-loop means of the Gaussian matrix model and terms of the genus expansion of the Kontsevich–Penner matrix model (KPMM), which is the generating function for volumes of discretized (open) moduli spaces M disc g,s (discrete volumes). Using these relations, we express Gaussian means in all orders of the genus expansion as polynomials in special times weighted by ancestor invariants of an underlying cohomological field theory. We translate the topological recursion of the Gaussian model into recurrence relations for the coefficients of this expansion, which allows proving that they are integers and positive. We find the coefficients in the first subleading order for M g,1 for all g in three ways: using the refined Harer–Zagier recursion, using the Givental-type decomposition of the KPMM, and counting diagrams explicitly.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J. Harer and D. Zagier, Invent. Math., 85, 457–485 (1986).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  2. É. Brezin and S. Hikami, Commun. Math. Phys., 283, 507–521 (2008); arXiv:0708.2210v1 [hep-th] (2007).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  3. A. Morozov and Sh. Shakirov, “From Brezin–Hikami to Harer–Zagier formulas for Gaussian correlators,” arXiv:1007.4100v1 [hep-th] (2010).

    Google Scholar 

  4. L. Chekhov and B. Eynard, JHEP, 0603, 014 (2006); arXiv:hep-th/0504116 (2005).

    Article  MathSciNet  ADS  Google Scholar 

  5. B. Eynard and N. Orantin, Commun. Number Theory Phys., 1, 347–452 (2007).

    Article  MATH  MathSciNet  Google Scholar 

  6. S. Gukov and P. Sułkowski, JHEP, 1202, 070 (2012); arXiv:1108.0002 (2011).

    Article  ADS  Google Scholar 

  7. M. Mulase and P. Sułkowski, “Spectral curves and the Schrödinger equations for the Eynard–Orantin recursion,” arXiv:1210.3006 (2012).

    Google Scholar 

  8. O. Dumitrescu and M. Mulase, “Quantization of spectral curves for meromorphic Higgs bundles through topological recursion,” arXiv:1411.1023 (2014).

    Google Scholar 

  9. J. E. Andersen, L. O. Chekhov, P. Norbury, and R. C. Penner, “Models of discretized moduli spaces, cohomological field theories, and Gaussian means,” arXiv:1501.05867v1 (2015).

    Google Scholar 

  10. L. Chekhov and Yu. Makeenko, Modern Phys. Lett. A, 7, 1223–1236 (1992); arXiv:hep-th/9201033v1 (1992).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  11. J. Ambjørn, L. Chekhov, C. F. Kristjansen, and Yu. Makeenko, Nucl. Phys. B, 404, 127–172 (1993); Erratum, 449, 681 (1995); arXiv:hep-th/9302014v1 (1993).

    Article  ADS  Google Scholar 

  12. Y. Manin, Frobenius Manifolds, Quantum Cohomology, and Moduli Spaces (AMS Colloq. Publ., Vol. 47), Amer. Math. Soc., Providence, R. I. (1999).

    MATH  Google Scholar 

  13. L. Chekhov and Yu. Makeenko, Phys. Lett. B, 278, 271–278 (1992); arXiv:hep-th/9202006v1 (1992).

    Article  MathSciNet  ADS  Google Scholar 

  14. A. Marshakov, A. Mironov, and A. Morozov, Phys. Lett. B, 265, 99–107 (1991).

    Article  MathSciNet  ADS  Google Scholar 

  15. P. Norbury, “Quantum curves and topological recursion,” arXiv:1502.04394v1 [math-ph] (2015).

    Google Scholar 

  16. L. Chekhov, J. Geom. Phys., 12, 153–164 (1993); arXiv:hep-th/9205106v1 (1992).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  17. P. Norbury, Trans. Amer. Math. Soc., 365, 1687–1709 (2013).

    Article  MATH  MathSciNet  Google Scholar 

  18. M. Mulase and M. Penkava, Adv. Math., 230, 1322–1339 (2012); arXiv:1009.2135v2 [math.AG] (2010).

    Article  MATH  MathSciNet  Google Scholar 

  19. P. Norbury, Math. Res. Lett., 17, 467–481 (2010).

    Article  MATH  MathSciNet  Google Scholar 

  20. L. Chekhov, Acta Appl. Math., 48, 33–90 (1997); arXiv:hep-th/9509001v1 (1995).

    Article  MATH  MathSciNet  Google Scholar 

  21. A. B. Givental, Moscow Math. J., 1, 551–568 (2001).

    MATH  MathSciNet  Google Scholar 

  22. B. Eynard, Commun. Number Theory Phys., 8, 541–588 (2014); arXiv:1110.2949v1 [math-ph] (2011).

    Article  MATH  MathSciNet  Google Scholar 

  23. P. Dunin-Barkowski, N. Orantin, S. Shadrin, and L. Spitz, Commun. Math. Phys., 328, 669–700 (2014); arXiv:1211.4021v1 [math-ph] (2012).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  24. B. Eynard, JHEP, 0411, 031 (2004); arXiv:hep-th/0407261v1 (2004).

    Article  MathSciNet  ADS  Google Scholar 

  25. L. Chekhov, B. Eynard, and N. Orantin, JHEP, 0612, 053 (2006); arXiv:math-ph/0603003v2 (2006).

    Article  MathSciNet  ADS  Google Scholar 

  26. A. Alexandrov, A. Mironov, and A. Morozov, Internat. J. Mod. Phys. A, 19, 4127–4165 (2004); arXiv:hep-th/0310113v1 (2003).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  27. B. Dubrovin, “Geometry of 2D topological field theories,” in: Integrable Systems and Quantum groups (Lect. Notes Math., Vol. 1620, M. Francaviglia and S. Greco, eds.), Springer, Berlin (1996), pp. 120–348; arXiv:hep-th/9407018v1 (1994).

    Chapter  Google Scholar 

  28. P. Dunin-Barkowski, P. Norbury, N. Orantin, P. Popolitov, and S. Shadrin, “Superpotentials and the Eynard–Orantin topological recursion,” (in preparation).

  29. J. E. Andersen, R. C. Penner, C. M. Reidys, and M. S. Waterman, J. Math. Biol., 67, 1261–1278 (2013).

    Article  MATH  MathSciNet  Google Scholar 

  30. J. Harer, Invent. Math., 84, 157–176 (1986).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  31. K. Strebel, Quadratic Differentials (Ergebn. Math. ihrer Grenzg., Vol. 3), Springer, Berlin (1984).

    Book  MATH  Google Scholar 

  32. R. C. Penner, Commun. Math. Phys., 113, 299–339 (1987).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  33. R. C. Penner, J. Differential Geom., 27, 35–53 (1988).

    MATH  MathSciNet  Google Scholar 

  34. M. L. Kontsevich, Commun. Math. Phys., 147, 1–23 (1992).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  35. S. Kharchev, A. Marshakov, A. Mironov, A. Morozov, and A. Zabrodin, Phys. Lett. B, 275, 311–314 (1992); arXiv:hep-th/9111037v1 (1991).

    Article  MathSciNet  ADS  Google Scholar 

  36. J. Ambjørn and L. Chekhov, Ann. Inst. Henri Poincaré D, 1, 337–361 (2014); arXiv:1404.4240v2 [math.AG] (2014).

    Article  Google Scholar 

  37. A. Alexandrov, A. Mironov, A. Morozov, and S. Natanzon, JHEP, 1411, 080 (2014); arXiv:1405.1395v3 [hep-th] (2014).

    Article  MathSciNet  ADS  Google Scholar 

  38. J. E. Andersen, L. O. Chekhov, C. M. Reidys, R. C. Penner, and P. Sułkowski, Nucl. Phys. B, 866, 414–443 (2013); arXiv:1205.0658v1 [hep-th] (2012).

    Article  MATH  ADS  Google Scholar 

  39. S. Keel, Trans. Amer. Math. Soc., 330, 545–574 (1992).

    MATH  MathSciNet  Google Scholar 

  40. B. Dubrovin, “Geometry of 2D topological field theories,” in: Integrable Systems and Quantum Groups (Lect. Notes Math., Vol. 1620, M. Francaviglia and S. Greco, eds.), Springer, Berlin (1996), pp. 120–348.

    Chapter  Google Scholar 

  41. C. Teleman, Invent. Math., 188, 525–588 (2012).

    Article  MATH  MathSciNet  ADS  Google Scholar 

  42. U. Haagerup and S. Thorbjørnsen, Infin. Dimens. Anal. Quantum Probab. Relat. Top., 15, 1250003 (2012).

    Article  MathSciNet  Google Scholar 

  43. R. J. Milgram and R. C. Penner, “Riemann’s moduli space and the symmetric group,” in: Mapping Class Groups and Moduli Spaces of Riemann Surfaces (Contemp. Math., Vol. 150, C.-F. Bödigheimer and R. M. Hain, eds.), Amer. Math. Soc., Providence, R. I. (1993), pp. 247–290.

    Chapter  Google Scholar 

  44. B. Fang, C.-C. M. Liu, and Z. Zong, “The Eynard–Orantin recursion and equivariant mirror symmetry for the projective line,” arXiv:1411.3557v2 [math.AG] (2014).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Center for Quantum Geometry of Moduli Spaces, Århus University, Copenhagen, Denmark

    J. E. Andersen & R. C. Penner

  2. California Institute of Technology, Pasadena, California, USA

    J. E. Andersen

  3. Steklov Mathematical Institute of Russian Academy of Sciences, Moscow, Russia

    L. O. Chekhov

  4. University of Melbourne, Melbourne, Australia

    P. Norbury

  5. Institut des Hautes Études Scientifique, Bures-sur-Yvette, France

    R. C. Penner

Authors
  1. J. E. Andersen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. L. O. Chekhov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. P. Norbury
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. R. C. Penner
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to L. O. Chekhov.

Additional information

Sections 2, 3, and 6.2 were written by L. O. Chekhov, and Secs. 1, 4, 5, and 7 and also the other parts of Sec. 6 were written by J. E. Andersen, P. Norbury, and R. C. Penner.

The research of L. O. Chekhov was funded by a grant from the Russian Science Foundation (Project No. 14-50-00005) and was performed in Steklov Mathematical Institute of Russian Academy of Sciences.

Prepared from an English manuscript submitted by the authors; for the Russian version, see Teoreticheskaya i Matematicheskaya Fizika, Vol. 185, No. 3, pp. 371–409, December, 2015.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Andersen, J.E., Chekhov, L.O., Norbury, P. et al. Topological recursion for Gaussian means and cohomological field theories. Theor Math Phys 185, 1685–1717 (2015). https://doi.org/10.1007/s11232-015-0373-0

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11232-015-0373-0

Keywords

Search

Navigation