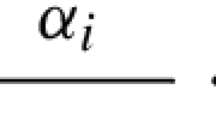Skip to main content
SpringerLink
Log in

Continuous quivers of type A (I) foundations

  • Published:
Rendiconti del Circolo Matematico di Palermo Series 2 Aims and scope Submit manuscript

Abstract

We generalize quivers of type A to continuous quivers of type A and prove initial results about pointwise finite-dimensional representations. Among these results is the classification of those representations and a decomposition theorem, recovering results of Botnan and Crawley-Boevey (J Algebra Appl (2015). https://doi.org/10.1142/S0219498815500668, Decomposition of persistence modules, to appear in Proceedings of the American Mathematical Society, preprint: https://arxiv.org/pdf/1811.08946.pdf). We also classify the indecomposable pointwise finite-dimensional projective representations. Finally, we prove that many of the properties of finite-dimensional representations of quivers of type \(A_n\) also hold for finitely generated representations of continuous quivers of type A. This is the self-contained foundational part of a series of works to study a generalization of continuous clusters categories and their relationship to other cluster structures of type A.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adams, H., Aminian, M., Farnell, E., Kirby, M., Mirth, J., Neville, R., Peterson, C., Shipman, P., Shonkwiler, C.: A fractal dimension for measures via persistent homology, to appear in Abel Symposia 2019, preprint: arXiv:1808.01079.pdf

  2. Auslander, M., Reiten, I.: Representation theory of Artin algebras. III. Almost split sequences. Commun. Algebra 3(3), 239–294 (1975). https://doi.org/10.1080/00927877508822046

    Article  MathSciNet  MATH  Google Scholar 

  3. Baur, K., Gratz, S.: Transfinite mutations in the completed infinity-gon. J. Combin. Ser. A 155, 321–359 (2018). https://doi.org/10.1016/j.jcta.2017.11.011

    Article  MathSciNet  MATH  Google Scholar 

  4. Botnan, M.B.: Interval decomposition of infinite Zigzag persistence modules. Proc. Am. Math. Soc. 145(8), 3571–3577 (2017). https://doi.org/10.1090/proc/13465

    Article  MathSciNet  MATH  Google Scholar 

  5. Botnan, M.B., Crawley-Boevey, W.: Decomposition of persistence modules, to appear in Proceedings of the American Mathematical Society, preprint: https://arxiv.org/pdf/1811.08946.pdf

  6. Buan, A., Marsh, R., Reineke, M., Reiten, I., Todorov, G.: Tilting theory and cluster combinatorics. Adv. Math. 204(2), 572–618 (2006). https://doi.org/10.1016/j.aim.2005.06.003

    Article  MathSciNet  MATH  Google Scholar 

  7. Caldero, P., Chapoton, F., Schiffler, R.: Quivers with Relations Arising From Clusters (\(A_n\) Case), Transactions of the American Mathematical Society, Volume 358, Number 3, 1347 – 1364

  8. Carlsson, G., de Silva, V., Morozov, D.: Zigzag persistent homology and real-valued functions. In Proceedings of the Twenty-fifth Annual Symposium on Computational Geometry, pp. 247–256 (2009). https://doi.org/10.1145/1542362.1542408

  9. Carlsson, G., Ishkhanov, T., de Silva, V., Zomorodian, A.: On the local behavior of spaces of natural images. Int. J. Comput. Vision 76(1), 1–12 (2008). https://doi.org/10.1007/s11263-007-0056-x

    Article  MathSciNet  MATH  Google Scholar 

  10. Chazal, F., Cohen-Steiner, D., Guibas, L.J., Mémoli, F., Oudot, S.Y.: Gromov-Hausdorff stable signatures for shapes using persistence. Comput. Graph. Forum 28(5), 1393–1403 (2009). https://doi.org/10.1111/j.1467-8659.2009.01516.x

    Article  Google Scholar 

  11. Chazal, F., Oudot, S.: Towards persistence-based reconstruction in Euclidean spaces. In Proceedings of the 24th Annual Symposium on Computational Geometry (2008), pp. 232–241, https://doi.org/10.1145/1377676.1377719

  12. Crawley-Boevey, W.: Decomposition of pointwise finite-dimensional persistence modules. J. Algebra Appl. (2015). https://doi.org/10.1142/S0219498815500668

    Article  MathSciNet  MATH  Google Scholar 

  13. Edelsbrunner, H., Harer, J.L.: Computational Topology: An Introduction. American Mathematical Society, New York (2010)

    MATH  Google Scholar 

  14. Hanson, E.J., Rock, J.D.: Decomposition of Pointwise Finite-Dimensional \({\mathbb{S}}^1\) Persistence Modules, arXiv:2006.13793 [math.RT] (2020), https://arXiv.org/pdf/arXiv:2006.13793

  15. Fomin, S., Zelevinksy, A.: Cluter algebras I: Foundations. J. Am. Math. Soc. 15(2), 497–529 (2002). https://doi.org/10.1090/S0894-0347-01-00385-X

    Article  Google Scholar 

  16. Gabriel, P.: Unzerlegbare Darstellungen. I, Manuscripta. Mathematica 6, 71–103 (1972). https://doi.org/10.1007/BF01298413

    Article  MathSciNet  MATH  Google Scholar 

  17. Gabriel, P., Roĭter, A.V.: Representations of Finite-Dimensional Algebras. Springer-Verlag, Berlin (1997)

    Book  Google Scholar 

  18. Igusa, K., Rock, J.D., Todorov, G.: Continuous Quivers of Type A (III) Embeddings of Cluster Theories, arXiv:2004.10740 [math.RT] (2020), https://arXiv.org/pdf/2004.10740

  19. Igusa, K., Todorov, G.: Continuous Cluster Categories I. Algebr. Represent. Theory 18(1), 65–101 (2015). https://doi.org/10.1007/s10468-014-9481-z

    Article  MathSciNet  MATH  Google Scholar 

  20. Jaquette, J., Schweinhart, B.: Fractal Dimension Estimation with Persistent Homology: A Comparative Study, arXiv:1907.11182v2 [math.DS] (2019), https://arxiv.org/pdf/1907.11182v2.pdf

  21. Nicolau, M., Levine, A.J., Carlsson, G.: Topology based data analysis identifies a subgroup of breast cancers with a unique mutational profile and excellent survival. Proc. Natl. Acad. Sci. 108(17), 7265–7270 (2011). https://doi.org/10.1073/pnas.1102826108

    Article  Google Scholar 

  22. Rock, J.D.: Continuous Quivers of Type A (II) The Auslander-Reiten Space, arXiv:1910.04140v1 [math.RT] (2019), https://arXiv.org/pdf/1910.04140.pdf

  23. Rock, J.D.: Continuous Quivers of Type A (IV) Continuous Mutation and Geometric Models of \({\mathbf{E}}\)-clusters, arXiv:2004.11341 [math.RT] (2020), arXiv:2004.11341

  24. Sala, F., Schiffmann, O.: Fock space representation of the circle quantum group, arXiv:1903.02813v1 [math.QA] (2019), https://arxiv.org/pdf/1903.02813.pdf

  25. Schweinhart, B.: Fractal Dimension and the Persistent Homology of Random Geometric Complexes, arXiv:1808.02196v5 [math.PR], https://arxiv.org/pdf/1808.02196.pdf

  26. Zomorodian, A., Carlsson, G.: Computing persistent homology. Discret. Comput. Geom. 33(2), 249–274 (2005). https://doi.org/10.1007/s00454-004-1146-y

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank Ralf Schiffler for organizing the Cluster Algebra School at the University of Connecticut and Shijie Zhu for helpful discussions. They would also like to thank Magnus B. Botnan, Bill Crawley-Boevey, Bernhard Keller, and Francesco Sala for references to related work. The second author would also like to thank Eric Hanson for helpful discussions.

Author information

Authors and Affiliations

  1. Brandeis University, Waltham, MA, USA

    Kiyoshi Igusa, Job D. Rock & Gordana Todorov

Authors
  1. Kiyoshi Igusa
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Job D. Rock
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Gordana Todorov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kiyoshi Igusa.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

First author supported by the Simons Foundation.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Igusa, K., Rock, J.D. & Todorov, G. Continuous quivers of type A (I) foundations. Rend. Circ. Mat. Palermo, II. Ser 72, 833–868 (2023). https://doi.org/10.1007/s12215-021-00691-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12215-021-00691-x

Keywords

Mathematics Subject Classification

Search

Navigation