Unchained polygons and the N-body problem

To the memory of J. Moser, with admiration

Abstract

We study both theoretically and numerically the Lyapunov families which bifurcate in the vertical direction from a horizontal relative equilibrium in ℝ³. As explained in [1], very symmetric relative equilibria thus give rise to some recently studied classes of periodic solutions. We discuss the possibility of continuing these families globally as action minimizers in a rotating frame where they become periodic solutions with particular symmetries. A first step is to give estimates on intervals of the frame rotation frequency over which the relative equilibrium is the sole absolute action minimizer: this is done by generalizing to an arbitrary relative equilibrium the method used in [2] by V. Batutello and S. Terracini.

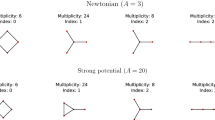
In the second part, we focus on the relative equilibrium of the equal-mass regular N-gon. The proof of the local existence of the vertical Lyapunov families relies on the fact that the restriction to the corresponding directions of the quadratic part of the energy is positive definite. We compute the symmetry groups G _r/s (N, k, η) of the vertical Lyapunov families observed in appropriate rotating frames, and use them for continuing the families globally.

The paradigmatic examples are the “Eight” families for an odd number of bodies and the “Hip- Hop” families for an even number. The first ones generalize Marchal’s P ₁₂ family for 3 bodies, which starts with the equilateral triangle and ends with the Eight [1, 3–6]; the second ones generalize the Hip-Hop family for 4 bodies, which starts from the square and ends with the Hip-Hop [1, 7, 8].

We argue that it is precisely for these two families that global minimization may be used. In the other cases, obstructions to the method come from isomorphisms between the symmetries of different families; this is the case for the so-called “chain” choreographies (see [6]), where only a local minimization property is true (except for N = 3). Another interesting feature of these chains is the deciding role played by the parity, in particular through the value of the angular momentum. For the Lyapunov families bifurcating from the regular N-gon whith N ≤ 6 we check in an appendix that locally the torsion is not zero, which justifies taking the rotation of the frame as a parameter.