Influence of fluid flows on electric double layers in evaporating colloidal sessile droplets

Abstract

A model is developed for describing the transport of charged colloidal particles in an evaporating sessile droplet on the electrified metal substrate in the presence of a solvent flow. The model takes into account the electric charge of colloidal particles and small ions produced by electrolytic dissociation of the active groups on the colloidal particles and solvent molecules. We employ a system of self-consistent Poisson and Nernst–Planck equations for electric potential and average concentrations of colloidal particles and ions with the appropriate boundary conditions. The fluid dynamics, temperature distribution and evaporation process are described with the Navier–Stokes equations, equations of heat conduction and vapor diffusion in air, respectively. The developed model is used to carry out a first-principles numerical simulation of charged silica colloidal particle transport in an evaporating aqueous droplet. We find that electric double layers can be destroyed by a sufficiently strong fluid flow.

Graphic Abstract

Appendices

Appendix A: Basic equations in the case of axial symmetry

In this appendix, we will write the basic equations in cylindrical coordinates r, z taking into account the axial symmetry.

Equation (1) in (r, z) coordinates takes the form

$$\begin{aligned}&- \bigg (\dfrac{\partial \phi }{\partial r} \dfrac{\partial \varepsilon }{\partial r} + \dfrac{\partial \phi }{\partial z} \dfrac{\partial \varepsilon }{\partial z} + \varepsilon \bigg (\dfrac{\partial ^2 \phi }{\partial r^2} + \dfrac{1}{r} \dfrac{\partial \phi }{\partial r} + \dfrac{\partial ^2 \phi }{\partial z^2}\bigg )\bigg )\nonumber \\&\quad = \frac{\rho (r, z, t)}{\varepsilon _{0}}. \end{aligned}$$

(A1)

The Nernst–Planck equation (5) in the (r, z) coordinates can be written as follows:

$$\begin{aligned}&\dfrac{\partial n_c}{\partial t} = \dfrac{q_c}{\gamma _c} \bigg (\dfrac{\partial \phi }{\partial r} \dfrac{\partial n_c}{\partial r} + \dfrac{\partial \phi }{\partial z} \dfrac{\partial n_c}{\partial z} + n_c \bigg (\dfrac{\partial ^2 \psi }{\partial r^2} + \dfrac{1}{r} \dfrac{\partial \phi }{\partial r} + \dfrac{\partial ^2 \phi }{\partial z^2}\bigg )\bigg ) \nonumber \\&\quad + \dfrac{\partial n_c}{\partial r} \dfrac{\partial D_c}{\partial r} + \dfrac{\partial n_c}{\partial z} \dfrac{\partial D_c}{\partial z} +D_c \bigg (\dfrac{\partial ^2 n_c}{\partial r^2} + \dfrac{1}{r} \dfrac{\partial n_c}{\partial r} + \dfrac{\partial ^2 n_c}{\partial z^2}\bigg ) \nonumber \\&\quad - \mathbf{v} \cdot \nabla n_c-n_c \nabla \cdot \mathbf{v} . \end{aligned}$$

(A2)

In the same way, we rewrite Eq. (7) for concentrations of ions, $n_i (r, z, t)$ ($i=\pm$),

$$\begin{aligned}&\dfrac{\partial n_i}{\partial t} = \dfrac{q_i}{\gamma _i} \bigg (\dfrac{\partial \phi }{\partial r} \dfrac{\partial n_i}{\partial r} + \dfrac{\partial \phi }{\partial z} \dfrac{\partial n_i}{\partial z}\nonumber \\&\quad + n_i \bigg (\dfrac{\partial ^2 \phi }{\partial r^2} + \dfrac{1}{r} \dfrac{\partial \phi }{\partial r} + \dfrac{\partial ^2 \phi }{\partial z^2}\bigg )\bigg ) + \dfrac{\partial n_i}{\partial r} \dfrac{\partial D_i}{\partial r} + \dfrac{\partial n_i}{\partial z} \dfrac{\partial D_i}{\partial z}\nonumber \\&\quad + D_i \bigg (\dfrac{\partial ^2 n_i}{\partial r^2} + \dfrac{1}{r} \dfrac{\partial n_i}{\partial r} + \dfrac{\partial ^2 n_i}{\partial z^2}\bigg ) - \mathbf{v} \cdot \nabla n_i-n_i \nabla \cdot \mathbf{v} .\nonumber \\ \end{aligned}$$

(A3)

In our simulation, we deal with an incompressible liquid, so that $\nabla \cdot \mathbf {v}=0$.

The boundary conditions for the electrostatic potential have the following form:

$$\begin{aligned}&\phi _{d}^{(sub)}=\phi _{sub}=\Phi , \end{aligned}$$

(A4)

$$\begin{aligned}&\varepsilon \bigg (r \dfrac{\partial \phi _{d}^{(+)}}{\partial r} + (z+R\cot {\theta }) \dfrac{\partial \phi _{d}^{(+)}}{\partial z}\bigg )\nonumber \\&\quad = r \dfrac{\partial \phi _{d}^{(-)}}{\partial r} + (z+R\cot {\theta }) \dfrac{\partial \phi _{d}^{(-)}}{\partial z}, \end{aligned}$$

(A5)

$$\begin{aligned}&\phi _d^{(-)} = \phi _d^{(+)}, \quad \phi \big | _{|r| \rightarrow \infty } = 0. \end{aligned}$$

(A6)

where R is the radius of the substrate and $\theta$ is the contact angle. The boundary conditions for the concentrations take the following form:

$$\begin{aligned}&r \dfrac{\partial n_{c,\pm }}{\partial r} + (z+R\cot {\theta }) \dfrac{\partial n_{c,\pm }}{\partial z} \nonumber \\&\quad +\dfrac{q_{c,\pm }n_{c,\pm }}{k_{B} T} \bigg (r \dfrac{\partial \phi _{d}^{(+)}}{\partial r} + (z+R\cot {\theta }) \dfrac{\partial \phi _{d}^{(+)}}{\partial z}\bigg ) = 0,\nonumber \\ \end{aligned}$$

(A7)

$$\begin{aligned}&\dfrac{\partial n_{c,\pm }}{\partial z} + \dfrac{q_{c,\pm }n_{c,\pm }}{k_{B} T} \dfrac{\partial \phi _{d}^{(sub)}}{\partial z} = 0. \end{aligned}$$

(A8)

Appendix B: Temperature distribution inside the droplet

The field of temperature, $T(\mathbf {r},t)$, inside the droplet can be obtained from the numerical solution of the heat transfer equation taking into account the liquid motion

$$\begin{aligned} \dfrac{\partial T}{\partial t} + \mathbf{v} \cdot \nabla T = \kappa \Delta T, \end{aligned}$$

(B1)

where $\kappa$ is the thermal diffusivity of the liquid. The boundary conditions for this equation have the following form: $\partial T / \partial r = 0$ at $r = 0$; $T = T_0$ at $z = 0$; $\partial T / \partial {\mathbf {n}} = -Q_0(r)/k$ at the droplet free boundary, where k is the thermal conductivity of the liquid; $Q_0(r) = LJ_s(r)$ is the heat flow with the specific heat of vaporization, L, and the vaporization flux density, $J_s(r)$, which can be calculated by the following interpolation formula [44]

$$\begin{aligned} J_s (r) = J_0(\theta ) (1-r^2/R^2)^{-(1/2-\theta /\pi )}, \end{aligned}$$

(B2)

where $J_0 (\theta )$ can be determined by the following expressions [45]:

$$\begin{aligned}&J_0 (\theta ) / (1-\Lambda (\theta )) = J_0(\pi /2) (0.27 \theta ^2 + 1.3), \end{aligned}$$

(B3)

$$\begin{aligned}&\Lambda (\theta ) = 0.2239(\theta -\pi /4)^2+0.3619, \end{aligned}$$

(B4)

$$\begin{aligned}&J_0(\pi /2) = D u_s/R. \end{aligned}$$

(B5)

We note that in our case, it is not necessary to solve the heat transfer equation inside the substrate, because we assume that the substrate connects with a thermostat, fixing its temperature $T_0$.

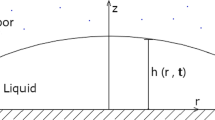
Note that the droplet surface of the spherical segment shape can be parameterized by the following equations:

$$\begin{aligned}&h(r,t)=\frac{R(\cos \varphi (r,t)-\cos \theta (t))}{\sin \theta (t)}; \nonumber \\&\varphi (r,t)=\arcsin \left( \frac{r\sin \theta (t)}{R}\right) , \end{aligned}$$

(B6)

where $\varphi$ is the angle between the normal vector and the vertical axis z.

Appendix C: Velocity distribution inside the droplet for the Marangoni flux case

In the axial symmetry case, the Navier–Stokes equations can be rewritten in terms of vorticity, $\gamma (r,z)={\partial u_r}/{\partial z}-{\partial u_z}/{\partial r}$, and stream function, $\psi$, as follows: [42]:

$$\begin{aligned}&\frac{\partial }{\partial t}\gamma (r,z)+(\mathbf {v}\cdot \pmb {\nabla })\gamma (r,z) \!=\! \nu \left( \Delta \gamma (r,z)-\frac{\gamma (r,z)}{r^2}\right) ,\qquad \end{aligned}$$

(C1)

$$\begin{aligned}&\frac{\partial ^2\psi }{\partial r^2}-\frac{1}{r}\frac{\partial \psi }{\partial r}+ \frac{\partial ^2\psi }{\partial z^2} = r\gamma , \end{aligned}$$

(C2)

where the stream function is related to the velocity components via the following relations ${\partial \psi }/{\partial z}=ru_r$, ${\partial \psi }/{\partial r}=-ru_z$.

The boundary conditions: $\gamma =0$ at $r=0$, $\gamma =\partial u_r/\partial z$ at $z=0$; $\gamma =\eta ^{-1}{d\sigma }/ds+2u_\tau d\varphi /ds$ at the droplet boundary; $\psi =0$ at all the boundaries: droplet boundary, axis of symmetry ($r=0$) and substrate-fluid interface ($z=0$). Here, ${d\sigma }/{ds}=\sigma ^{\prime }\partial T/\partial s$ is the derivative of the surface tension with respect to the arc length along the droplet surface, where the distribution of temperature discussed above is taken into account; $\sigma ^{\prime }=\partial \sigma /\partial T$ is derivative of surface tension with respect to the temperature. Variable $\varphi$ is the angle between the normal vector and the vertical axis z, so that $\partial T/\partial s = \cos \varphi \cdot \partial T/\partial r - \sin \varphi \cdot \partial T/\partial z$, and the tangent component of velocity is $u_\tau = u_r \cos \varphi -u_z \sin \varphi$. For the spherical shape of the droplet, described by equations (B6), the arc length s is described by $\varphi$ via the relation $s = R\varphi / \sin \theta$. In particular, at the contact line $s_{max} = R\theta / \sin \theta$.

Appendix D: Boundary conditions for Navier–Stokes equations for the capillary fluid flow case

The boundary conditions for Navier–Stokes equations discussed in Appendix C were derived with the assumption that $u_n = 0$. That is why the capillary flow was not taken into account in this derivation. In order to take into account the capillary fluid flow, it is necessary to rewrite the boundary conditions in a more general form. For the vorticity at the droplet boundary, we have

$$\begin{aligned} \gamma = \frac{1}{\eta }\frac{d\sigma }{ds} + 2u_\tau \frac{d\varphi }{ds}-2\frac{\partial u_n}{\partial s}, \end{aligned}$$

(D1)

where $u_n = u_r \sin \varphi + u_z \cos \varphi = -\partial \psi / (r\partial s)$. For the stream function $\psi =0$ at the axis of the droplet symmetry and the droplet-substrate interface. However, at the droplet boundary, we have

$$\begin{aligned} \psi = \int _0^s (-r u_n) ds. \end{aligned}$$

(D2)

As it follows from the mass conservation law in an infinitely small cylindrical volume near the droplet surface, on the droplet surface, the following relation

$$\begin{aligned} u_n - \frac{\partial h(r,t)}{\partial t}\cos \varphi = \frac{J_s(r,t)}{n} \end{aligned}$$

(D3)

is fulfilled, where n is the liquid density.

Note that $m = n\pi h_0^2 (R-h_0/3)$, where $h_0 = R(1-\cos \theta )/\sin \theta$, i.e., $m = \frac{1}{3}\pi R^3n (3-\tan (\theta /2))\tan ^2(\theta /2)$. Therefore,

$$\begin{aligned} \frac{dm}{dt} = -\frac{1}{2}n\pi R^3 \frac{\tan \frac{\theta }{2}-2}{\cos ^2\frac{\theta }{2}}\tan \frac{\theta }{2}\cdot \frac{d\theta }{dt}. \end{aligned}$$

(D4)

Thus, using the relation

$$\begin{aligned} \frac{dm}{dt}=-\int _0^{s_{max}} 2\pi r(s) J(r(s)) ds, \end{aligned}$$

(D5)

one can calculate $d\theta /dt$. Further, using equations (B6), we get

$$\begin{aligned} \frac{\partial h(r,t)}{\partial t} = \frac{R }{\sin ^2\theta }\left( 1-\frac{\cos \theta }{\cos \varphi }\right) \frac{d\theta }{dt}. \end{aligned}$$

(D6)

Knowing $\partial h(r,t)/\partial t$, we obtain $u_n$ on the droplet boundary using Eq. (D3). Knowing $u_n$, we can use boundary conditions (D1) and (D2).