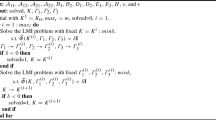Skip to main content
SpringerLink
Log in

Completeness on the Stability Criterion of Fractional Order LTI Systems

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

Abstract

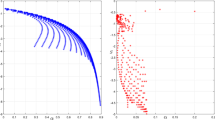
The importance of the concept of stability in fractional order system and control has been recognized for some time now. Recently, it has become evident that many conclusions were drawn, but little consensus was reached. Consequently, there is an urgent need for a much deeper understanding of such a concept. With the definition of fractional order positive definite matrix, a set of equivalent and elegant stability criteria are developed via revisiting a stability criterion we proposed before. All the results are formed in terms of linear matrix inequalities. Afterwards, a series of interesting properties of these criteria are revealed profoundly, including completeness, singularity, conservatism, etc. Eventually, a simulation study is provided to validate the effectiveness of the obtained results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. H.S. Ahn and Y.Q. Chen, Necessary and sufficient stability condition of fractional-order interval linear systems. Automatica 44, No 11 (2008), 2985–2988 10.1016/j.automatica.2008.07.003

    Article  MathSciNet  Google Scholar 

  2. R. Caponetto and S. Graziani and V. Tomasello and A. Pisano, Identification and fractional super-twisting robust control of IPMC actuators. Fract. Calc. Appl. Anal. 18, No 6 (2015), 1358–1378 10.1515/fca-2015-0079; https://www.degruyter.com/view/j fca.2015.18.issue-6/issue-files/fca.2015.18.issue-6.xml

    Article  MathSciNet  Google Scholar 

  3. L.P. Chen and Y.G. He and Y. Chai and R.C. Wu, New results on stability and stabilization of a class of nonlinear fractional-order systems. Nonlinear Dynam. 75, No 4 (2014), 633–641 10.1007/s11071-013-1091-5

    Article  MathSciNet  Google Scholar 

  4. M. Chilali and P. Gahinet and P. Apkarian, Robust pole placement in LMI regions. IEEE Trans. Autom. Control 44, No 12 (1999), 2257–2270 10.1109/9.811208

    Article  MathSciNet  Google Scholar 

  5. M.A. Duarte-Mermoud and N. Aguila-Camacho and J.A. Gallegos and and R. Castro-Linares, Using general quadratic Lyapunov functions to prove Lyapunov uniform stability for fractional order systems. Commun. Nonlinear. Sci. 22, No 1 (2015), 650–659 10.1016/j.cnsns.2014.10.008

    Article  MathSciNet  Google Scholar 

  6. M.O. Efe, Fractional order systems in industrial automation-a survey. IEEE Trans. Ind. Inform. 7, No 4 (2011), 582–591 10.1109/TII.2011.2166775

    Article  Google Scholar 

  7. C. Farges and M. Moze and J. Sabatier, Pseudo-state feedback stabilization of commensurate fractional order systems. Automatica 46, No 10 (2010), 1730–1734 10.1016/j.automatica.2010.06.038

    Article  MathSciNet  Google Scholar 

  8. T.J. Freeborn and B. Maundy and A.S. Elwakil, Fractional-order models of supercapacitors, batteries and fuel cells: A survey. Renew. Sust. Energ. Rev. 4, No 3 (2015), 1–7 10.1007/s40243-015-0052-y

    MATH  Google Scholar 

  9. C. Ionescu and C. Muresan, Sliding mode control for a class of subsystems with fractional order varying trajectory dynamics. Fract. Calc. Appl. Anal. 18, No 6 (2015), 1441–1451 10.1515/fca-2015-0083; }rs https://www.degruyter.com url }/viewZj/fca.2015.18.issue-6/issue-files/fca.2015.18.issue-6.xml

    Article  MathSciNet  Google Scholar 

  10. Y. Li and Y.Q. Chen and I. Podlubny, Mittag–Leffler stability of fractional order nonlinear dynamic systems. Automatica 45, No 8 (2009), 1965–1969 10.1016/j.automatica.2009.04.003

    Article  MathSciNet  Google Scholar 

  11. S. Liang and C. Peng and Y. Wang, Improved linear matrix inequalities stability criteria for fractional order systems and robust stabilization synthesis: The 0 < α < 1 case. Contl. Theor. Appl. 30, No 4 (2013), 531–535 10.7641/CTA.2013.20674

    Google Scholar 

  12. J.G. Lu and Y.Q. Chen, Robust stability and stabilization of fractional-order interval systems with the fractional order α: The 0 < α < 1 case. IEEE Trans. Autom. Control 55, No 1 (2010), 152–158 10.1109/TAC.2009.2033738

    Article  MathSciNet  Google Scholar 

  13. J.G. Lu and Y.Q. Chen, Stability and stabilization of fractional-order linear systems with convex polytopic uncertainties. Fract. Calc. Appl. Anal. 16, No 1 (2013), 142–157 10.2478/s13540-013-0010-2; }rs https://www.degruyter.com url }/view/j/fca.2013.16.issue-1/issue-files/fca.2013.16.issue-1.xml

    Article  MathSciNet  Google Scholar 

  14. J.A.T. Machado and V. Kiryakova and F. Mainardi, Recent history of fractional calculus. Commun. Nonlinear. Sci. 16, No 3 (2011), 1140–1153 10.1016/j.cnsns.2010.05.027

    Article  MathSciNet  Google Scholar 

  15. B.B. Mandelbrot, A class of long-tailed probability distributions and the empirical distribution of city sizes. In: F. Massarik and P. Ratoosh, Mathematical Explanations in Behavioral Science Homewood Editions New York, (1965), 322–332

    Google Scholar 

  16. D. Matignon, Stability results for fractional differential equations with applications to control processing, IMACS Multiconference: Computational Engineering in Systems Applications Lille France, 1996), 963–968

    Google Scholar 

  17. M. Moze and J. Sabatier and A. Oustaloup, LMI tools for stability analysis of fractional systems, 5th Internat. Conference on Multibody Systems, Nonlinear Dynamics, and Control Long Beach USA, 2005), 1611–1619 10.1115/DETC2005-85182

    Google Scholar 

  18. A. Oustaloup and B. Mathieu and P. Lanusse, The CRONE control of resonant plants: application to a flexible transmission. Eur. J. Control 1, No 2 (1995), 113–121 10.1016/S0947-3580(95)70014-0

    Article  Google Scholar 

  19. I. Podlubny, Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Eqnations, to Methods of Their Solution and Some of Their Applications Academic Press San Diego, 1999

    MATH  Google Scholar 

  20. I. Podlubny, Fractional-order systems and PIλDμ controllers. IEEE Trans. Autom. Control 44, No 1 (1999), 208–214 10.1109/9.739144

    Article  MathSciNet  Google Scholar 

  21. J. Sabatier and M. Moze and C. Farges, On stability of fractional order systems. Third IFAC Workshop on Fractional Differentiation and its Applications Ankara Turkey, 2008), hal-00322949

    Google Scholar 

  22. M.S. Tavazoei, Time response analysis of fractional-order control systems: A survey on recent results. Fract. Calc. Appl. Anal. 17, No 2 (2014), 440–461 10.2478/s13540-014-0179-z }rs https://www.degruyter.com url }/viewZj/fca.2014.17.issue-2/issue-files/fca.2014.17.issue-2.xml

    Article  MathSciNet  Google Scholar 

  23. M.S. Tavazoei and M. Haeri, A note on the stability of fractional order systems. Math. Comput. Simulat. 79, No 5 (2009), 1566–1576 10.1016/j.matcom.2008.07.003

    Article  MathSciNet  Google Scholar 

  24. J.C. Trigeassou and N. Maamri and J. Sabatier and A. Oustaloup, A Lyapunov approach to the stability of fractional differential equations. Signal Process 91, No 3 (2011), 437–445 10.1016/j.sigpro.2010.04.024

    Article  Google Scholar 

  25. S. Victor and P. Melchior, Improvements on flat output characterization for fractional systems. Fract. Calc. Appl. Anal. 18, No 1 (2015), 238–260 10.1515/fca-2015-0016 https://www.degruyter.com/view/j/fca.2015.18.issue-1/issue-files/fca.2015.18.issue-1.xml

    Article  MathSciNet  Google Scholar 

  26. Y.H. Wei and W.P. Tse and Z. Yao and Y. Wang, Adaptive backstepping output feedback control for a class of nonlinear fractional order systems. Nonlinear Dynam. 86, No 2 (2016), 1047–1056 10.1007/s11071-016-2945-4

    Article  MathSciNet  Google Scholar 

  27. Y.H. Wei and W.P. Tse and B. Du and Y. Wang, An innovative fixed-pole numerical approximation for fractional order systems. ISA Transactions 62 (2016), 94–102 10.1016/j.isatra.2016.01.010

    Article  Google Scholar 

  28. C. Yin and Y.Q. Chen and S.M. Zhong, Fractional-order sliding mode based extremum seeking control of a class of nonlinear systems. Automatica 50, No 12 (2014), 3173–3181 10.1016/j.automatica.2014.10.027

    Article  MathSciNet  Google Scholar 

  29. J.M. Yu and H. Hu and S.B. Zhou and X.R. Lin, Generalized Mittag-Leffler stability of multi-variables fractional order nonlinear systems. Automatica 49, No 6 (2013), 1798–1803 10.1016/j.automatica.2013.02.041

    Article  MathSciNet  Google Scholar 

  30. R.X. Zhang and G. Tian and S.P. Yang and H.F. Cao, Stability analysis of a class of fractional order nonlinear systems with order lying in (0, 2). ISA Transactions 56 (2015), 102–110 10.1016/j.isatra.2014.12.006

    Article  Google Scholar 

  31. X.F. Zhang and Y.Q. Chen, D-stability based LMI criteria of stability and stabilization for fractional order systems. Internat. Design Engineering Technical Conferences & Computers and Information in Engineering Conference Boston USA, 2015),DETC2015-46692 10.1115/DETC2015-46692

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Vibration Control and Vehicle Control (VCVC) Lab Dept. of Automation, University of Science and Technology of China, No. 443 Huang Shan Rd., Shu Shan District, Hefei, 230027, P. R. China

    Yiheng Wei, Yuquan Chen, Songsong Cheng & Yong Wang

Authors
  1. Yiheng Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yuquan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Songsong Cheng
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Yong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yiheng Wei.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wei, Y., Chen, Y., Cheng, S. et al. Completeness on the Stability Criterion of Fractional Order LTI Systems. FCAA 20, 159–172 (2017). https://doi.org/10.1515/fca-2017-0008

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1515/fca-2017-0008

MSC 2010

Key Words and Phrases

Search

Navigation